Your next great Lessn starts here.

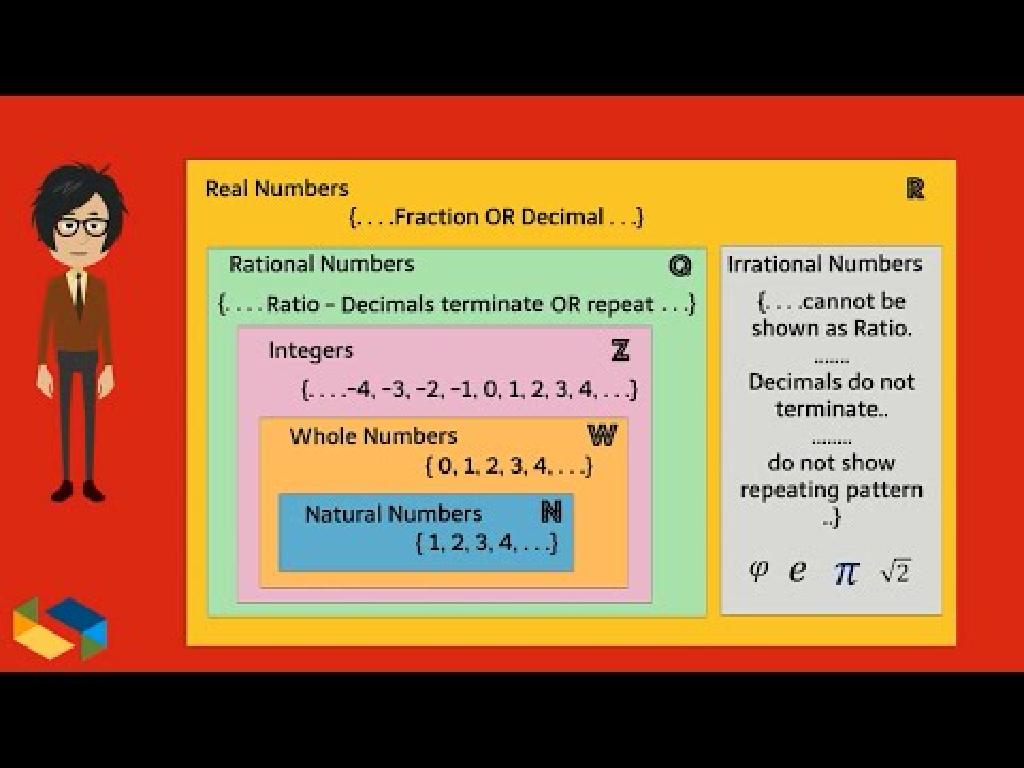
Understanding Rational Numbers – Define rational numbers – Numbers that can be expressed as a fraction a/b, where a and b are integers and b is not zero. – Characteristics of rational numbers – They include integers, fractions, and repeating or terminating decimals. – Examples of rational numbers – 1/2, -3/4, 0.75, -2, 5 – Absolute value concept – Absolute value is the distance from zero on a number line, denoted by | |, e.g., | 3| = 3 | This slide introduces the concept of rational numbers, which are any numbers that can be expressed as a fraction of two integers, with the denominator not being zero. This includes positive and negative integers, fractions, and both repeating and terminating decimals. Emphasize that the absolute value of a rational number is its distance from zero on the number line, regardless of direction, which is why | 3| equals 3. Provide various examples to illustrate the concept and ensure students understand that the absolute value represents a non-negative value of the number.

Understanding Absolute Value – Define Absolute Value – Absolute Value is the distance of a number from zero, regardless of direction. – Distance from zero on a number line – Think of it as how far a number is from zero: |-3| = 3, because it’s 3 units away. – Always positive or zero – It never represents a negative value: |7| = 7, |-7| = 7. – Notation and examples – Use ‘|’ symbols to denote Absolute Value: |5|, |-8|, |0| = 0. | Introduce the concept of Absolute Value to students as a measure of distance on the number line, emphasizing that it is always non-negative. Explain that Absolute Value is not concerned with the direction (left or right from zero), but purely with the magnitude of distance. Provide clear examples using both positive and negative numbers, as well as zero, to illustrate that the Absolute Value of any number is either positive or zero. Use the vertical bar notation to familiarize students with how Absolute Value is represented in mathematical expressions. Encourage students to think of real-life scenarios where only the magnitude of a value matters, not the direction, such as the distance traveled.

Absolute Value of Rational Numbers – Find absolute value of fractions – | -3/4 | becomes 3/4 after removing the sign – Absolute value of decimals – | 2.5 | remains 2.5 as it’s already positive – Examples: | -3/4 |, | 2.5 |, | -1.2 | – | -1.2 | turns into 1.2, sign is disregarded – Distance from zero is key – Think of it as the number’s ‘step count’ from zero | This slide introduces the concept of absolute value in the context of rational numbers, both fractions and decimals. The absolute value of a number is its distance from zero on the number line, regardless of direction. It’s important to emphasize that the absolute value is always non-negative. Use the examples to show how to strip the negative sign from negative numbers to find their absolute value. Encourage students to visualize the number line and to think of absolute value as how many steps it takes to get back to zero. This concept is foundational for understanding rational numbers and will be applied in future lessons involving complex calculations.

Comparing Absolute Values of Rational Numbers – Compare rational numbers with Absolute Value – Absolute Value measures distance from zero, regardless of direction – Example: | -7/3 | vs | 2/3 | – | -7/3 | = 7/3 and | 2/3 | = 2/3, so 7/3 > 2/3 – Ordering numbers using Absolute Value – List numbers from least to greatest Absolute Value | This slide introduces students to the concept of comparing and ordering rational numbers by their Absolute Values. Start by explaining that Absolute Value represents the distance a number is from zero on a number line, which is always positive. Use the example to show how to compare the Absolute Values of two rational numbers. Demonstrate that | -7/3 |, which is 7/3, is greater than | 2/3 |, which remains 2/3. Then, guide students on how to use Absolute Values to arrange a set of rational numbers in order. This concept is crucial for understanding the magnitude of numbers in different contexts.

Real-life Applications of Absolute Value – Absolute value in daily life – Temperature: Understanding changes – How much did the temperature rise or fall from zero? – Finance: Gains and losses – Tracking money earned or spent without regard to plus/minus – Neutral distance measurement – How far something is, regardless of direction | This slide aims to show students how the concept of absolute value is relevant and commonly used in everyday situations. Absolute value helps us describe and quantify real-world scenarios in a neutral and non-directional way. For instance, when discussing temperature, we can use absolute value to express the magnitude of change from a starting point, such as the difference in degrees from zero. In finance, it allows us to consider the amount of money gained or lost, without the initial positive or negative connotation. And when it comes to distance, absolute value helps us understand how far apart two points are, without considering the direction. Encourage students to think of other examples where absolute value might be applicable in their daily lives.

Class Activity: Exploring Absolute Values – Understand Absolute Values – Worksheet practice on rational numbers – Calculate the Absolute Value of given rational numbers – Pair up for answer comparison – Check your answers with a classmate – Discuss findings with the class – Share what you’ve learned about Absolute Values | This activity is designed to reinforce the concept of absolute value in the context of rational numbers. Provide students with a worksheet containing a variety of rational numbers and ask them to calculate the absolute value of each. Encourage them to work independently first, and then pair up with a partner to compare their answers. This peer interaction will help them to understand different approaches and correct any misconceptions. After the pair work, lead a class discussion where students can share their findings and reflect on the importance of absolute value in mathematics. Possible activities for different pairs could include finding absolute values of positive and negative fractions, mixed numbers, and decimals to cater to varying levels of difficulty.

Wrapping Up: Absolute Value – Recap: What is Absolute Value? The distance of a number from zero on a number line, regardless of direction. – Importance of Absolute Value Helps in understanding magnitude and is used in real-world situations like distances. – Review of today’s examples We looked at examples like |-3| = 3 and |4/5| = 4/5. – Open floor for questions | As we conclude today’s lesson, let’s revisit the concept of absolute value, which is the distance of a number from zero on the number line without considering direction. Emphasize its importance in various real-life contexts, such as finding the actual distance between two points. Review the examples discussed in class to reinforce learning, such as the absolute value of negative numbers and fractions. Finally, encourage students to ask questions or express any confusion they might have. Addressing these will help ensure that they have a solid understanding of the topic as they move forward.

Homework: Mastering Absolute Values – Practice absolute value problems – Solve assigned problems to understand absolute values of rational numbers – Study for the upcoming quiz – Review notes and complete practice exercises to prepare for the quiz – Compile questions for next class – Write down any uncertainties or confusions about the topic – Review rational numbers concepts – Revisit the definition and properties of rational numbers | This homework assignment is designed to reinforce students’ understanding of the absolute value of rational numbers. Encourage them to complete the practice problems to gain confidence in identifying and working with absolute values. Remind them that the quiz will cover all aspects of rational numbers, so thorough preparation is crucial. Students should come to the next class with questions, demonstrating their engagement with the material. This will also provide an opportunity for clarification before the quiz. Additionally, reviewing the fundamental concepts of rational numbers will help solidify their grasp of the topic.