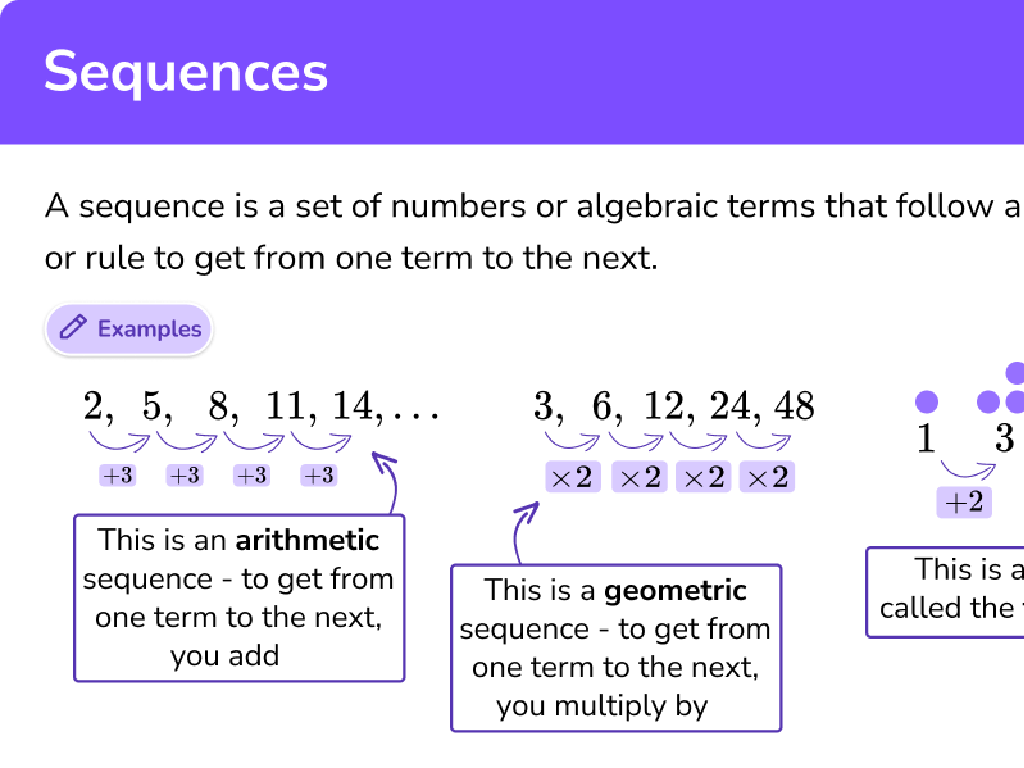
Estimate Sums And Differences Of Fractions Using Benchmarks
Subject: Math
Grade: Fifth grade
Topic: Add And Subtract Fractions
Please LOG IN to download the presentation. Access is available to registered users only.
View More Content
Estimating Sums and Differences of Fractions
– Recap: What are fractions?
– Fractions represent parts of a whole, like 1/2 of a pizza.
– Understanding estimation
– Estimation helps us find a number close to the exact answer, quickly.
– The usefulness of estimating fractions
– Estimating makes math faster and easier in real-life situations.
– Estimation techniques in practice
– Use benchmarks like 0, 1/2, and 1 to estimate sums and differences.
|
Begin with a brief review of fractions to ensure students recall that fractions represent parts of a whole. Then, introduce the concept of estimation as a method to find an approximate answer without calculating the exact number, which can save time and effort. Discuss why it’s useful to estimate fractions, such as when measuring ingredients in cooking or determining time. Finally, demonstrate how to use benchmarks like 0, 1/2, and 1 to estimate the results of adding or subtracting fractions, which is a practical skill for everyday problem-solving. Encourage students to think of situations where they could apply these techniques.
Estimating with Fraction Benchmarks
– Understand common benchmarks
– Benchmarks like 0, 1/2, and 1 guide our estimates
– Using benchmarks to estimate
– Compare fractions to 0, 1/2, or 1 to estimate their size
– Practice estimation examples
– If a fraction is just over 1/2, estimate up to 1 for sums
– Why benchmarks are helpful
– Benchmarks simplify complex fraction problems
|
This slide introduces students to the concept of using benchmarks to estimate the sums and differences of fractions. Benchmarks are standard numbers we use to make quick estimates of where fractions lie on the number line. By comparing fractions to 0, 1/2, and 1, students can quickly decide if a fraction is closer to one of these benchmarks and make an estimation. For example, if a fraction is slightly more than 1/2, students can round up to 1 for a sum estimation. This method is particularly useful when precise calculations are not necessary and can greatly simplify problem-solving. Encourage students to practice with real-life examples, such as estimating the amount of pizza left or the distance run in a race, to reinforce the concept.
Estimating Sums of Fractions
– Learn steps to estimate sums
– Round fractions to 0, 1/2, or 1 to simplify
– Example: Estimate 3/8 + 4/5
– 3/8 (~1/2) + 4/5 (~1) = ~1 1/2 or 1.5
– Group practice estimation
– Work in groups to estimate sums of fractions
– Discuss estimation benefits
|
This slide introduces the concept of estimating the sum of fractions, a key skill in fifth-grade math. Start by explaining the steps to estimate sums, which include rounding fractions to the nearest benchmark (0, 1/2, or 1) for easier mental calculation. Use the example 3/8 + 4/5 to show how to round each fraction and then add them together. Organize a group practice session where students work together to estimate sums of different fractions, fostering collaboration and reinforcing the concept. Emphasize the importance of estimation in making quick and reasonable predictions in real-life situations, such as when measuring ingredients for a recipe or determining time left for an activity.
Estimating Differences of Fractions
– Steps to estimate fraction differences
– Round fractions to 0, 1/2, or 1 to simplify
– Example: Estimate 5/6 – 1/3
– 5/6 is close to 1, and 1/3 is about 1/2. So, 1 – 1/2 = 1/2
– Group practice activity
– Work in groups to estimate differences using benchmarks
|
This slide introduces the concept of estimating the differences between fractions by using benchmarks such as 0, 1/2, or 1. Start by explaining the steps to round fractions to these benchmarks to simplify the process. Use the example of 5/6 – 1/3 to illustrate how to estimate: since 5/6 is close to 1 and 1/3 is about 1/2, the estimated difference is 1/2. Encourage students to practice this skill in groups with various fraction pairs. Provide guidance on how to round fractions and ensure they understand that estimation is not about finding the exact answer but rather a close approximation that is easier to calculate.
Estimating Sums and Differences of Fractions
– Visualize with number lines
– Number lines help us see where fractions fit between whole numbers
– Round to nearest benchmark
– Round fractions to 0, 1/2, or 1 to simplify calculations
– Check your estimates
– Compare estimates to actual sums/differences to verify accuracy
– Practice estimation skills
|
This slide introduces students to the concept of estimating sums and differences of fractions using benchmarks. Encourage students to use number lines as a visual aid to understand the position of fractions relative to whole numbers. Teach them to round fractions to the nearest benchmark (0, 1/2, or 1) to make mental calculations easier. Emphasize the importance of checking their estimates against the actual sums or differences to ensure accuracy. Provide practice problems where students can apply these tips and tricks to strengthen their estimation skills. This will help them become more efficient in solving problems involving fractions.
Class Activity: Fraction Estimation Game
– Split into teams for estimation
– Estimate sums and differences
– Guess the result of fraction addition or subtraction
– Use benchmarks for quick decisions
– 0, 1/2, and 1 are good benchmarks for quick estimates
– Share and discuss estimates
– Explain your reasoning to the class
|
This interactive class activity is designed to help students practice estimating the sums and differences of fractions using benchmarks. Divide the class into small teams and provide each team with a set of fraction problems. Encourage them to use benchmarks such as 0, 1/2, and 1 to make quick and reasonable estimates without calculating the exact sums or differences. After the activity, have each team share their estimates with the class and discuss the different approaches taken. This will foster a collaborative learning environment and enhance their understanding of fractions. Possible variations of the activity could include using visual aids, incorporating a timed challenge, or creating a friendly competition between teams.
Wrapping Up: Estimating Fractions
– Review of fraction estimation
– Practice makes perfect
– Homework: Estimation worksheet
– Complete the provided worksheet to practice estimating sums and differences of fractions.
– Bring questions next class
– Note any difficulties to discuss.
|
As we conclude today’s lesson on estimating sums and differences of fractions, remind students of the key points and strategies discussed. Emphasize the importance of practice in mastering this skill, as it helps solidify their understanding and improves accuracy. For homework, assign the provided worksheet which contains various problems requiring students to estimate the sums and differences of fractions using benchmarks. Encourage them to attempt all problems and bring up any questions or challenges they encounter to the next class for clarification. This will not only help them but also provide feedback on areas that may need further review.