Your next great Lessn starts here.

Introduction to Fractions – Recap: Definition of a fraction – A fraction represents a part of a whole – Numerators vs. Denominators – Top number (numerator) and bottom number (denominator) – Visualize fractions with examples – Pizza slices, pie pieces, and divided apples – Practice with real-life fractions | Begin the lesson by recapping the concept of fractions, ensuring students remember that a fraction represents a part of a whole. Clarify the roles of numerators and denominators, with the numerator indicating how many parts are being considered and the denominator showing the total number of equal parts in the whole. Use visual aids like pictures of pizza slices, pie pieces, and divided apples to help students visualize fractions in a tangible way. Engage the class with examples that relate to their everyday experiences, such as sharing snacks or dividing items among friends, to solidify their understanding. Encourage students to think of other everyday examples and share them with the class. This will set a strong foundation for learning how to complete addition and subtraction sentences with fractions.

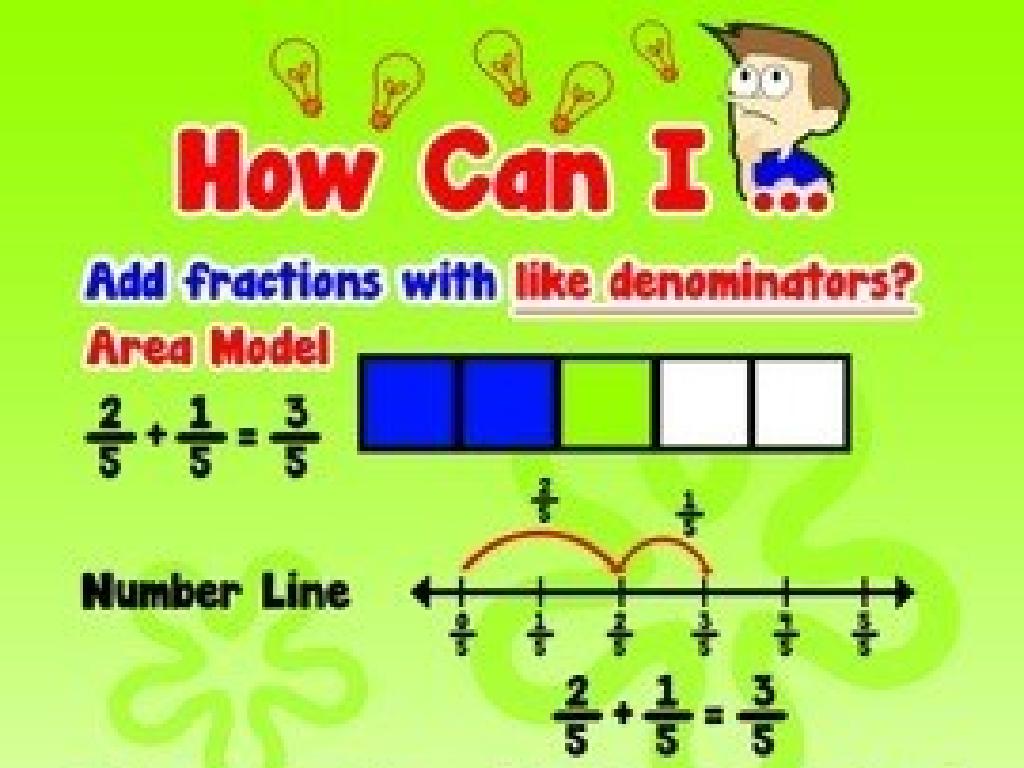
Adding Fractions with Same Denominators – Add fractions with like denominators – Simply add the numerators and keep the same denominator – Visual aids for fraction addition – Use pie charts or bar models to show parts being combined – Example: 1/4 + 2/4 equals 3/4 – By adding the tops (numerators), we get 3/4 as the sum | When adding fractions with the same denominator, students should be taught to add only the numerators (the top numbers) while keeping the denominator (the bottom number) the same. Visual aids like pie charts or bar models can be extremely helpful in showing how parts of the same whole are combined. For example, if we have one quarter of a pie and add two more quarters, we now have three quarters of the pie. This concept is fundamental in understanding fraction addition before moving on to fractions with different denominators. Ensure students practice with various examples and use visual aids to reinforce the concept.

Subtracting Fractions with Same Denominator – Subtract numerators directly – Just like 3 apples – 1 apple = 2 apples, for 3/4 – 1/4, subtract 3 – 1 – Keep the denominator same – Visual aids for subtraction – Use pie charts or bar models to show parts being taken away – Example: 3/4 – 1/4 – 3/4 pie minus 1/4 pie leaves us with 1/2 pie | When subtracting fractions with the same denominator, students should understand that only the numerators change while the denominator remains constant. This is because the denominator represents the total number of equal parts the whole is divided into, which doesn’t change when subtracting parts. Visual aids like pie charts or bar models can be very helpful in showing this concept, as they allow students to see the parts being removed. For example, if you have a pie cut into 4 equal parts and you take away 1 part, you are left with 3 parts. This is represented by the fraction 3/4 – 1/4 = 2/4, which simplifies to 1/2. Encourage students to practice with different examples and use visual aids to reinforce their understanding.

Completing Addition Sentences with Fractions – Understand addition sentences – An addition sentence with fractions combines parts to make a whole. – Learn to find missing numerators – The numerator is the top number of a fraction, representing parts of a whole. – Activity: Fill in the blank – Use fraction knowledge to find what adds with 1/3 to make 1. | This slide introduces students to the concept of completing addition sentences with fractions. Start by explaining that an addition sentence with fractions is like putting pieces together to make a whole. Emphasize the role of the numerator and how it can change while the denominator remains constant. For the activity, guide students to understand that 1/3 needs 2/3 more to make a whole (1), since 1/3 + 2/3 = 3/3, which equals 1. Encourage students to use visual aids like fraction strips or pie charts to visualize the concept. Provide additional examples if time allows and ensure that students are comfortable with the idea before moving on to more complex problems.

Complete the Sentence: Subtraction with Fractions – Understanding subtraction sentences – Subtraction sentences with fractions have a missing part we need to find. – How to find the missing numerator – To find a missing numerator, subtract the fraction from the whole. – Activity: Solve 5/6 – __ = 1/2 – Use fraction subtraction to find what number completes the sentence. | This slide introduces students to the concept of completing subtraction sentences involving fractions. Start by explaining that a subtraction sentence with fractions is like a puzzle where we need to find the missing piece. Teach them that to find a missing numerator, they should think of subtracting the known fraction from the whole fraction given. For the activity, guide the students to solve the subtraction sentence 5/6 – __ = 1/2 by finding the missing fraction that makes the sentence true. Encourage them to use fraction bars or number lines if they need a visual aid to understand the concept better. The activity will help solidify their understanding of fraction subtraction and how to find missing numerators.

Adding and Subtracting Fractions with Unlike Denominators – Find a common denominator – A shared multiple of the denominators, e.g., for 1/3 and 1/6, it’s 6 – Add or subtract the numerators – Keep the common denominator, add/subtract the top numbers – Example: 1/3 + 1/6 – Convert 1/3 to 2/6 and then add to 1/6 to get 3/6 – Simplify the fraction if needed – Reduce 3/6 to its simplest form, which is 1/2 | This slide introduces the concept of adding and subtracting fractions with unlike denominators. Start by explaining the necessity of finding a common denominator, which is a number that both denominators can divide into evenly. Use visual aids or fraction strips to help students understand this concept. Once a common denominator is found, guide students to convert each fraction to an equivalent fraction with the common denominator. Then, they can easily add or subtract the numerators while keeping the denominator the same. After combining the fractions, students should simplify the result to its lowest terms. Encourage students to practice with different sets of fractions and to check their work by simplifying their answers.

Class Activity: Fraction Puzzles – Pair up for fraction puzzles – Use fraction strips for visual aid – Fraction strips represent different fractions visually – Create addition & subtraction sentences – Combine strips to form addition sentences, take away for subtraction – Solve your own fraction sentences – Test each other’s sentences and find the solutions | This activity is designed to help students understand fractions through a hands-on experience. By working in pairs, students can collaborate and discuss strategies for solving fraction puzzles. Provide each pair with a set of fraction strips, which are a great visual tool for comparing different fractions and understanding their sizes relative to one another. Encourage students to manipulate the strips to create their own addition and subtraction sentences, fostering a deeper comprehension of how fractions can be combined and separated. As they solve their own and each other’s sentences, they will practice their computational skills and enhance their understanding of fraction operations. Possible activities include combining strips to match a given fraction sum, finding the difference between two strips, or even challenging another pair to solve a puzzle they’ve created.

Review and Homework: Mastering Fractions – Review today’s fraction concepts – Discuss questions or difficulties – Homework: Fractions worksheet – Add and subtract fractions as instructed – Practice makes perfect! – Remember: Regular practice improves skills | This slide is aimed at reinforcing the day’s lessons on adding and subtracting fractions. Start by reviewing the key concepts, ensuring that students are clear on how to find common denominators and combine fractions. Open the floor for any questions students may have, addressing difficulties they encountered during the lesson. For homework, assign a worksheet that provides additional practice on adding and subtracting fractions, which will help solidify their understanding. Emphasize the importance of practice in mastering math skills and encourage students to attempt all problems and seek help if needed.