Your next great Lessn starts here.

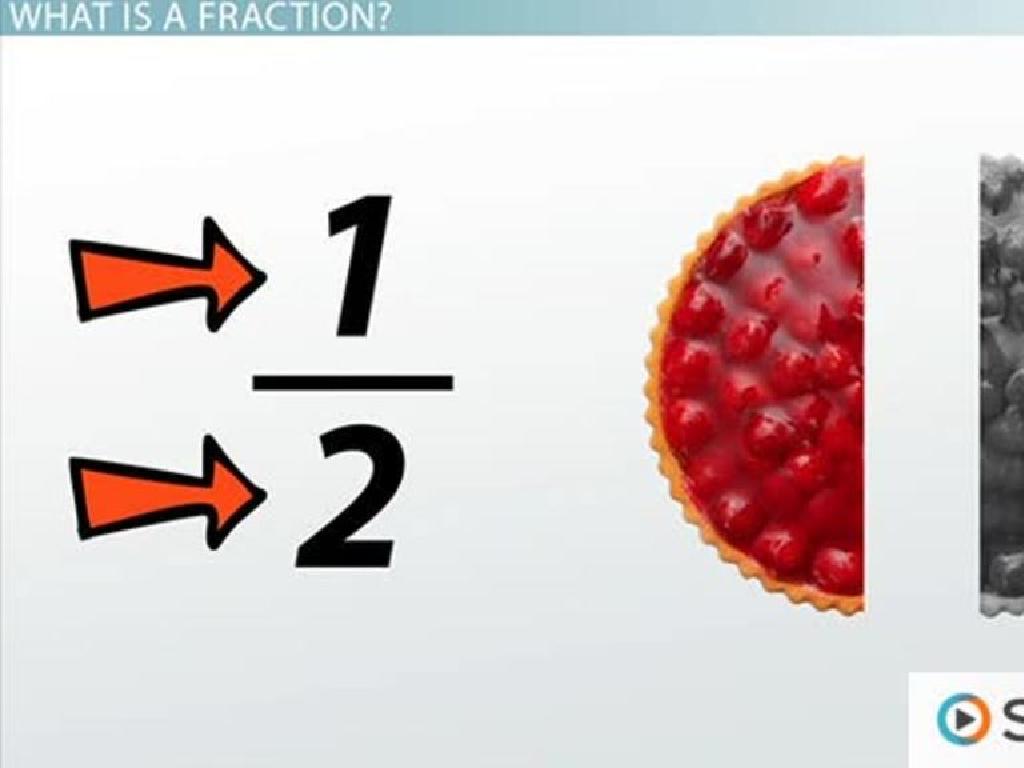
Introduction to Fractions – What exactly is a fraction? – A fraction represents a part of a whole. – Numerator and Denominator explained – Top number (numerator) and bottom number (denominator). – Fractions in daily life – Pizza slices, sharing candy, measuring cups. – Comparing fractions – Which is larger: 1/2 pizza or 1/4 pizza? | This slide introduces the concept of fractions to fourth-grade students. Begin by explaining that a fraction represents a part of a whole, something they encounter in various forms every day. Clarify the terms numerator and denominator, with the numerator indicating how many parts we have and the denominator showing the total number of equal parts in the whole. Use relatable examples like slices of pizza or sharing candy to illustrate fractions in a context familiar to them. Encourage students to think of their own examples. Conclude by introducing the idea of comparing fractions with a simple example, setting the stage for deeper exploration into finding smaller or larger fractions.

Comparing Fractions – Understanding fraction comparison – Comparing fractions means deciding which is larger or smaller. – Symbols: >, for greater, < for less, and = for equal. – Same denominator comparison – If denominators are the same, just compare numerators. – Practice with examples – Let's compare 1/4 to 3/4 and see which is larger. | This slide introduces the concept of comparing fractions, which is a fundamental skill in understanding mathematical relationships between different quantities. Start by explaining what it means to compare fractions and ensure that students are comfortable with the greater than, less than, and equal signs. Emphasize that when fractions have the same denominator, comparison is straightforward simply look at the numerators. Provide several examples for the students to practice, such as comparing 1/4 to 3/4, and explain that since the denominators are the same (4), we only need to look at the numerators to determine that 3/4 is larger than 1/4. Encourage students to work through examples in pairs or groups for better understanding.

Finding Smaller or Larger Fractions – Steps to find smaller fractions – Compare numerators when denominators are the same – Steps to find larger fractions – Compare denominators when numerators are the same – Using 1/2 as a benchmark – If a fraction is more than 1/2, it’s larger; less than 1/2, it’s smaller – Practice comparing fractions – Let’s find which is larger: 3/8 or 5/8? | This slide introduces the concept of comparing fractions by finding which are smaller or larger. Start by explaining that when fractions have the same denominator, the one with the smaller numerator is the smaller fraction. Conversely, when they have the same numerator, the fraction with the larger denominator is smaller. Introduce the concept of using benchmarks, such as 1/2, to quickly estimate the size of a fraction. For example, if a fraction is greater than 1/2, it is closer to 1 and therefore larger than fractions less than 1/2. Encourage students to practice by comparing fractions with common numerators or denominators, and using 1/2 as a reference point. Provide several examples and encourage students to explain their reasoning.

Understanding Equivalent Fractions – What are equivalent fractions? – Fractions that have the same value, even if they look different – How to create equivalent fractions – Multiply the numerator and denominator by the same number – Using equivalents to compare – Compare fractions by finding common denominators – Practice with examples | This slide introduces the concept of equivalent fractions, which are different fractions that represent the same part of a whole. Teach students how to create equivalent fractions by multiplying the numerator and denominator by the same number. This is a crucial skill for comparing fractions, as it allows students to find a common denominator and determine which fraction is larger or smaller. Provide examples for the class to work through together, such as comparing 1/2 and 2/4 by showing they are equivalent. Encourage students to practice creating and using equivalent fractions with various examples.

Using Number Lines to Compare Fractions – Place fractions on a number line – Fractions represent points on a number line, between 0 and 1 – Compare fractions using the line – Look at their positions; left is smaller, right is larger – Identify smaller and larger fractions – The further from 0, the larger the fraction – Practice with examples | This slide introduces the concept of using number lines as a visual aid to compare fractions. Start by explaining how to place fractions on a number line, ensuring that students understand that each fraction corresponds to a specific point. Emphasize that fractions closer to 0 are smaller and those further away are larger. Use clear examples to show how to compare fractions by looking at their placement on the number line. For instance, 1/4 is smaller than 3/4 because it is closer to 0. Encourage students to practice by placing various fractions on a number line and determining which are smaller or larger. This will help solidify their understanding of the concept.

Class Activity: Fraction Comparison Game – Let’s play a fun fraction game! – Work in pairs to compare fractions – Partner up and take turns picking fractions – Find the smaller or larger fraction – Use fraction strips or number lines to decide – See who can do it faster! – Make it a friendly competition with classmates | This interactive game is designed to help students understand the concept of comparing fractions in a fun and engaging way. Have the students work in pairs. Each pair will take turns to draw fraction cards and decide which is smaller or larger. They can use fraction strips or number lines to help them visualize and compare the sizes. Encourage them to explain their reasoning to each other to reinforce their understanding. To add excitement, turn it into a timed challenge to see which pair can correctly compare the most fractions in a set time. Possible variations of the activity could include using different sets of fractions, incorporating mixed numbers, or having a tournament-style competition where pairs face off against each other.

Wrapping Up: Comparing Fractions – Review of fraction comparison – Why comparing matters – Helps in making fair decisions and understanding parts of a whole. – Homework: Practice worksheet – Complete the worksheet to practice finding smaller or larger fractions. – Bring questions next class – Ready to discuss any challenges faced. | As we conclude today’s lesson on comparing fractions, it’s crucial to emphasize the real-world applications of this skill, such as dividing a pizza among friends or measuring ingredients in a recipe. The homework assignment is a worksheet that will provide students with additional practice to reinforce their understanding of how to find smaller or larger fractions. Encourage students to attempt all problems and remind them that these exercises are designed to build their confidence in comparing fractions. In the next class, we will review the homework, address any questions, and ensure that everyone is comfortable with the concept.