Your next great Lessn starts here.

Welcome to Direct Variation – Define Direct Variation – A relationship where as one amount increases, another amount increases at a constant rate. – Direct Variation in real life – Examples: speed and distance, hours and pay. – Today’s Goal: Write equations – Solve direct variation problems – Use the formula y = kx, where k is the constant of variation. | Today’s lesson introduces the concept of direct variation, a fundamental principle in algebra where two variables change at a constant rate. This concept is widely applicable in real-world scenarios such as calculating speed (distance over time) or understanding wages (pay over hours). The goal for today is for students to learn how to write direct variation equations using the formula y = kx, where ‘k’ represents the constant of variation. Students will practice identifying this constant in various scenarios and solve problems to reinforce their understanding. Encourage students to think of other real-life examples where direct variation is observed and discuss how this concept is relevant to everyday life.

Understanding Direct Variation – Direct variation defined – When two variables increase/decrease together – Formula: y = kx – ‘y’ varies directly with ‘x’ with constant ‘k’ – Finding the constant ‘k’ – ‘k’ is the ratio of y to x (k = y/x) – Solving direct variation problems – Use the formula to solve for unknowns | Direct variation is a fundamental concept in algebra where two variables change at the same rate. The formula y = kx represents this relationship, where ‘k’ is the constant of variation. It’s crucial for students to understand that ‘k’ remains the same as ‘x’ and ‘y’ change proportionally. To find ‘k’, students will divide ‘y’ by ‘x’ using given values. Once ‘k’ is known, they can solve various direct variation problems by plugging in values for ‘x’ or ‘y’ to find the other variable. Provide examples with real-life contexts, such as speed and time, to illustrate direct variation. Encourage students to practice with different values to strengthen their understanding.

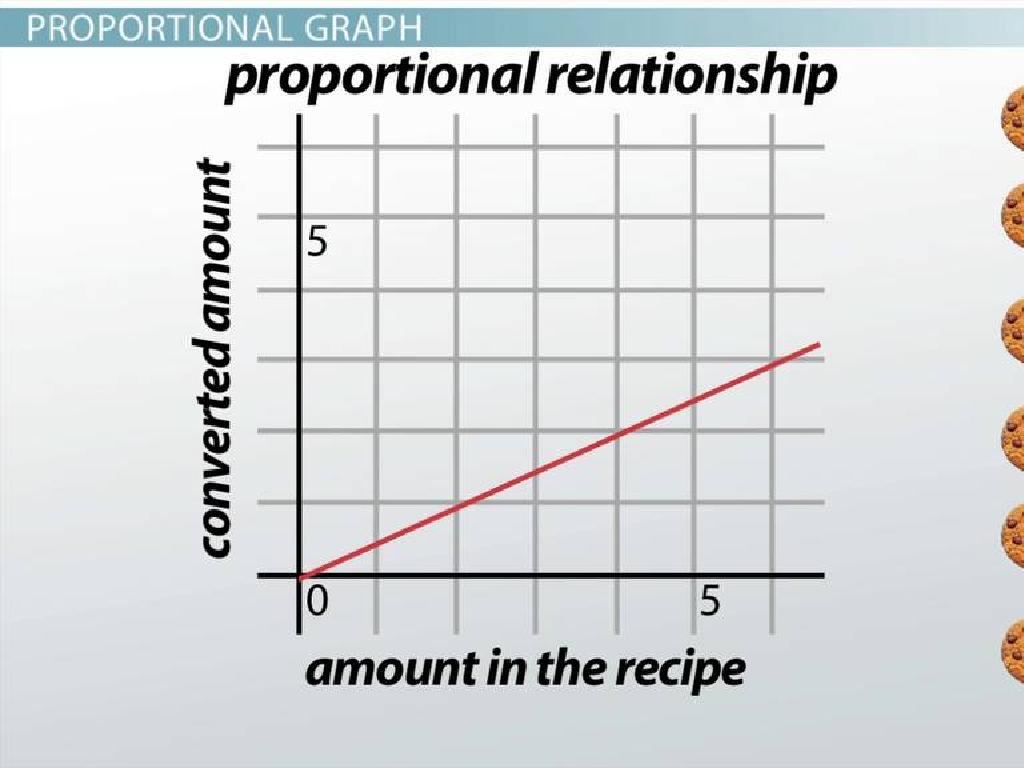
Identifying Direct Variation – Criteria for direct variation – Two quantities vary directly if they increase/decrease proportionally. – Plotting points on a graph – Points that lie on a straight line through the origin (0,0) indicate direct variation. – Recognizing direct variation – Example: Distance traveled over time at a constant speed. – Direct variation in tables – Tables showing direct variation have ratios of y to x that are constant. | When teaching direct variation, start by explaining that it occurs when two variables change at the same rate. A graph of these variables will show a straight line passing through the origin. Provide students with examples of tables and graphs that illustrate direct variation, such as the relationship between distance and time when moving at a constant speed. Encourage students to look for a constant ratio of y to x in tables as a sign of direct variation. During the lesson, have students practice plotting points and identifying whether they show direct variation.

Calculating the Constant of Variation – Use y = kx to find ‘k’ – Example: y = 12 when x = 3 – To solve for ‘k’, divide y by x: k = y/x = 12/3 = 4 – Practice: Find ‘k’ for y = 24, x = 6 – Apply the formula: k = y/x = 24/6 = 4 – Understanding ‘k’ in direct variation – ‘k’ represents how y changes with x | This slide introduces the concept of the constant of variation ‘k’ in direct variation equations. Start by explaining the formula y = kx, where ‘k’ is the constant of variation. It’s crucial for students to understand that ‘k’ remains the same as x and y change proportionally. Use the example to show how to calculate ‘k’ by dividing y by x. Then, provide a practice problem for students to solve on their own, reinforcing the concept. Emphasize that ‘k’ is a fixed number that describes the rate at which y changes in relation to x. Encourage students to solve for ‘k’ in various direct variation scenarios to gain a solid understanding of the relationship between variables in direct variation.

Writing Direct Variation Equations – Steps to write direct variation equations – Identify variables, establish a relationship – Determine the constant ‘k’ with known values – Use the formula k = y/x where y and x are known values – Create an equation using ‘k’ – Formulate y = kx using the calculated ‘k’ – Solve direct variation problems – Apply the equation to find unknown variables | This slide is aimed at guiding students through the process of writing and solving direct variation equations. Start by explaining the concept of direct variation, where one variable changes directly as the other. Emphasize the importance of the constant of variation ‘k’, and show how to calculate it using known values of x and y from a given set of data. Once ‘k’ is found, instruct students on how to create a direct variation equation of the form y = kx. Finally, provide practice problems for students to apply these steps and solve for unknown variables, reinforcing their understanding of direct variation.

Solving Direct Variation Problems – Apply direct variation equation – Example: k = 4, find y for x = 5 – Using y = kx, substitute k = 4 and x = 5 to find y – Practice: k = 2.5, find y for x = 8 – Try it yourself with k = 2.5 and x = 8 – Understand the relationship – Direct variation means y increases as x increases | This slide focuses on applying the direct variation equation y = kx to solve problems. Start by explaining the concept of direct variation, where y varies directly as x. The constant of variation ‘k’ represents the rate at which y changes with respect to x. In the example, students will substitute k = 4 and x = 5 into the equation to find y. For the practice problem, students will apply what they’ve learned to find y when k = 2.5 and x = 8. Emphasize the proportional relationship between x and y in direct variation. Encourage students to explain the steps they take to solve the problems and to understand how changes in x affect y.

Real-World Applications of Direct Variation – Understanding direct variation – Speed relates to travel time – If speed is constant, travel time varies directly with distance. – Explore everyday examples – Consider examples like fuel consumption, cooking recipes, and shopping budgets. – Class discussion on applications | This slide aims to show students how direct variation is present in everyday scenarios and to help them understand the concept through tangible examples. Direct variation means two variables change in the same way; if one increases, the other does too. A classic example is speed and travel time: the faster you go, the less time a trip takes, assuming constant speed. Encourage students to think of other examples where one quantity changes as another does, such as ingredients in a recipe or the amount of money spent in relation to the number of items bought. During the class discussion, prompt students to identify variables in their examples and explain the direct variation relationship. This will solidify their understanding and help them recognize direct variation in the world around them.

Class Activity: Direct Variation Challenge – Pair up and find the constant of variation – Formulate a direct variation equation – Solve a real-world problem using your equation – Example: If y varies directly with x and y=8 when x=2, find y when x=10. – Present your findings to the class | This activity is designed to reinforce the concept of direct variation through hands-on practice. Students will work in pairs to analyze sets of data and determine the constant of variation (k), which is the ratio of y to x (y/x). Once they have found k, they will use it to create an equation of the form y = kx. Then, they will apply their equation to a real-world scenario, such as calculating the cost of items or the distance traveled over time, to solve a problem. Finally, each pair will present their equation and how they used it to solve the problem, allowing for peer learning. Possible activities: 1) Calculating the cost of fruits per pound, 2) Determining the speed of a car over time, 3) Figuring out the amount of ingredients needed for a recipe based on servings, 4) Estimating the height of a plant as it grows over weeks, 5) Computing the time it takes to download data based on internet speed.

Review and Q&A: Direct Variation – Recap today’s key points Direct variation: y = kx, where k is the constant of variation. – Open floor for questions – Offer more examples For instance, if y varies directly with x and y = 10 when x = 2, find k. – Encourage participation Ask students to come up with questions or points they want to discuss. | This slide is meant to consolidate the students’ understanding of direct variation and to clarify any doubts they might have. Begin by summarizing the main concept of direct variation, emphasizing the equation y = kx, where k is the constant of variation. Encourage students to ask questions they may have about the lesson, providing a safe space for them to seek clarification. Be prepared to provide additional examples to help solidify their understanding. For example, if a car travels at a constant speed, the distance covered varies directly with the time traveled. Use this time to engage with the students, ensuring they feel comfortable with the material before moving on.