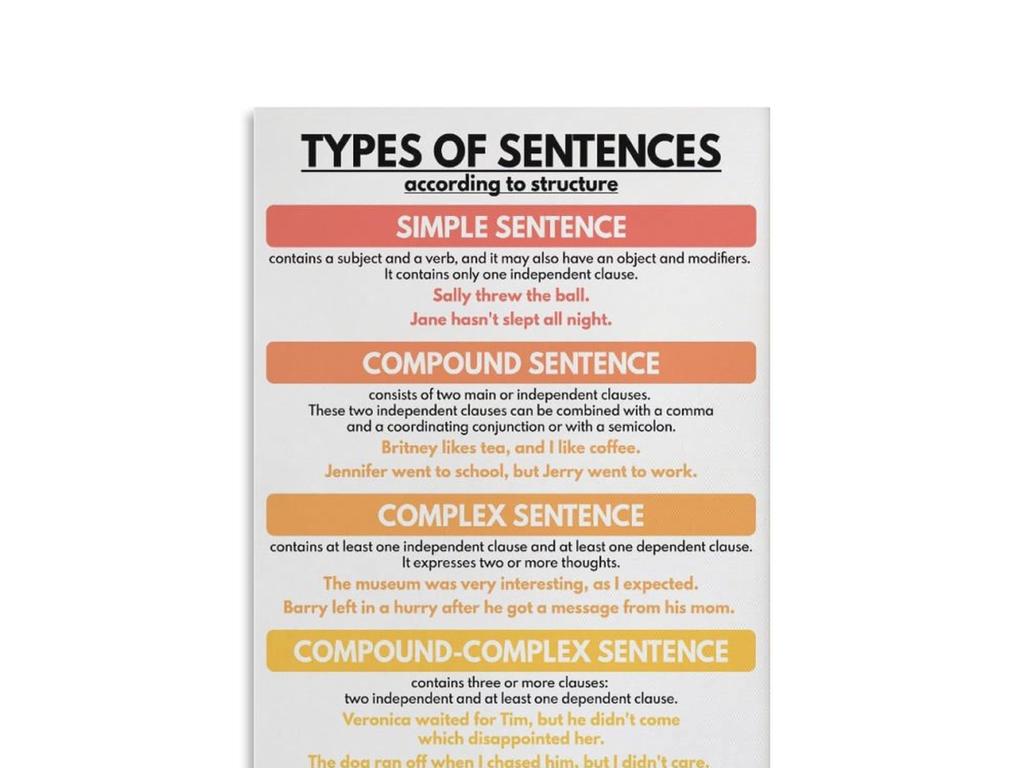
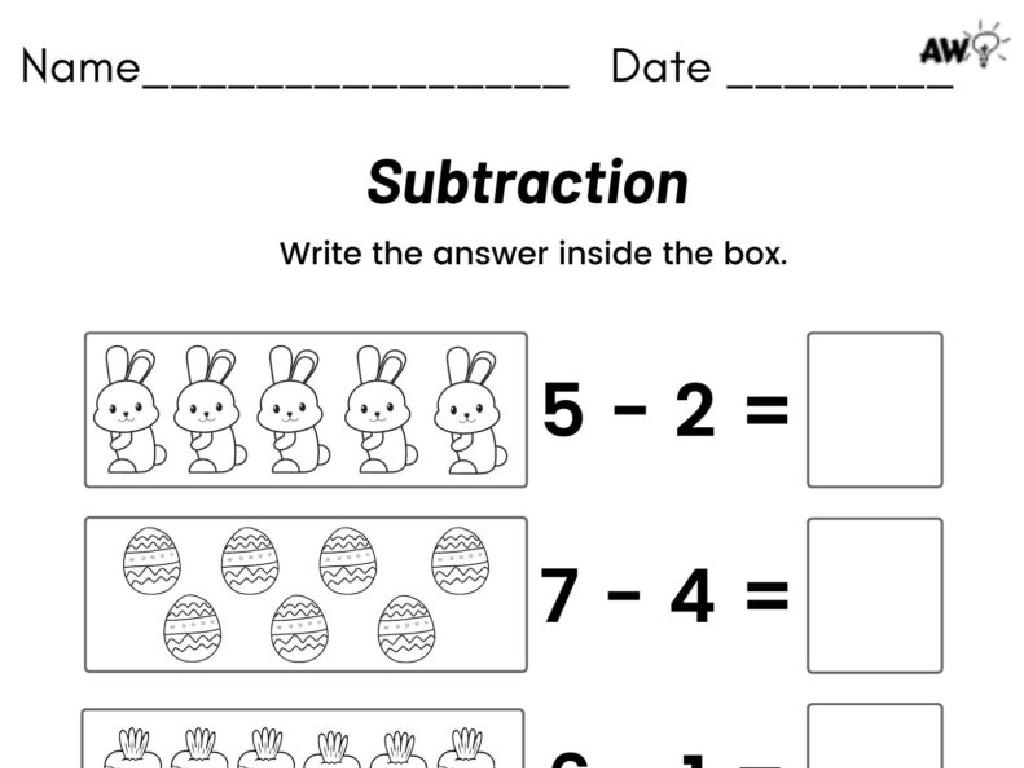
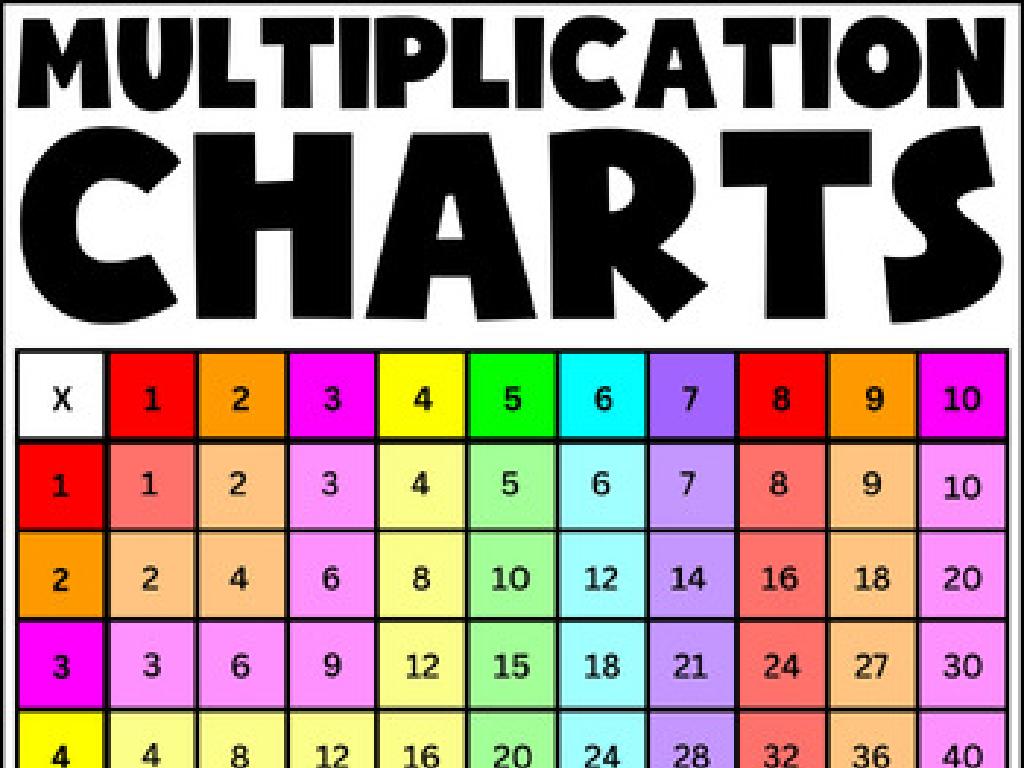
Divide 2-Digit Numbers By 1-Digit Numbers Using Area Models
Subject: Math
Grade: Fourth grade
Topic: Divide By One-Digit Numbers
Please LOG IN to download the presentation. Access is available to registered users only.
View More Content
Welcome to Division: Sharing Equally
– Division as equal sharing
– Division splits a number into equal parts
– Meaning of division
– To divide is to split into equal groups
– Division in daily life
– Sharing snacks, dividing toys among friends
– Area models for division
– Visual way to see division using rectangles
|
This slide introduces the concept of division to fourth-grade students by relating it to the idea of sharing equally. Begin by explaining that division is a way of splitting a whole into equal parts or groups. Use relatable examples such as sharing snacks among a group of friends or dividing toys to ensure everyone gets the same amount. Emphasize that division is not just a math operation but a skill used in everyday life. Introduce area models as a visual tool that helps students understand division by representing numbers as areas of rectangles. This will set the foundation for more complex division problems and help students visualize the division process.
Recap: Multiplication & Division Relationships
– Review multiplication facts
– Quick recall of times tables is essential
– Relate multiplication & division
– Division is finding how many times a number is contained within another number
– Understand ‘undoing’ multiplication
– Division can reverse a multiplication problem
|
Begin the lesson with a quick review of multiplication facts to ensure students are comfortable with their times tables, as this is crucial for understanding division. Explain how multiplication and division are inverse operations, meaning they ‘undo’ each other. For example, if 3 x 4 = 12, then 12 ÷ 4 = 3. Emphasize that division is essentially asking how many times a divisor fits into the dividend. This foundational understanding will help students grasp the concept of using area models for division, as they will see how these operations are interconnected. Use simple examples to illustrate these points and prepare students for applying this knowledge to area models for division.
Dividing 2-Digit Numbers by 1-Digit Numbers
– Step-by-step division process
– Break down the number into smaller parts that you can divide.
– Use multiplication to divide
– Remembering your times tables can make division easier.
– Example: Divide 56 by 7
– 56 divided by 7 equals 8. How do we get there using an area model?
– Practice with area models
|
This slide introduces the concept of dividing 2-digit numbers by 1-digit numbers using area models. Start by explaining the step-by-step process of division, breaking down the larger number into more manageable parts. Emphasize the importance of multiplication facts in making division simpler, as knowing the inverse operation is key to understanding division. Use the example of 56 ÷ 7 to show how to set up an area model and visually represent the division process. Encourage students to practice with different numbers and use area models to solidify their understanding. Provide additional examples and practice problems for students to work on as homework.
Introduction to Area Models for Division
– What is an area model?
– A visual representation of multiplication and division
– Area models aid division understanding
– Breaks down division into manageable parts
– Rows and columns in area models
– Rows represent the divisor, columns form the dividend
– Practice with simple examples
|
Begin by explaining that an area model is a visual tool that helps us understand and solve multiplication and division problems. It’s like a rectangle divided into smaller parts, where the length and width represent the factors in multiplication or the dividend and divisor in division. Show how area models can simplify division by breaking it into smaller, more manageable chunks, making it easier for students to see the relationship between multiplication and division. Illustrate the concept of rows and columns by using an area model to divide a 2-digit number by a 1-digit number, and walk through a simple example step by step. Encourage students to draw their own area models and use them to solve division problems.
Dividing with Area Models
– Create an area model for 56 ÷ 7
– Draw a rectangle, label one side 56 and divide by 7
– Break down 56 into smaller parts
– Example: 56 can be broken into 50 and 6
– Fill the model with rows and columns
– Each row represents a group of 7 in the model
– Understand division through area
|
This slide introduces the concept of using area models to visualize division. Start by explaining that an area model is a rectangle that represents the dividend, in this case, 56. Show how to label one side of the rectangle with the total amount (56) and the other side with the divisor (7). Then, demonstrate how to break down the dividend into more manageable parts (such as 50 and 6) that can be easily divided by the divisor. Illustrate how to fill in the area model with rows and columns, where each row represents a group of 7. This visual method helps students understand how division works by organizing the dividend into equal parts. Encourage students to practice with different dividends and divisors to become comfortable with the concept.
Division Practice with Area Models
– Divide 48 by 6 using an area model
– How many 6s fit into 48? Draw it out.
– Class activity: 72 divided by 8
– Let’s solve 72 ÷ 8 as a team, step by step.
– Tips for verifying answers
– Use multiplication to check your division result.
– Practice makes perfect!
|
This slide is aimed at providing students with hands-on practice in dividing 2-digit numbers by 1-digit numbers using area models. Start by guiding students through the process of dividing 48 by 6, illustrating how to draw an area model and partition it into equal parts. Then, engage the whole class in a collaborative effort to divide 72 by 8, encouraging students to participate in drawing the model and discussing each step. Emphasize the importance of checking work by multiplying the divisor by the quotient to see if it equals the dividend. Provide additional practice problems for students to work on independently, and encourage them to use the tips provided to check their answers.
Class Activity: Division with Area Models
– Pair up and solve division problems
– Create area models for solutions
– Draw rectangles to represent problems, divide into equal parts
– Present models to the class
– Discuss strategies and solutions
– Compare different methods used by classmates
|
This interactive class activity is designed to help students understand the concept of division using area models. Students will pair up, which encourages collaboration and peer learning. They will solve division problems by drawing area models, which are rectangles divided into smaller, equal parts that represent the division process. After solving the problems, each pair will present their area model to the class, explaining their thought process and how they used the model to find the answer. This presentation will allow students to articulate their understanding and demonstrate their problem-solving skills. Finally, the class will discuss the various strategies and solutions, providing an opportunity for students to learn from each other and see different approaches to the same problem. The teacher should circulate during the activity, provide guidance as needed, and facilitate the final discussion to ensure a comprehensive understanding of the topic.
Wrapping Up: Division with Area Models
– Review of area model division
– Practice is key to mastery
– Homework: 5 area model problems
– Solve using the steps we learned today
– Share solutions next class
– Be ready to discuss how you solved them
|
As we conclude today’s lesson, it’s important to recap the steps of dividing 2-digit numbers by 1-digit numbers using area models. Emphasize to students that practicing these problems will help solidify their understanding of the concept. For homework, they are to complete 5 division problems using the area model method. Encourage them to follow the steps discussed in class and remind them that they will have the opportunity to share their solutions and methods in the next class, fostering a collaborative learning environment. This will also allow you to assess their grasp of the concept and provide additional support where needed.