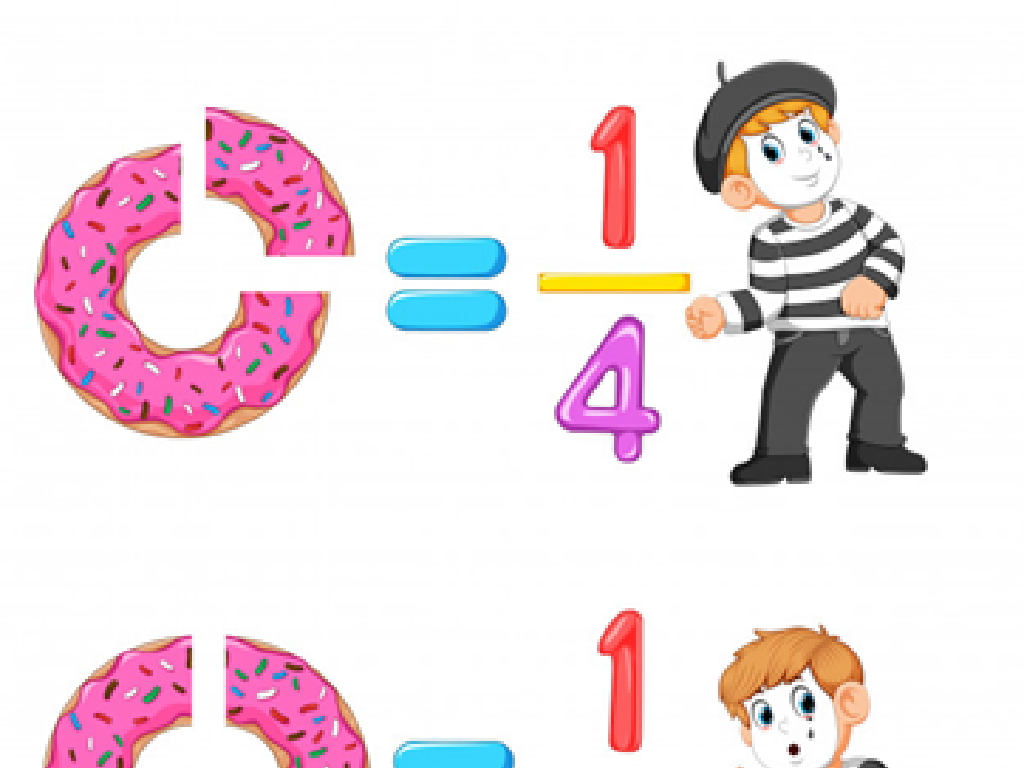Your next great Lessn starts here.

Welcome to Equivalent Fractions! – Understanding basic fractions – Fractions represent parts of a whole – Exploring equivalent fractions – Fractions with different numbers but same value – Significance of equivalent fractions – Useful for comparing and simplifying fractions – Practical examples – Using fractions in recipes or dividing a pizza | This slide introduces the concept of equivalent fractions to fourth-grade students. Begin by explaining what fractions are, emphasizing that they represent parts of a whole. Introduce equivalent fractions as different fractions that represent the same amount. Discuss why understanding equivalent fractions is crucial for skills like comparing fractions and simplifying calculations. Use relatable examples such as adjusting recipes or evenly dividing a pizza among friends to illustrate the concept. Encourage students to think of other situations where they might use equivalent fractions. The goal is to help students recognize equivalent fractions in various contexts and understand their practical applications.

Understanding Fractions – A fraction represents part of a whole – Consists of a numerator and denominator – Example: 1/2 is one out of two parts – If a pizza is cut into 2 pieces, 1/2 is one slice – Equivalent fractions have the same value – 2/4 is also one out of two parts, like 1/2 | Begin the lesson by explaining that a fraction represents a part of a whole, such as a slice of pizza. The top number, or numerator, indicates how many parts we have, while the bottom number, or denominator, shows how many equal parts the whole is divided into. Use visual aids like a circle (pizza) to demonstrate 1/2. Then, introduce the concept of equivalent fractions by showing that 2/4 is the same as 1/2, using the pizza example cut into 4 pieces, where 2 slices represent the same amount as 1 slice from a pizza cut into 2 pieces. This will help students visualize and understand that different fractions can represent the same quantity.

Visualizing Fractions – Fractions as visual models – Pie charts show parts of a whole – Imagine cutting a pie into equal slices. Each slice is a fraction of the pie. – Bar models divide objects into equal parts – Think of a chocolate bar split into equal sections. Each section is a part of the chocolate bar. – Examining examples of equivalent fractions – Let’s explore how 1/2 is the same as 2/4 by drawing and comparing. | This slide aims to help students understand fractions by visualizing them. Using pie charts, we can illustrate how a whole is divided into equal parts, with each part representing a fraction. Similarly, bar models can show how objects can be split into equal sections, each representing a fraction of the object. By looking at examples together, students can see how different fractions can actually be equivalent, such as 1/2 and 2/4, when they represent the same portion of a whole. Encourage students to draw their own pie charts and bar models to represent equivalent fractions and share their examples with the class.

Understanding Equivalent Fractions – What are equivalent fractions? – Fractions that have the same value – Example: 1/2 equals 2/4 and 3/6 – 1/2 is the same as 2/4 or 3/6 – Different but same size – How to find equivalent fractions? – Multiply or divide numerator and denominator by the same number | This slide introduces the concept of equivalent fractions to fourth-grade students. Start by explaining that equivalent fractions are different ways to represent the same portion of a whole. Use visual aids like pie charts or fraction bars to show that 1/2, 2/4, and 3/6 cover the same amount of space, even though the numbers are different. Emphasize that the size or the value of the fraction doesn’t change, only the way we write it. Teach them the method to find equivalent fractions by multiplying or dividing both the numerator and denominator by the same number. Provide several examples and encourage students to create their own equivalent fractions during practice.

Creating Equivalent Fractions – Multiply or divide both top and bottom – Use the same number for numerator and denominator – Keeps the fraction’s value the same – It’s like creating a ‘fraction twin’ – Example: 1/2 becomes 2/4 – Multiply 1/2’s top and bottom by 2 to get 2/4 – Practice with different numbers – Try using 3, 4, or 5 to make more equivalents | When teaching equivalent fractions, emphasize that multiplying or dividing both the numerator (top number) and the denominator (bottom number) by the same non-zero number will result in a fraction equivalent to the original. This concept is crucial as it does not change the value of the fraction, just its appearance. Use concrete examples, such as 1/2, and visually show how multiplying both parts by 2 yields 2/4, an equivalent fraction. Encourage students to practice with different numbers to build their understanding and confidence. Provide several examples and possibly incorporate visual aids like fraction bars or circles to help students visualize the concept.

Practice Finding Equivalent Fractions – Find fractions equal to 1/3 – Multiply top and bottom by the same number – For example, multiply both by 2 to get 2/6 – Discover new equivalent fractions – Can you find more like 3/9, 4/12, 5/15? – Share your fractions with the class | This slide is aimed at helping students practice the concept of equivalent fractions. Start by using 1/3 as a base example. Explain that multiplying the numerator (top number) and the denominator (bottom number) by the same number will give a fraction equivalent to 1/3. Encourage the students to use different numbers to multiply and find new fractions that are equivalent to 1/3. As they come up with answers like 2/6, 3/9, 4/12, and so on, have them share their findings with the class. This will reinforce their understanding of equivalent fractions and provide practice in calculating them. The activity will also help students recognize patterns in numbers and improve their multiplication skills.

Simplifying Fractions – Simplify to find equivalents – Divide by greatest common factor – The largest number that divides both numerator & denominator – Example: Simplify 4/8 – 4 is the GCF of 4 and 8 – 4/8 becomes 1/2 when divided by 4 – Both top and bottom numbers are divided by 4 | This slide introduces the concept of simplifying fractions as a method to find equivalent fractions. It’s important to explain that equivalent fractions are different fractions that represent the same value. Simplifying a fraction involves dividing the numerator (top number) and the denominator (bottom number) by their greatest common factor (GCF). The GCF is the largest number that can divide both numbers without leaving a remainder. For example, the GCF of 4 and 8 is 4. When we divide both the numerator and denominator of 4/8 by 4, we get the simplified fraction 1/2. Encourage students to practice this process with different fractions and to always look for the GCF to simplify fractions effectively.

Equivalent Fractions in Real Life – Understanding equivalent fractions – Fractions that are different but represent the same amount – Pizza slice fractions – A pizza cut into 4 or 8 slices can show 1/2 – Sharing with friends – If 3 friends share 6 apples, each gets 2/6, same as 1/3 – Everyday examples | This slide aims to help students recognize equivalent fractions in everyday situations, reinforcing the concept that fractions can look different but still represent the same value. Use the example of a pizza to illustrate how the same pizza can be divided into various numbers of slices, yet some fractions will still show the same amount of pizza. For instance, 2 out of 4 slices is the same as 4 out of 8 slices. When sharing items equally among friends, like splitting 6 apples among 3 friends, students can see that each person gets 2/6 of the apples, which is equivalent to 1/3. Encourage students to think of other daily examples where they might encounter equivalent fractions, such as measuring ingredients in cooking or dividing a pack of stickers.

Class Activity: Fraction Bingo – Let’s play Fraction Bingo! – Receive your unique bingo card – Each card has different fractions – Listen for equivalent fractions – I’ll call out fractions; find the equivalent on your card – Mark your card for matches – Use a marker to cover the correct fraction – Aim for five in a row to win! | This interactive activity is designed to help students recognize and find equivalent fractions in a fun and engaging way. Distribute the bingo cards, ensuring each student has a unique set of fractions. As you call out various fractions, students will need to determine if they have an equivalent fraction on their card and mark it. This game encourages quick thinking and reinforces the concept of equivalent fractions. Be prepared with small prizes for the winners to make the game exciting. Possible variations of the activity could include having students call out the fractions, using visual fraction representations, or creating themed bingo cards.