Your next great Lessn starts here.

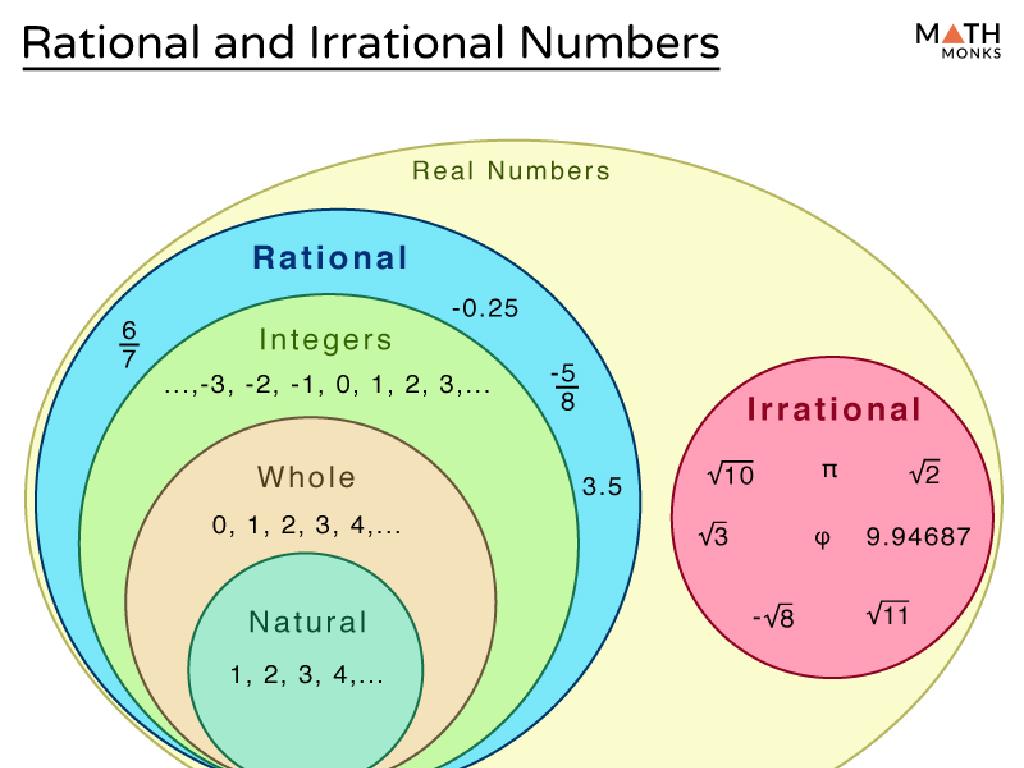
Exploring Prime and Composite Numbers – What are prime numbers? – A prime number has only two factors: 1 and itself, like 2, 3, 5, 7… – What are composite numbers? – Composite numbers have more than two factors, such as 4, 6, 8, 9… – Prime and composite numbers up to 100 – Let’s list primes and composites between 1-100 – Significance in mathematics – These numbers are the basics for understanding math concepts like LCM and GCD | This slide introduces the fundamental concepts of prime and composite numbers, which are essential for understanding higher-level math topics. Begin by defining prime numbers as those that can only be divided evenly by 1 and themselves, with examples like 2, 3, 5, and 7. Then, explain that composite numbers have more than two factors, providing examples like 4, 6, 8, and 9. Encourage students to identify and list prime and composite numbers up to 100, reinforcing their understanding of factors and multiples. Emphasize the importance of these concepts as they form the building blocks for learning about least common multiples (LCM) and greatest common divisors (GCD). Prepare interactive activities where students can practice identifying and categorizing numbers to solidify their comprehension.

Exploring Factors – Understanding factors – Factors are numbers multiplied to get another number. – Example: Factors of 6 – 1, 2, 3, and 6 are factors of 6 because 1×6=6 and 2×3=6. – Activity: Find factors of 8 – Work with a partner to list factors of 8. | This slide introduces the concept of factors in mathematics. Begin by explaining that factors are numbers that, when multiplied together, result in another number. Use 6 as an example to illustrate this point, showing that 1, 2, 3, and 6 are all factors of 6 because they can be paired in multiplication to equal 6. For the class activity, have students pair up and use multiplication to find all the factors of 8. This exercise will help solidify their understanding of factors through hands-on practice. Possible factors for 8 include 1, 2, 4, and 8. Encourage students to check their answers by multiplying the factors to see if they get 8. This activity promotes collaboration and application of the concept of factors.

Exploring Multiples – Multiples from multiplication – When we multiply a number by 1, 2, 3, etc., we get its multiples. – Example: Multiples of 5 – Like 5 x 1 = 5, 5 x 2 = 10, up to 5 x 5 = 25. – Activity: Find multiples of 7 – Use your whiteboard to list 7 x 1, 7 x 2, 7 x 3, and 7 x 4. | This slide introduces the concept of multiples to fourth-grade students. Begin by explaining that multiples are the result of multiplying a number by integers (whole numbers). Use the example of 5 to show the first five multiples, which helps students visualize the concept. For the activity, instruct students to write the first four multiples of 7 on their whiteboards, which will be 7, 14, 21, and 28. This hands-on activity reinforces their understanding of multiplication and the concept of multiples. As they work, circulate the room to offer guidance and ensure understanding. Encourage students to share their answers with the class to foster a collaborative learning environment.

Exploring Prime Numbers – Prime numbers have two factors – A number is prime if it’s only divisible by 1 and itself – Examples: 2, 3, 5, 7, 11… – These are the first few primes under 100 – 2 is the sole even prime – Every even number is divisible by 2, but 2 is prime because it has no other divisors – Primes are greater than 1 | This slide introduces the concept of prime numbers to fourth-grade students. Prime numbers are defined as numbers that have exactly two distinct factors: 1 and the number itself. Provide examples of prime numbers less than 100 to help students recognize patterns. Emphasize that 2 is unique as the only even prime number since all other even numbers can be divided by 2 and thus have at least one additional factor. Encourage students to understand that all prime numbers are greater than 1. You can use this opportunity to discuss why 1 is not considered a prime number. To reinforce learning, ask students to identify prime numbers from a list of numbers up to 100.

Exploring Composite Numbers – Definition of composite numbers – Numbers with more than two different factors – Examples of composite numbers – 4 has factors 1, 2, 4; 6 has 1, 2, 3, 6; 8 has 1, 2, 4, 8 – More than two factors – If a number has factors other than 1 and itself, it’s composite – Non-prime numbers over 1 – Every number >1 that’s not prime is composite | This slide introduces the concept of composite numbers to fourth-grade students. Composite numbers are defined as numbers that have more than two factors. Provide examples such as 4, 6, and 8, and list their factors to illustrate the concept. Explain that in addition to 1 and the number itself, composite numbers have other divisors. Emphasize that all numbers greater than 1 that are not prime are considered composite. This will help students differentiate between prime and composite numbers. Encourage students to identify and list factors of given composite numbers to reinforce their understanding.

Prime or Composite? – Practice identifying numbers – Is 9 prime or composite? – 9 can be divided by 1, 3, and 9. So, it’s composite! – Explore if 23 is prime or composite – 23 can only be divided by 1 and itself, making it prime. – Understanding prime and composite – Prime numbers have only two factors; composite numbers have more than two. | This slide is aimed at helping students practice the concept of identifying prime and composite numbers. Start by explaining that a prime number has exactly two distinct positive divisors: 1 and itself. On the other hand, a composite number has more than two divisors. Use 9 and 23 as examples to illustrate the concept. For 9, show that it can be divided evenly by 1, 3, and 9, which makes it composite. For 23, demonstrate that no other number except 1 and 23 can divide it without leaving a remainder, confirming it’s prime. Encourage students to use this knowledge to classify other numbers as prime or composite and prepare a few more examples for them to practice.

Discovering Patterns in Prime and Composite Numbers – Patterns in prime numbers – Primes only have 2 factors, 1 and themselves. Notice any patterns? – Composite numbers patterns – Composites have more than 2 factors. Can you find a pattern? – Discuss findings with a classmate – Share observations with the class | This slide aims to engage students in recognizing patterns within prime and composite numbers up to 100. Encourage them to observe that prime numbers, except for 2, are all odd and cannot be evenly divided by other numbers. Ask them to look for patterns in the spacing between primes. For composite numbers, guide them to see that they have multiple factors and may follow multiplication tables. Have students pair up to discuss their observations, fostering collaborative learning. After the discussion, invite students to share their findings with the class to reinforce their understanding and to learn from each other’s observations.

Class Activity: Prime and Composite Hunt – Explore numbers up to 100 – Classify numbers as prime or composite – Prime numbers have only two factors: 1 and the number itself – Work in teams on a number hunt – 10 minutes to find as many as possible | This activity is designed to help students understand the concept of prime and composite numbers through an interactive classroom hunt. Divide the class into small teams and assign each team different ranges of numbers to search for within the classroom. Provide them with a chart or a worksheet to classify each number they find as either prime or composite. Encourage them to use divisibility rules to determine the classification quickly. After 10 minutes, regroup and discuss the findings. Possible variations of the activity could include: 1) Using number cards hidden around the room, 2) Assigning each team a specific set of numbers to find, or 3) Creating a competition to see which team can classify the most numbers correctly. This hands-on approach will help solidify their understanding of prime and composite numbers.

Conclusion: Prime and Composite Numbers – Recap on prime numbers – Prime numbers have only two factors: 1 and themselves – Recap on composite numbers – Composite numbers have more than two factors – Significance in mathematics – Quick quiz on prime/composite – Is 37 prime or composite? How about 51? | As we wrap up today’s lesson, let’s review what we’ve learned. Prime numbers are special numbers that can only be divided by 1 and themselves without leaving a remainder. Examples include 2, 3, 5, 7, 11, and so on. Composite numbers, on the other hand, have more than two factors and can be divided evenly by numbers other than 1 and themselves, like 4, 6, 8, 9, etc. Understanding these concepts is crucial because they are the building blocks of our number system and are used in various areas of mathematics, including factoring and understanding least common multiples. To test our knowledge, let’s answer this quick quiz: Is 37 prime or composite? How about 51? Remember, to determine if a number is prime or composite, think about whether it has factors other than 1 and itself. For the teacher: Have students raise their hands to answer the quiz and explain their reasoning. This will help reinforce their understanding and provide an opportunity for peer learning.