Your next great Lessn starts here.

Converting Improper Fractions & Mixed Numbers – Recap on fractions basics – Fractions represent parts of a whole – Define mixed numbers – Mixed numbers: Whole number + fraction – Define improper fractions – Improper fractions: Numerator > denominator – Conversion process – Steps to convert between the two types | Begin the lesson with a quick review of fractions to ensure students recall that fractions represent parts of a whole. Introduce mixed numbers as a combination of a whole number and a fraction, and improper fractions as fractions where the numerator is larger than the denominator. Explain the process of conversion: for mixed to improper, multiply the whole number by the denominator, add the numerator, and write the result over the original denominator. For improper to mixed, divide the numerator by the denominator for the whole number, and the remainder becomes the new numerator over the original denominator. Provide examples and practice problems to solidify understanding.

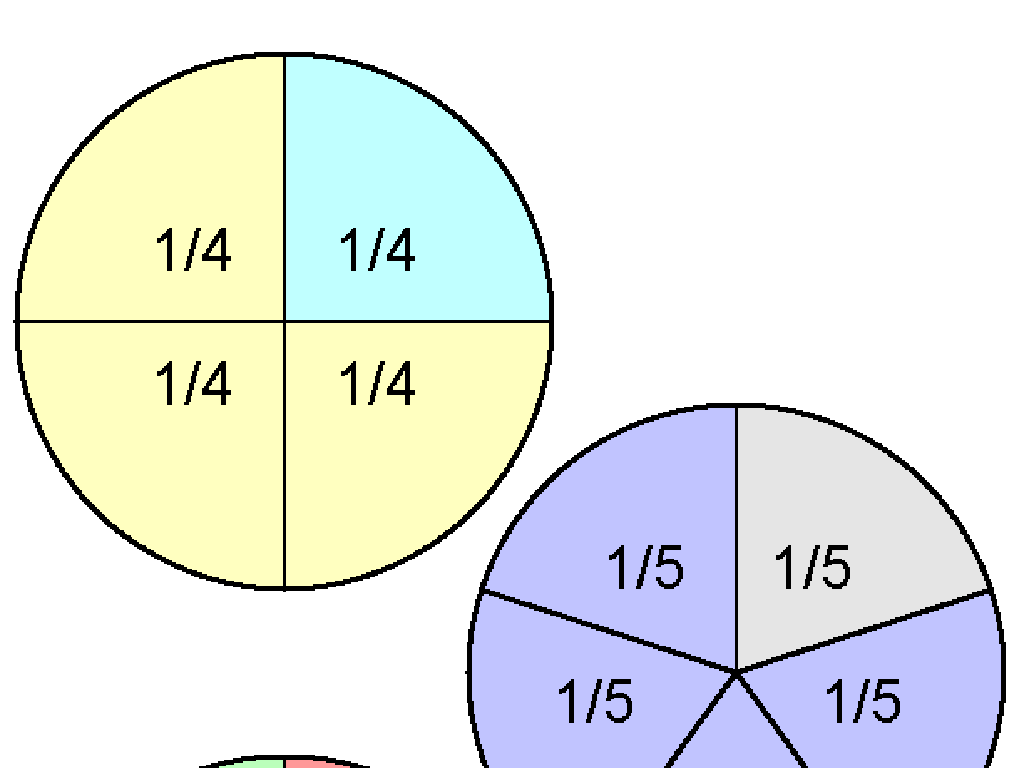
Understanding Fractions – What is a fraction? – A fraction represents a part of a whole. – Numerator vs. denominator – Numerator: top number, Denominator: bottom number. – Examples of proper fractions – 1/2 (half), 3/4 (three quarters), 5/8 (five eighths). | This slide introduces the concept of fractions to fifth-grade students. Begin by explaining that a fraction represents a part of a whole or a division of a whole into equal parts. The numerator, which is the top number of a fraction, indicates how many parts we have. The denominator, the bottom number, tells us into how many parts the whole is divided. Provide examples of proper fractions, where the numerator is less than the denominator, to help students visualize and understand the concept. Use visual aids like pie charts or fraction bars if possible to illustrate these examples. Encourage students to think of fractions they encounter in everyday life, such as cutting a pizza or sharing candy.

Understanding Mixed Numbers – Define mixed numbers – A number made up of a whole number and a fraction, like 2 1/3. – Parts of a mixed number – Consists of a whole number part and a fractional part, e.g., 3 (whole) + 1/4 (fraction). – Real-life mixed number examples – Used in cooking (1 1/2 cups of sugar), or measuring (5 3/4 inches of ribbon). – Practice with mixed numbers | This slide introduces students to mixed numbers, which combine whole numbers with fractions to represent quantities greater than one but less than two whole units. Emphasize that the whole number and the fraction are distinct parts that make up the mixed number. Provide relatable examples such as cooking measurements or lengths in inches to illustrate the concept. Encourage students to think of other examples where they encounter mixed numbers in their daily lives. The practice component should involve converting between improper fractions and mixed numbers to solidify their understanding.

Understanding Improper Fractions – Definition of improper fractions – A fraction where the numerator is greater than or equal to the denominator, e.g., 7/4 – Identifying numerators & denominators – Numerator is the top number, denominator is the bottom number – Real-world improper fraction examples – Examples: Slices of pizza left vs. whole pizzas, hours worked vs. a full day – Converting to mixed numbers – Divide the numerator by the denominator to convert, e.g., 7/4 = 1 3/4 | This slide introduces students to improper fractions, which are fractions where the numerator (top number) is larger than or equal to the denominator (bottom number). Use visual aids like pie charts or pizza slices to help students identify and understand improper fractions in a real-world context. Explain how to find the numerator and denominator in an improper fraction. Provide relatable examples, such as dividing a set of pizza slices among friends or comparing hours worked to a full day. Finally, demonstrate how to convert an improper fraction to a mixed number by dividing the numerator by the denominator and explaining the remainder as a new fraction.

Converting Improper Fractions to Mixed Numbers – Step-by-step conversion guide – Divide numerator by denominator – If 7/4, divide 7 by 4. – Quotient becomes the whole number – For 7/4, quotient is 1; it’s the whole number. – Remainder forms the new fraction – The remainder 3 is the numerator; denominator stays 4. | This slide is aimed at teaching fifth graders the process of converting improper fractions to mixed numbers. Start by explaining that an improper fraction has a numerator larger than its denominator. Then, guide them through the division process: the numerator is divided by the denominator. The quotient (whole number result) becomes the whole number part of the mixed number, and the remainder becomes the numerator of the fractional part. The denominator remains the same. Use examples like 7/4 to illustrate the process: 7 divided by 4 equals 1 with a remainder of 3, so the mixed number is 1 3/4. Encourage students to practice with different improper fractions to solidify their understanding.

Converting Mixed Numbers to Improper Fractions – Why convert to improper fractions? – To simplify calculations or compare fractions – Multiply whole number by denominator – If 2 1/3, multiply 2 (whole number) by 3 (denominator) – Add the numerator to the product – After multiplying, add the numerator: 6 (from step 2) + 1 – Place result over original denominator – The improper fraction is 7/3, keeping the denominator the same | Understanding how to convert mixed numbers to improper fractions is crucial for performing operations like addition, subtraction, multiplication, and division with fractions. Start by multiplying the whole number part of the mixed number by the denominator of the fractional part. Then, add the numerator to this product. The sum becomes the new numerator, while the denominator remains unchanged. For example, to convert 2 1/3 to an improper fraction, multiply 2 by 3 to get 6, add the numerator 1 to get 7, and place it over the original denominator to get 7/3. This process is essential for fifth graders to master as it lays the foundation for more advanced math concepts.

Let’s Practice Together: Conversion Fun – Convert improper fraction to mixed number – Example: 7/4 becomes 1 3/4 – Convert mixed number to improper fraction – Example: 2 1/3 becomes 7/3 – Group practice with examples Work together to understand steps – Guided assistance for all Teacher will help with tricky parts | This slide is designed for a collaborative classroom activity where students will practice converting between improper fractions and mixed numbers. Start with an example of converting an improper fraction, such as 7/4, to a mixed number by dividing the numerator by the denominator to find the whole number, and then using the remainder as the new numerator. Next, show how to convert a mixed number, like 2 1/3, back into an improper fraction by multiplying the whole number by the denominator and adding the numerator. Organize the class into small groups and provide them with a set of practice problems. Circulate around the room, offering guidance and support as needed. This hands-on activity will help solidify the students’ understanding of the concepts and provide an opportunity for immediate feedback.

Class Activity: Fraction Conversion Challenge – Pair up with a classmate – Convert mixed numbers to improper fractions – Use the formula: mixed number = whole number + (numerator/denominator) – Convert improper fractions to mixed numbers – Use the formula: improper fraction = (whole number * denominator) + numerator / denominator – Share and explain your answers | This activity is designed to encourage collaboration and reinforce the concept of converting between mixed numbers and improper fractions. Provide each pair with a worksheet containing a list of mixed numbers and improper fractions. Students should work together to convert each number to the other form using the appropriate formulas. After completing the worksheet, pairs will share their answers with the class and explain the process they used to arrive at their solutions. This will help students articulate their understanding and learn from each other’s strategies. For the teacher: Prepare diverse examples to cater to different skill levels, and be ready to assist pairs that may struggle with the concept. Encourage students to think about the visual representation of the fractions as they convert them.

Wrapping Up: Fractions Mastery – Review of fraction conversions – Why conversion skills matter – Understanding conversions helps in math and real-life problems. – Homework: Conversion worksheet – Complete the worksheet to practice converting between improper fractions and mixed numbers. – Keep practicing at home! | As we conclude today’s lesson, it’s important to recap the steps for converting between improper fractions and mixed numbers. Emphasize the importance of mastering these skills, as they are not only crucial for advanced math concepts but also for solving everyday problems. For homework, students are assigned a worksheet that provides additional practice on these conversions. Encourage them to attempt all problems and remind them that practice is key to becoming confident in their conversion skills. The worksheet should be designed to cater to various difficulty levels, ensuring that all students can participate and improve.