Your next great Lessn starts here.

Welcome to Integers! – Understanding Integers – Integers include whole numbers and their opposites. – Real-life integer applications – Temperatures, bank transactions, and elevations. – Positive vs. Negative Numbers – Positive numbers are above zero, negatives below. – Adding and Subtracting Rules – Same signs add and keep, different signs subtract. | Introduce the concept of integers as not just whole numbers, but also their opposites, including negative numbers. Provide relatable examples such as temperatures (above/below freezing), bank transactions (deposits/withdrawals), and elevations (above/below sea level) to illustrate the use of integers in everyday life. Clarify the difference between positive and negative numbers, emphasizing their placement on the number line. Explain the basic rules for adding and subtracting integers: when the signs are the same, add the numbers and keep the sign; when the signs are different, subtract the smaller number from the larger and keep the sign of the larger number. This foundational knowledge will be crucial for understanding more complex mathematical concepts involving integers.

Understanding Integers – Integers: Positive and Negative – Whole numbers without fractions; includes negative numbers. – Visualizing with a Number Line – A line with numbers where positions represent values. – Zero: The Neutral Integer – Zero is neither positive nor negative; it’s the starting point. – Adding and Subtracting Rules – Use the number line to add (move right) and subtract (move left). | Integers are the set of whole numbers that include all positive numbers, negative numbers, and zero. They do not include fractions or decimals. A number line is a helpful tool for visualizing integers; it allows students to understand the position and value of numbers in relation to zero. Zero is considered the neutral integer and acts as a separator between positive and negative numbers. When adding and subtracting integers, the number line can be used to demonstrate movement to the right for addition and to the left for subtraction. Emphasize the importance of understanding these concepts as a foundation for all operations with integers.

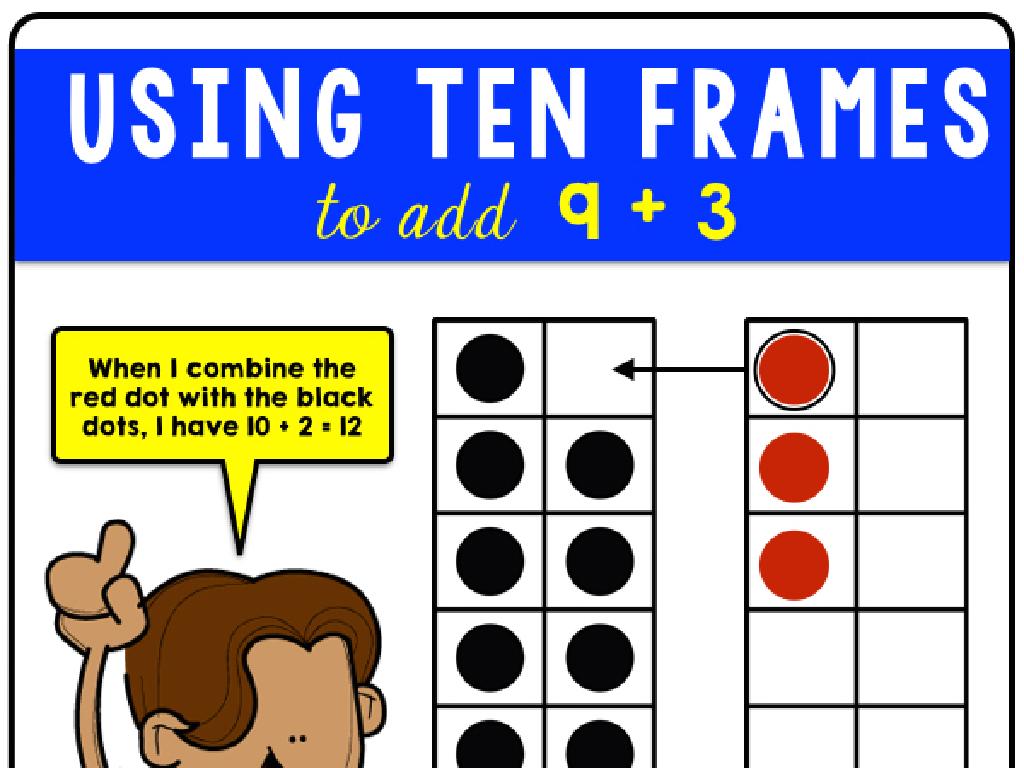
Adding Integers – Rules for adding integers – Same signs add and keep, different signs subtract – Adding positive numbers – Positive + Positive = More Positive – Adding negative numbers – Negative + Negative = More Negative – Example problems with solutions – E.g., 5 + (-3) = 2; -7 + (-2) = -9 | When adding integers, it’s crucial to understand the rules that govern how they combine. If the integers have the same sign, we add their absolute values and keep the common sign. If they have different signs, we subtract the smaller absolute value from the larger one and keep the sign of the number with the larger absolute value. Provide students with examples of adding positive numbers to reinforce the concept that the sum of two positives is a larger positive number. Similarly, demonstrate that adding two negative numbers yields a more negative result. Use example problems to illustrate these concepts, ensuring to include both straightforward and complex problems to challenge the students and solidify their understanding.

Subtracting Integers – Rules for integer subtraction – To subtract, add the opposite: a – b = a + (-b) – Subtracting positive numbers – When subtracting positives, count down the number line – Subtracting negative numbers – For negatives, subtract as if adding two positives – Worked-out example problems – Example: 5 – (-3) = 5 + 3 = 8; -7 – 4 = -7 + (-4) = -11 | This slide introduces the concept of subtracting integers, a fundamental skill in 8th-grade mathematics. Begin by explaining the rule for subtracting integers, which is to add the additive inverse of the number being subtracted. Emphasize that subtracting a positive number means moving to the left on the number line, while subtracting a negative is like adding two positive numbers. Provide clear examples to illustrate these concepts, ensuring to work through the problems step by step. Encourage students to practice with additional problems and to visualize the number line when performing these operations.

Combining Addition and Subtraction of Integers – Combine addition & subtraction rules – Follow order of operations – Apply PEMDAS to integers for correct results – Solve mixed integer problems – Use number lines or integer chips for visualization – Practice with class examples – Example: 5 + (-3) – 2 + 8 | This slide aims to integrate the rules of adding and subtracting integers and applying the order of operations to solve mixed problems. Start by reviewing the rules for addition and subtraction of integers. Emphasize the importance of the order of operations, commonly remembered by the acronym PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction), to ensure students arrive at the correct answer. Provide visual aids like number lines or integer chips to help students understand the concept of combining positive and negative values. Conclude with class examples that students can work through together, such as 5 + (-3) – 2 + 8, to solidify their understanding. Encourage students to explain their thought process during the practice to facilitate peer learning.

Class Activity: Integer Operations Relay – Divide into small groups – Solve integer addition/subtraction – Each group tackles a set of problems involving both positive and negative numbers. – Race to complete problems – The first group to finish with all correct answers wins. – Review answers collectively | This activity is designed to encourage teamwork and reinforce the concept of adding and subtracting integers. Start by dividing the class into small groups, aiming for a mix of abilities in each group. Provide each group with a set of problems that require them to add and subtract positive and negative integers. The race element adds excitement and encourages focus. Once a group believes they have completed the problems correctly, they signal they are finished. After all groups are done, review each problem as a class, discussing different methods used to find the solutions. This is also a great opportunity to address common mistakes and ensure understanding. Possible variations of the activity could include a relay format where students take turns solving problems, or a ‘scavenger hunt’ where each solved problem leads to the next.