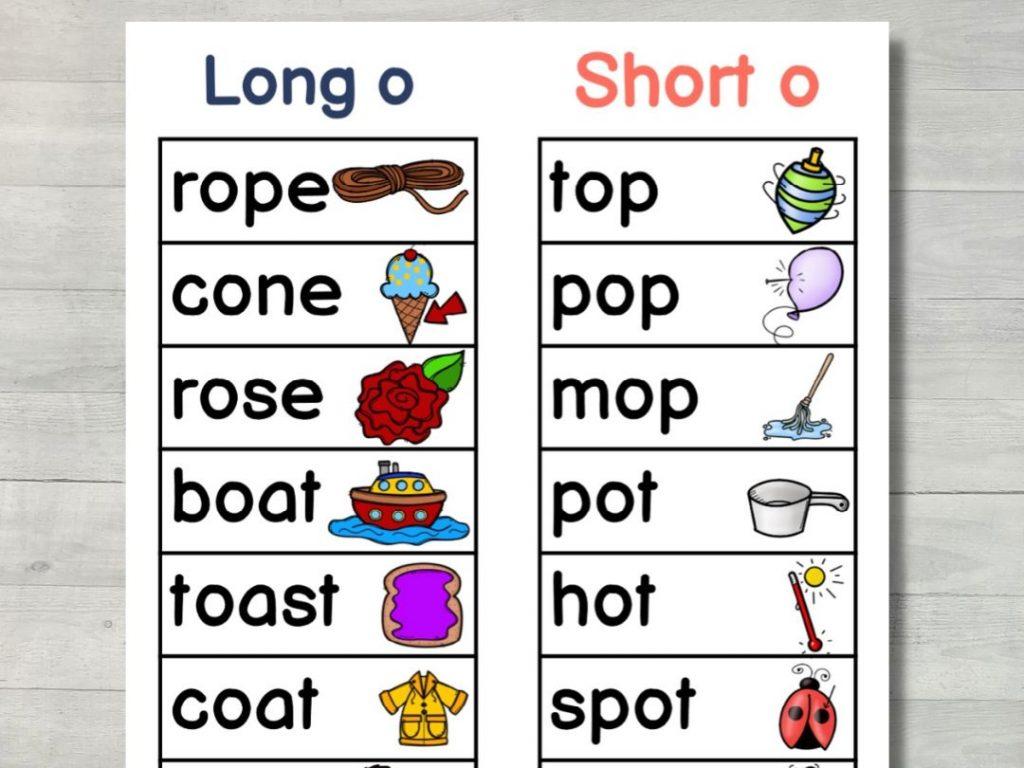
Fractions And Mixed Numbers With Denominators Of 10 And 100
Subject: Math
Grade: Fourth grade
Topic: Mixed Numbers
Please LOG IN to download the presentation. Access is available to registered users only.
View More Content
Welcome to Fractions: Denominators of 10 & 100
– Fractions represent parts of a whole
– Like a pizza cut into equal slices
– Denominators tell us the total parts
– Bottom number of a fraction, e.g., in 1/10, 10 is the denominator
– Today’s focus: Denominators 10 & 100
– We’ll explore fractions like 3/10, 7/100, and more
– Understanding 1/10 and 1/100
– 1/10 is one slice of a 10-sliced pizza; 1/100 is one penny in a dollar
|
This slide introduces the concept of fractions to fourth-grade students, focusing on those with denominators of 10 and 100. Begin by explaining that fractions are a way to represent parts of a whole, using everyday examples like slices of pizza. Clarify that the denominator is the number below the fraction line, indicating how many equal parts the whole is divided into. Emphasize the day’s lesson on fractions with denominators of 10 and 100, which are common in our decimal system and relate to dimes in a dollar and pennies in a dollar, respectively. Encourage students to think of other examples where they encounter parts of 10 and 100 in their daily lives.
Understanding Fractions
– A fraction represents a part of a whole
– Top number is the numerator
– It tells how many parts we have
– Bottom number is the denominator
– It tells how many equal parts the whole is divided into
– Examples: 1/10, 3/100, 7/10
– These are fractions with denominators 10 and 100
|
This slide introduces the concept of fractions to fourth-grade students. Begin with the definition of a fraction as a part of a whole, which can be anything like a pizza or a chocolate bar. Explain that the numerator, the top number of a fraction, indicates how many parts of the whole we’re talking about. The denominator, the bottom number, tells us into how many equal parts the whole is divided. Provide examples with denominators of 10 and 100 to align with the lesson’s focus. Use visual aids like pie charts or bar models to help students visualize fractions. Encourage students to think of their own examples of fractions they encounter in daily life.
Exploring Tenths: Fractions and Decimals
– Understanding tenths in fractions
– Tenths are fractions with denominator 10, like 1/10, 3/10, 7/10.
– How to convert tenths to decimals
– Converting is easy: 1/10 equals 0.1, 3/10 equals 0.3, and so on.
– Real-life examples of tenths
– Money is a great example: 10 cents is 1/10 of a dollar.
|
This slide introduces students to the concept of tenths in the context of fractions and their conversion to decimal form. Start by explaining that the denominator of 10 signifies that a whole is divided into 10 equal parts. Emphasize that converting tenths to decimals is straightforward because the denominator 10 relates directly to the base-10 number system used in decimals. Use relatable examples such as money to illustrate tenths, as most students are familiar with dimes and their relation to a dollar. Encourage students to think of other examples where they encounter tenths in everyday life, such as measurements on a ruler. This will help solidify their understanding of the concept.
Fractions with Denominators of 100
– Understanding hundredths
– Hundredths are parts of 100, like 1/100 or 25/100.
– Converting to decimals
– To convert, divide the numerator by 100, e.g., 25/100 = 0.25.
– Real-life examples
– Money is a great example: $0.25 is 25/100 of a dollar.
– Practice with hundredths
|
This slide introduces students to the concept of hundredths and how they relate to fractions and decimals. Start by explaining that hundredths are just like any other fraction, but with a denominator of 100. This makes them very useful for converting to decimals since 100 is a base of our number system. Use examples like money to illustrate hundredths in a context familiar to students. For instance, pennies to dollars can help them understand the concept practically. Encourage students to practice converting between fractions with denominators of 100 and decimals, and provide additional real-life examples for them to apply their knowledge.
Understanding Mixed Numbers
– Mixed numbers explained
– A number made up of a whole number and a fraction
– Whole numbers and fractions
– Identify the whole part and the fraction part in 3 1/10, 7 25/100
– Examples: denominators 10, 100
– 1 1/10, 5 1/2 (as 50/100), 2 3/100
– Practice with mixed numbers
– Use examples to add and subtract mixed numbers
|
Introduce mixed numbers as a combination of whole numbers and fractions. Emphasize that the denominator tells us the number of equal parts the whole is divided into, and in this case, it’s either 10 or 100. Provide clear examples of mixed numbers with these denominators, such as 1 1/10 and 7 25/100, and demonstrate how to identify the whole number and fractional parts. Encourage students to practice by creating their own mixed numbers and by adding and subtracting mixed numbers with like denominators. This will help solidify their understanding of the concept.
Adding and Subtracting Mixed Numbers
– Add mixed numbers with same denominators
– Stack the fractions and add the whole numbers separately
– Subtract mixed numbers with same denominators
– Keep the denominator, subtract the fractions, and the whole numbers
– Solve practice problems together
– Understand adding and subtracting rules
– Remember to simplify your answer if possible
|
This slide is aimed at teaching fourth graders how to handle mixed numbers with denominators of 10 and 100. Start by explaining that mixed numbers consist of a whole number and a fraction. When adding or subtracting mixed numbers with like denominators, we combine the whole numbers and the fractions separately. Emphasize the importance of keeping the denominators the same while performing the operations. After explaining the concepts, work through practice problems as a class to reinforce the learning. Encourage students to simplify their answers, which may include converting improper fractions back into mixed numbers. Provide several examples and ensure that students understand each step before moving on to independent practice.
Class Activity: Fraction Fun with Strips!
– Craft your own fraction strips
– Label strips for tenths and hundredths
– Each strip is a whole divided into equal parts: 10 for tenths, 100 for hundredths
– Combine strips to make mixed numbers
– A mixed number has a whole number and a fraction: like 1 and 7/10
– Explore mixed numbers with strips
|
This activity is designed to help students visualize fractions and understand mixed numbers. Provide students with materials to create fraction strips, such as colored paper or cardstock. Guide them to divide and label each strip into tenths and hundredths accurately. Once the strips are ready, demonstrate how to combine whole numbers with fraction parts to form mixed numbers. For example, combining one whole strip with a strip divided into tenths to create 1 and 7/10. Encourage students to experiment with different combinations, and facilitate a discussion on how the strips represent mixed numbers. Possible activities include: creating a mixed number wall, fraction strip matching games, or a ‘fraction strip race’ where students challenge each other to form mixed numbers faster.
Wrapping Up: Fractions & Mixed Numbers
– Recap of today’s fractions lesson
– We learned about fractions with denominators of 10 and 100.
– Time for your questions!
– Homework: Practice Worksheet
– Complete the worksheet on fractions and mixed numbers.
– Keep practicing at home!
– Try to solve problems using today’s lesson.
|
This slide is aimed at reviewing the day’s lesson on fractions and mixed numbers with denominators of 10 and 100. Start by summarizing the key points covered in the lesson, ensuring that students have a clear understanding of the concepts. Open the floor for any questions the students might have, providing clarification where needed. Introduce the homework assignment, which is a practice worksheet designed to reinforce today’s learning. Encourage students to attempt the worksheet independently, reminding them that practice is crucial for mastering the concepts. Offer support for students who may need extra help and remind them that they can bring any questions to the next class.