Your next great Lessn starts here.

Evaluating Numerical Expressions Step by Step – Today’s focus: Evaluating expressions – Understand Order of Operations – PEMDAS: Parentheses, Exponents, Multiplication/Division, Addition/Subtraction – Importance of step-by-step solving – Prevents mistakes and ensures accuracy – Practice problems – Solve sample expressions using PEMDAS | This slide introduces the concept of evaluating numerical expressions using the Order of Operations, commonly remembered by the acronym PEMDAS. Emphasize the importance of solving math problems one step at a time to avoid errors and achieve accurate results. Provide examples of numerical expressions and guide students through solving them step by step. Encourage students to practice with problems of varying difficulty to solidify their understanding. The goal is for students to become comfortable with the process and recognize the consistent logic behind the Order of Operations.

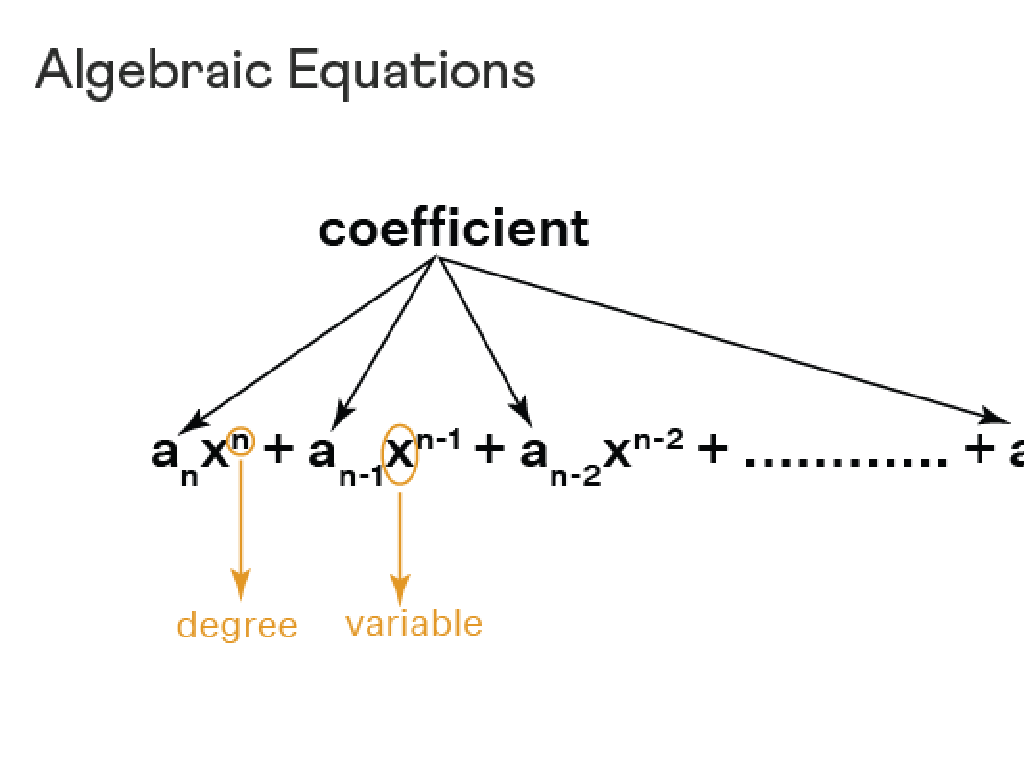
Understanding Numerical Expressions – Define numerical expressions – A mathematical phrase combining numbers and operators – Simple expression examples – For instance, 3 + 4 and 6 * 2 are simple expressions – Expression components – Terms are the numbers, coefficients multiply the terms, and operators are symbols like +, -, *, / – Practice with expressions | This slide introduces the concept of numerical expressions to sixth-grade students. Begin with the definition, ensuring students understand that numerical expressions are combinations of numbers and mathematical operators without an equals sign. Provide clear examples of simple expressions, such as addition or multiplication problems. Then, break down the parts of an expression, explaining terms, coefficients, and operators. Use visual aids or props if possible to illustrate each part. Finally, engage students with practice problems where they identify these components within different expressions, reinforcing their understanding through active participation.

Understanding Order of Operations: PEMDAS/BODMAS – What is PEMDAS/BODMAS? – A rule for the sequence to evaluate expressions: Parentheses, Exponents, Multiplication/Division, Addition/Subtraction. – Solving Parentheses/Brackets first – Brackets group parts of expressions that should be calculated first, e.g., (2 + 3) × 4. – Exponents/Orders come next – Exponents (or orders) like squares and cubes are solved after parentheses, e.g., 2^3 = 8. – Practice with examples – Let’s apply these rules to solve expressions step by step. | This slide introduces the concept of the order of operations, a fundamental principle in mathematics that dictates the sequence in which parts of a numerical expression should be evaluated. PEMDAS/BODMAS is an acronym that helps students remember this sequence: Parentheses/Brackets, Exponents/Orders, Multiplication/Division (from left to right), and Addition/Subtraction (from left to right). Emphasize the importance of solving expressions within parentheses/brackets first as they override the standard order. Then, discuss why exponents/orders are addressed before basic operations like multiplication or addition. Provide clear examples to illustrate each step, and encourage students to practice with additional problems to solidify their understanding.

Understanding Order of Operations – Multiply and divide left to right – Perform multiplication/division as they appear, moving from left to right. – Add and subtract left to right – After multiplication/division, add/subtract from left to right. – The significance of order – Correct order ensures accurate results. – Examples demonstrating order – Calculate 3 + 4 x 2 and 3 x 4 + 2 to see different outcomes. | This slide emphasizes the importance of following the correct order of operations when evaluating numerical expressions. Students should understand that multiplication and division come before addition and subtraction, but are performed in the order they appear from left to right. It’s crucial to highlight why maintaining this order is necessary, as it affects the outcome of the calculation. Provide clear examples to illustrate how different orders can lead to different results, reinforcing the concept. Encourage students to practice with additional problems to solidify their understanding.

Let’s Practice Together: Evaluating Expressions – Walk through a sample problem – Example: Evaluate 3 + 5 x 2 – Solve step by step – Start with multiplication: 5 x 2 = 10 – Discuss each step – Then add to the first number: 3 + 10 – Answer any questions – Final answer: 13 | This slide is designed for a collaborative class activity where students will practice evaluating numerical expressions using the order of operations. Begin with a sample problem, such as 3 + 5 x 2, and guide students through solving it one operation at a time. Emphasize the importance of following the correct order: multiplication before addition. After solving the multiplication, proceed with the addition to find the final answer. Encourage students to ask questions at each step to ensure understanding. Prepare to address common misconceptions and provide additional examples if needed. This activity will help solidify the concept of step-by-step evaluation of expressions.

Your Turn to Try: Evaluating Expressions – Solve practice problems individually – Explain each step of your solution – Break down the problem and justify each operation – Pair up for problem-solving – Collaborate and discuss different approaches – Check your partner’s work – Provide constructive feedback on solutions | This slide is designed to engage students in active practice of evaluating numerical expressions. Students should start by attempting the problems on their own, ensuring they understand the process of solving step by step. Encourage them to verbalize their reasoning as they solve each problem, which reinforces their understanding. Pairing up allows students to collaborate and learn from each other, offering a chance to discuss different methods and strategies. It’s also an opportunity for peer teaching, which can be very effective. The teacher should circulate the room, offering guidance and ensuring that pairs are providing each other with constructive feedback. Possible activities could include solving expressions with different operations, checking for correct order of operations, and creating their own expressions for partners to solve.

Common Mistakes in Evaluating Expressions – Remember the order of operations – Use PEMDAS: Parentheses, Exponents, Multiplication/Division, Addition/Subtraction – Keep operation signs clear – Confusing ‘+’ for ‘-‘ or ‘×’ for ‘÷’ can change the result – Simplify expressions completely – Ensure all operations are performed and the expression is in simplest form | When teaching students to evaluate numerical expressions, it’s crucial to emphasize common errors to avoid. Stress the importance of the order of operations, often remembered by the acronym PEMDAS, to solve expressions correctly. Clarify that mixing up operation signs can lead to incorrect answers and that they should double-check their work. Remind students to simplify expressions fully, leaving no operations undone. Use examples to illustrate each point and provide practice problems that specifically target these common mistakes to reinforce correct methods.

Class Activity: Expression Relay – Divide into small groups – Each group receives expressions – Solve expressions step by step – Use PEMDAS to evaluate each expression carefully – First group to finish wins | This activity is designed to encourage teamwork and reinforce the concept of evaluating numerical expressions using the order of operations. Before starting, review the PEMDAS rule with the class. Divide the class into small groups of 3-4 students. Provide each group with a set of numerical expressions to solve. Remind them to solve each expression one step at a time, following the correct order of operations. The first group to solve all their expressions correctly wins a small prize or recognition. Possible expressions to use could include: ’12 + 18 ÷ 3′, ‘5 × (6 – 2)’, ’15 – 3 × 4′, etc. Make sure to have different sets of expressions for each group to prevent copying. This activity will help students practice their problem-solving skills and understand the importance of a systematic approach to mathematics.

Wrapping Up: Order of Operations – Review today’s key points – Understand order of operations – It’s crucial for solving math problems accurately. – Complete the homework worksheet – Worksheet has problems to apply what you’ve learned. – Practice makes perfect | As we conclude today’s lesson, it’s important to recap the steps for evaluating numerical expressions. Emphasize the importance of following the order of operations (PEMDAS) to ensure accuracy in their calculations. For homework, students are assigned a worksheet with a variety of mixed operation problems to reinforce their understanding and practice the skills learned. Remind students that consistent practice is key to mastering mathematical concepts. The worksheet should be completed and ready to be discussed in the next class, providing an opportunity to address any questions or difficulties the students may have encountered.