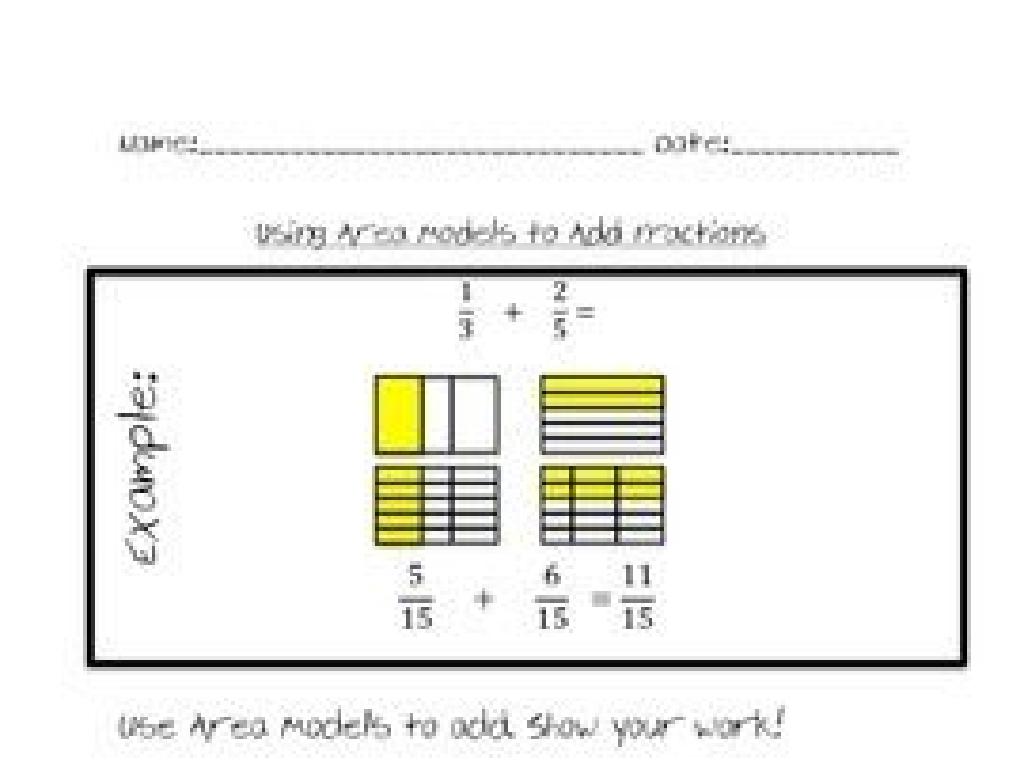
Multiply 2-Digit Numbers By 2-Digit Numbers: Choose The Area Model
Subject: Math
Grade: Fourth grade
Topic: Multiply By Two-Digit Numbers
Please LOG IN to download the presentation. Access is available to registered users only.
View More Content
Welcome to Multiplication: 2-Digit Adventure!
– Explore 2-digit multiplication
– Multiplication as area concept
– Imagine a rectangle’s length and width as numbers to multiply.
– Significance of learning multiplication
– Helps solve real-world problems, like arranging seats in rows and columns.
– Engaging multiplication activities
– Use area models to visualize and calculate products.
|
This slide introduces the concept of multiplying 2-digit numbers using the area model, which is a visual representation of multiplication as the area of a rectangle. Emphasize the importance of understanding multiplication not just as rote memorization, but as a tool for solving practical problems, such as organizing spaces or calculating areas. Encourage students to think of multiplication as building blocks to more complex math concepts. Provide examples of area models and prepare engaging activities where students can practice creating and solving their own area models with 2-digit numbers. This will help solidify their understanding and make the learning process interactive and fun.
Multiplying with the Area Model
– What is the Area Model?
– A tool to multiply using a visual rectangle shape
– Visualize multiplication as an area
– Imagine a rectangle split into parts to represent each digit
– Break down big problems
– Divide a big problem into smaller, easier pieces
– Example: Small number multiplication
– Let’s multiply 13 x 24 using the area model!
|
The Area Model is a visual method for teaching multiplication that helps students understand the concept by breaking it down into smaller, more manageable parts. Start by explaining that the area model uses the dimensions of rectangles to represent the factors in a multiplication problem. Show how to draw a rectangle and divide it into sections that correspond to the place values of the digits in the numbers being multiplied. Work through an example with smaller numbers, such as 13 x 24, by breaking it into parts (10 x 20, 10 x 4, 3 x 20, 3 x 4) and then adding the areas together to find the total product. This method reinforces the distributive property and helps students visualize the multiplication process.
Multiplying Using the Area Model
– Practice: Multiply 12 x 3
– Let’s use the area model to multiply 12 by 3.
– Arrange numbers in the area model
– Place the larger number on top and the smaller to the side.
– Calculate area for the product
– Multiply lengths of sides to find the area, which is the product.
– Understand the multiplication process
– Visualize how multiplication combines groups of numbers.
|
This slide introduces the concept of using the area model to multiply two-digit numbers. Start with a simpler example, 12 x 3, to help students grasp the method. Explain how to set up the area model by arranging the numbers, with the larger number on top and the smaller number on the side, representing the lengths of the rectangle’s sides. Then, demonstrate how to calculate the area of the rectangle, which represents the product of the multiplication. Emphasize that this visual model helps in understanding how multiplication works as combining groups of numbers. Encourage students to draw their own area models and calculate the product for additional practice.
Multiplying 2-Digit Numbers Using Area Model
– The big challenge: 34 x 76
– We’re going to multiply 34 by 76 using the area model.
– Break it down into parts
– Split 34 into 30 and 4, and 76 into 70 and 6. Multiply each pair.
– Add areas for the product
– Add the results of the four multiplications to get the final answer.
– Practice with different numbers
|
This slide introduces the concept of multiplying two 2-digit numbers using the area model, which is a visual method that breaks down the multiplication into smaller, more manageable parts. Start with a challenging example, such as 34 x 76, and demonstrate how to decompose each number into tens and ones. Then, create a grid that represents the area model, multiplying each part and filling in the corresponding sections of the grid. Finally, add all the areas together to find the final product. Encourage students to practice this method with different numbers to solidify their understanding. Provide several examples and guide them through the process step by step, ensuring they grasp the concept of area addition.
Multiplying with the Area Model
– Draw a large rectangle
– Break down both numbers
– Split into tens and ones, e.g., 23 becomes 20 and 3
– Multiply the parts separately
– Each rectangle section represents a part of the equation
– Add all parts for the total
– Combine the four products to get the final answer
|
This slide introduces the area model method for multiplying two-digit numbers, a visual way to understand multiplication. Start by drawing a large rectangle and dividing it into four parts, based on the tens and ones of each number. For example, if multiplying 23 by 47, decompose into 20 and 3, and 40 and 7. Multiply each corresponding area (20×40, 20×7, 3×40, 3×7), then add the products (800+140+120+21) to find the total (1081). This method helps students visualize the multiplication process and understand the concept of place value in calculations. Encourage students to practice with different numbers and to check their work by traditional multiplication.
Let’s Practice Together: Area Model Multiplication
– Practice Problem: 23 x 45
– Work in pairs using the area model
– Split 23 into 20 and 3, and 45 into 40 and 5. Multiply the parts to fill the area model.
– Share solutions with the class
– Discuss different methods and answers with classmates.
– Understand the area model method
– The area model helps visualize multiplication as the sum of partial products.
|
This slide is designed for a class activity where students will apply the area model to multiply two-digit numbers. The practice problem 23 x 45 is a hands-on way for students to engage with the concept. Instruct them to work in pairs to encourage collaboration and discussion, which can lead to a deeper understanding of the area model. After solving, students will share their solutions with the class, allowing them to see different approaches to the same problem. As a teacher, facilitate the activity by walking around to assist pairs as needed and ensure that each group understands the area model method. Prepare to highlight common mistakes and clarify misconceptions during the sharing session. The goal is for students to become comfortable with breaking down numbers and using the area model to solve multiplication problems.
Common Mistakes in Area Model Multiplication
– Remember to add all areas
– Keep place values straight
– Tens are 10x more than ones; don’t confuse them
– Organize work neatly
– Draw grids clearly, label each section
– Use tips to avoid errors
– Double-check work, use graph paper for alignment
|
When teaching students to multiply 2-digit numbers using the area model, it’s crucial to highlight common errors and how to avoid them. Emphasize the importance of adding all the partial products to get the final answer. Stress the significance of place value, as mixing up tens and ones can lead to incorrect results. Encourage students to keep their work organized by drawing clear grids and labeling each section, which helps in tracking the calculation process. Provide tips such as double-checking each step and using graph paper to keep numbers aligned. During the lesson, work through examples together and discuss these common pitfalls to reinforce good habits.
Class Activity: Area Model Art
– Create area model artwork
– Choose two 2-digit numbers
– Draw and color your model
Use poster paper to draw the grid, label dimensions, and color in sections
– Present your model to class
Explain how you used the area model to multiply your numbers
|
This activity is designed to help students visualize the process of multiplying two 2-digit numbers using the area model. Students will choose their own numbers, which encourages engagement and allows for differentiated learning. They will then create a visual representation of the multiplication process on poster paper. Encourage creativity in their drawings and color-coding to represent different sections of the model. As they present, students should explain the steps they took to multiply the numbers using the area model, which reinforces their understanding and communication skills. Possible variations for different students could include using different number sets, incorporating real-life applications, or challenging them to explain the model to a partner.
Review and Reflect: Multiplication with Area Models
– Recap: Area model multiplication
– We learned to multiply 2-digit numbers using the area model.
– Area models in daily life
– Imagine dividing a garden into sections to plan planting.
– Applying what we’ve learned
– Looking ahead: More multiplication
– Practice makes perfect! Try more problems at home.
|
Today’s lesson focused on understanding the area model for multiplying two-digit numbers. Reinforce the concept by summarizing the steps taken to break down the numbers into tens and ones, creating a visual representation of the problem. Discuss how this method can be applied to real-world scenarios, such as planning a garden layout or arranging furniture in a room, where area calculations are necessary. Encourage students to reflect on their learning and understand how it fits into the larger picture of mathematics. Prepare them for the next class by suggesting they practice with additional problems, ensuring they’re ready to build on this foundation with more complex multiplication challenges.