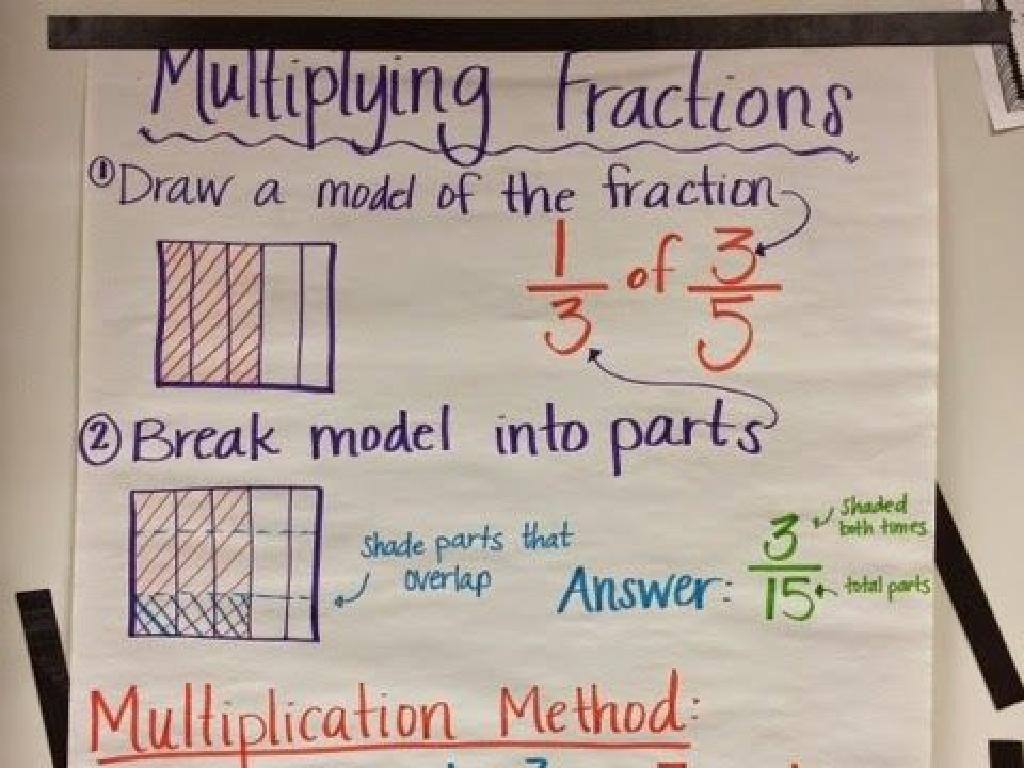
Multiply Mixed Numbers And Whole Numbers
Subject: Math
Grade: Sixth grade
Topic: Multiply Fractions
Please LOG IN to download the presentation. Access is available to registered users only.
View More Content
Multiplying Mixed Numbers with Whole Numbers
– Quick review of fractions
– Fractions represent parts of a whole
– Mixed numbers introduction
– Mixed numbers have a whole number and a fraction
– Multiplying mixed numbers by whole numbers
– Convert to improper fraction, then multiply
– Practical examples
– Apply what we’ve learned to solve real-world problems
|
Begin with a brief review of fractions to ensure students recall how to identify and work with them. Introduce mixed numbers as a combination of a whole number and a fraction. Explain the process of converting mixed numbers to improper fractions for easier multiplication with whole numbers. Provide practical examples, such as multiplying recipes or cutting wood, to demonstrate the application of this concept. Encourage students to visualize the process to better understand the multiplication of mixed numbers with whole numbers.
Understanding Mixed Numbers
– Define a mixed number
– A number with a whole part and a fraction part, e.g., 2 1/2
– Examples of mixed numbers
– For instance, 3 3/4 (pizza slices), 5 1/2 (hours of sleep)
– Convert to improper fractions
– Multiply the whole number by the denominator, add the numerator
– Practice conversion
– Let’s turn 4 2/3 into an improper fraction together
|
Begin by defining a mixed number as a combination of a whole number and a fraction. Provide relatable examples such as pieces of pizza or hours of sleep to help students visualize mixed numbers. Explain the process of converting mixed numbers to improper fractions by using the formula: (whole number × denominator) + numerator, all over the original denominator. Work through an example as a class, such as converting 4 2/3 into an improper fraction, to demonstrate the process. Encourage students to practice this conversion with several examples to ensure understanding.
Multiplication Review: Mixed Numbers
– Review multiplication facts
– Quick refresher of times tables
– Multiplication in daily life
– Discuss how we use multiplication for tasks like cooking or shopping
– Multiply whole numbers
– Understand multiplying larger whole numbers
– Practice with examples
|
Begin the lesson with a quick review of multiplication facts to ensure students are comfortable with basic times tables. Highlight the importance of multiplication in everyday scenarios, such as calculating total cost while shopping or doubling a recipe while cooking. Then, focus on the process of multiplying whole numbers, ensuring students understand the concept of adding a number to itself a certain number of times. Provide examples for the students to solve, reinforcing the lesson and preparing them for multiplying mixed numbers and whole numbers. Encourage students to ask questions and work through problems together.
Multiplying Whole Numbers by Fractions
– Understand the multiplication process
– Multiplication as repeated addition
– Follow a step-by-step example
– Example: 3 x (4/5) = ? Convert whole number to fraction (3/1), then multiply numerators and denominators.
– Comprehend why the method works
– Relate to area models and visual representation to show equal parts of a whole being combined.
|
This slide aims to break down the concept of multiplying whole numbers by fractions for sixth-grade students. Begin by explaining multiplication as repeated addition, which they are familiar with, and then transition to how it applies to fractions. Provide a clear, step-by-step example, such as multiplying 3 by 4/5, showing the conversion of the whole number to a fraction (3/1) and then multiplying across for numerators and denominators. Emphasize understanding the underlying concept by relating it to visual models, such as shaded areas representing fractions of a whole, to illustrate why the method works. This will help students grasp the concept and apply it to various problems.
Multiplying Mixed Numbers by Whole Numbers
– Convert mixed numbers to improper fractions
– Change a mixed number like 2 1/3 to an improper fraction, 7/3
– Multiply the improper fraction by the whole number
– Multiply 7/3 (improper fraction) by a whole number, e.g., 7/3 * 4
– Convert the result to a mixed number
– Change the product, e.g., 28/3, back to a mixed number, 9 1/3
– Simplify the final answer if needed
– If the fraction part can be simplified, do it to get the simplest form
|
This slide is aimed at teaching students the process of multiplying mixed numbers with whole numbers. Start by converting mixed numbers into improper fractions to simplify the multiplication process. Once converted, multiply the improper fraction by the whole number just as you would with a regular fraction. After obtaining the product, convert it back to a mixed number if the numerator is larger than the denominator. Lastly, ensure the final answer is in its simplest form by simplifying the fraction part of the mixed number. Provide examples for each step and encourage students to practice with different sets of numbers to gain confidence.
Let’s Practice Together: Multiplying Mixed Numbers
– Example 1: 2 1/3 x 4
– Convert to improper fraction: 7/3 x 4
– Example 2: 5 x 7 1/2
– Convert 7 1/2 to 15/2, then multiply: 5 x 15/2
– Group Activity: Partner Problem Solving
– Work with a partner to solve similar problems
|
This slide is designed for a collaborative classroom activity where students practice multiplying mixed numbers with whole numbers. Begin by walking through the first example, converting the mixed number to an improper fraction (2 1/3 to 7/3) and then multiplying by the whole number (4). Repeat the process with the second example, converting 7 1/2 to 15/2 before multiplying by 5. For the group activity, students should pair up and tackle a set of practice problems together, allowing them to discuss strategies and reinforce their understanding. Provide guidance on converting mixed numbers and multiplying fractions, and encourage students to check each other’s work. Possible activities could include creating a poster of their solutions, explaining their problem-solving process to the class, or creating their own mixed number multiplication problems for others to solve.
Common Mistakes in Multiplying Mixed Numbers
– Convert mixed numbers to improper fractions
e.g., Convert 2 1/3 to 7/3 before multiplying
– Simplify fractions before multiplying
Reduce fractions to simplest form to make multiplication easier
– Convert back to mixed numbers after multiplying
After multiplying, change improper fractions like 15/4 to mixed numbers, e.g., 3 3/4
– Practice with examples to avoid errors
|
When teaching multiplication of mixed numbers, it’s crucial to emphasize the importance of converting mixed numbers to improper fractions before multiplying. This avoids confusion and simplifies the calculation process. Students should also be reminded to simplify fractions before multiplying to make the math less complex. After finding the product, it’s important to convert any improper fractions back to mixed numbers for the final answer. Provide practice problems that reinforce these steps and correct common mistakes. Encourage students to double-check their work for these errors.
Class Activity: Fraction Multiplication Challenge
– Work in groups on multiplication
– Use fraction strips for visualization
– Fraction strips help see the fractions visually and understand the multiplication process
– Present solutions to the class
– Explain your reasoning
– Discuss how you got the answer and why your method works
|
This activity is designed to promote collaborative learning and a deeper understanding of multiplying mixed numbers and whole numbers. Divide the class into small groups and provide them with a set of multiplication problems involving mixed numbers and whole numbers. Hand out fraction strips to each group to aid in visualizing the multiplication process. After solving the problems, each group will present their solutions to the class, explaining the steps they took and the reasoning behind their methods. This will help students articulate their thought process and solidify their understanding. As a teacher, facilitate the activity by ensuring each group understands the task, offer guidance if they struggle with the concept, and encourage clear and thorough explanations during presentations. Possible activities could include multiplying different sets of mixed numbers and whole numbers, comparing results with traditional multiplication methods, and discussing common mistakes to watch out for.
Wrapping Up: Multiplication Mastery
– Review of mixed number multiplication
– Practice makes perfect
– Consistent practice is key to understanding math concepts.
– Homework: Multiplication worksheet
– Complete the provided worksheet to reinforce today’s lesson.
– Keep practicing at home!
|
As we conclude today’s lesson on multiplying mixed numbers with whole numbers, it’s crucial to emphasize the importance of practice in mastering this skill. The homework assignment is a worksheet that includes a variety of problems to help students solidify their understanding of the multiplication process. Encourage students to attempt each problem and to review the steps we’ve learned if they encounter difficulties. Remind them that making mistakes is a part of learning and that each problem they solve correctly will build their confidence and proficiency in math.