Your next great Lessn starts here.

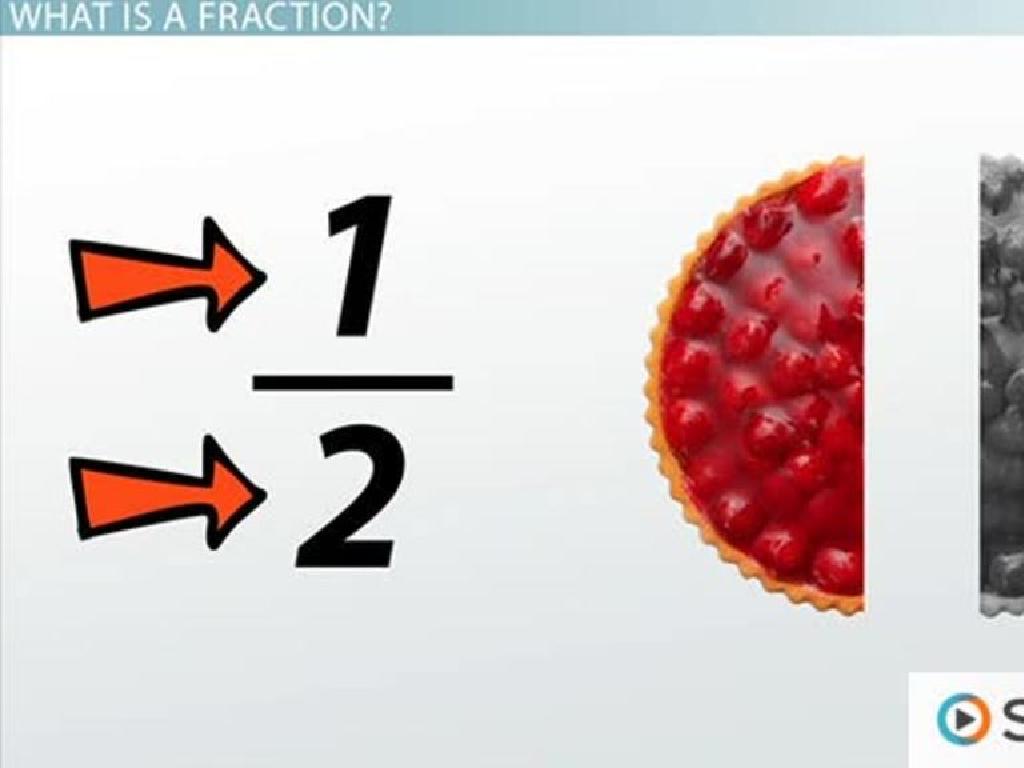
Introduction to Fractions – Fractions represent parts of a whole – Like a pizza sliced into 4 pieces, each piece is a fraction of the pizza – Fractions are everywhere in daily life – Examples: 1/2 cup of sugar, 1/4 of an hour – Review: Numerator over Denominator – Numerator (top number) shows how many parts we have, denominator (bottom number) shows how many equal parts the whole is divided into – Understanding equals dividing a number | This slide introduces the concept of fractions to fourth graders by relating it to familiar items, such as slices of pizza, to illustrate parts of a whole. It’s important to use everyday examples to help students see the relevance of fractions in their daily lives. A quick review of the terms numerator and denominator will reinforce their understanding of fractions. Emphasize that the numerator indicates the number of parts being considered, while the denominator indicates the total number of equal parts that make up a whole. This foundational knowledge will be crucial as they learn to model and multiply fractions.

Fractions of a Number: Model and Multiply – Understanding fractions of a number – Finding a fraction of a number means multiplying the fraction by the number. – Visualizing with models – Draw a shape, divide it into equal parts, shade the fraction you need. – Example: 1/2 of 8 – 1/2 of 8 equals 4 because 1/2 times 8 equals 4. – Multiplying to find fractions – Use multiplication to calculate the fraction of any number. | This slide introduces the concept of finding a fraction of a number, which is a foundational skill in understanding fractions. Start by explaining that to find a fraction of a number, we multiply the fraction by the number. Use visual aids like pie charts or bar models to help students visualize the concept. For example, to find 1/2 of 8, we can draw a bar divided into 8 equal parts and shade 4 to represent 1/2. This visual representation reinforces the multiplication of 1/2 by 8 to get 4. Encourage students to practice with different fractions and numbers to solidify their understanding.

Multiplying Fractions by Whole Numbers – Multiplication with fractions – It’s like adding the fraction to itself multiple times. – Steps to multiply fractions – 1) Multiply the whole number by the numerator. 2) Keep the same denominator. – Example: Multiply 3 by 2/4 – 3 x 2/4 equals 6/4 or 1 1/2 | When we multiply a whole number by a fraction, we are essentially adding the fraction to itself as many times as the whole number indicates. It’s important to walk through the process step by step: first, multiply the whole number by the numerator of the fraction, then place the product over the original denominator. For example, multiplying 3 by 2/4, we multiply 3 (the whole number) by 2 (the numerator) to get 6, and then we place the 6 over the original denominator, 4, resulting in 6/4, which simplifies to 1 1/2. This slide will help students visualize the process and understand the concept of multiplying fractions by whole numbers.

Visualizing Fraction Multiplication – Use bar models for multiplication – Bar models show parts of a whole. Multiply 1/2 by 4 by shading 4 halves. – Interactive class example – Let’s multiply 2/3 by 3 together using a bar divided into thirds. – Discuss model benefits – How do bar models make multiplication clearer? – Understanding multiplication visually | This slide aims to help students visualize the process of multiplying fractions with whole numbers using bar models. Begin by explaining that bar models represent fractions as parts of a whole, making it easier to see the multiplication process. Conduct an interactive example where the class works through a multiplication problem together, such as 2/3 x 3, using a bar model. Engage the class in a discussion about how the model aids their understanding, emphasizing that visual models can make abstract concepts more concrete. Encourage students to think of other situations where visual models could be helpful in math.

Fraction Multiplication Practice – Find 1/4 of 12 – 1/4 of 12 equals 3. (12 divided by 4) – Multiply 5 by 3/8 – 5 times 3/8 equals 15/8 or 1 7/8. | This slide is designed for a class activity where students will practice multiplying fractions with whole numbers. For problem 1, guide the students to understand that finding 1/4 of 12 is the same as dividing 12 by 4. For problem 2, demonstrate how to multiply a whole number by a fraction by multiplying the numerator (5 x 3) and keeping the denominator the same (8). Then, simplify the fraction if possible. Encourage students to visualize these problems with models or drawings. Possible activities include using fraction circles, drawing bar models, or creating real-life scenarios where these calculations would be necessary.

Class Activity: Fraction Multiplication Art – Create fraction-based art – Multiply to find colored squares – If 1/2 of your art is blue and you have 20 squares, how many do you color blue? – Share art and explain process – Understand fractions through creativity – This activity combines art with math to visualize fraction multiplication. | This activity is designed to help students understand the concept of multiplying fractions by whole numbers through a creative and engaging art project. Students will create their own artwork on a grid, using colors to represent different fractions. They will then use multiplication to determine how many squares of each color they should have. For example, if 1/2 of their art is blue and the total number of squares is 20, they need to calculate 1/2 of 20 to find out how many squares to color blue. After completing their artwork, students will share their creations with the class and explain the multiplication process they used. This will reinforce their understanding and allow them to see how fractions are used in a real-world context. Possible variations of the activity could include using different fractions or a larger grid for more complexity.

Wrapping Up: Fractions of a Number – Recap of fraction multiplication – Remember how to model and multiply fractions with whole numbers. – Significance of learning fractions – Fractions are everywhere: cooking, money, time! – Homework: Multiplication worksheet – Complete the worksheet to become a fractions expert. – Keep practicing at home! | As we conclude today’s lesson, it’s important to review the key concepts of multiplying fractions with whole numbers. Emphasize the real-world applications of fractions, such as in recipes or budgeting, to illustrate why understanding fractions is crucial. For homework, assign a practice worksheet that reinforces today’s lesson and encourages repetition for mastery. Remind students that consistent practice is key to becoming confident in working with fractions. During the next class, be prepared to review the homework answers and address any questions or difficulties the students may have encountered.