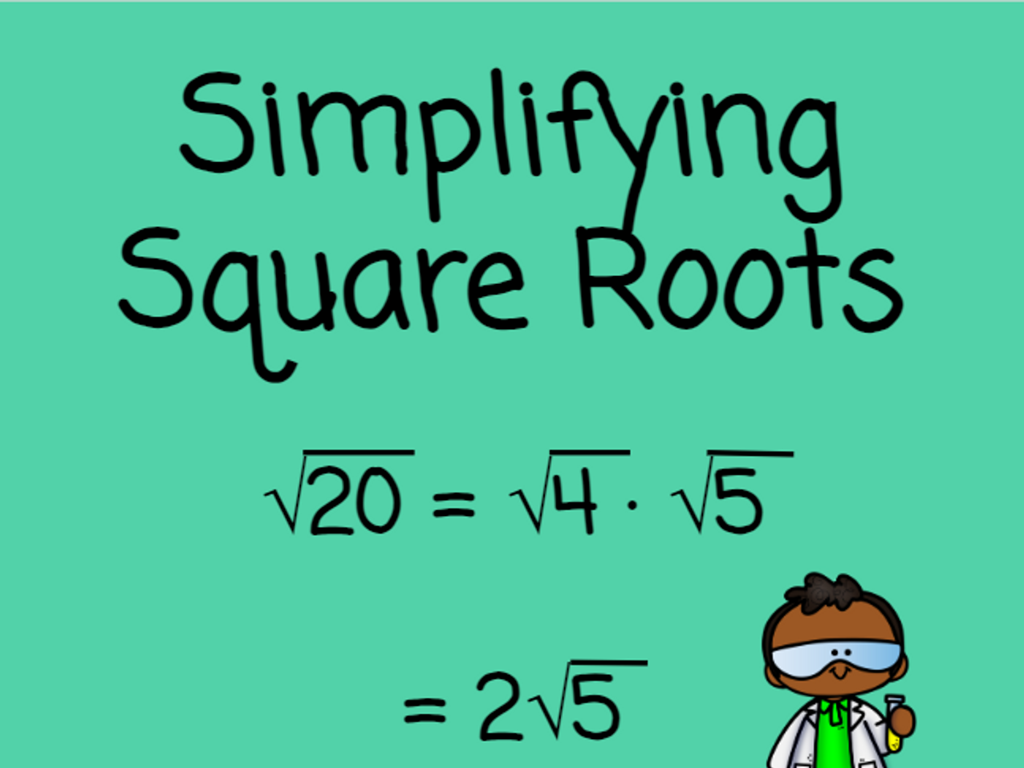Your next great Lessn starts here.

Multiplying Mixed Numbers by a Fraction – What is a mixed number? A mixed number has a whole number and a fraction, like 2 1/2. – Exploring fractions A fraction represents a part of a whole, like 1/4 of a pizza. – Multiplying mixed numbers Convert mixed numbers to improper fractions, then multiply. – Practice problems Let’s solve 2 1/2 x 1/4 together as an example. | Begin the lesson by explaining what mixed numbers are, using examples that include both whole numbers and fractions. Then, move on to a brief review of fractions, ensuring students understand how they represent parts of a whole. Next, introduce the concept of multiplying a mixed number by a fraction, emphasizing the need to convert mixed numbers to improper fractions before multiplying. Provide a step-by-step guide on how to perform the multiplication. Conclude with practice problems, encouraging students to try converting and multiplying on their own, and then review the solutions together in class.

Review: Multiplying Fractions – Recap simple fraction multiplication – Example: Multiply 1/2 by 3/4 – 1/2 x 3/4 = 1/2 of 3/4 or 3/8 – Visualize fraction multiplication – Use area models or number lines to show how parts of a whole are multiplied – Understand multiplication concept – Multiplying fractions combines parts of two wholes | Begin with a quick review of how to multiply simple fractions, ensuring students recall the process of multiplying numerators and denominators. Use the example 1/2 x 3/4 to illustrate this concept, showing that multiplying these fractions gives us a part of a part, which is 3/8 in this case. Introduce visual aids like area models or number lines to help students visualize the concept of fraction multiplication. Emphasize that when we multiply fractions, we are combining parts of two different wholes to find a new part of a whole. This foundational understanding will be crucial as they progress to multiplying mixed numbers by fractions.

Understanding Mixed Numbers – Definition of mixed numbers – A mixed number has a whole part and a fraction part – Identifying parts of mixed numbers – Whole number is separate, fraction is part of a whole – Examples of mixed numbers – 2 1/3, 4 1/2, and 5 3/4 are mixed numbers – Multiplying with fractions – To multiply, convert to improper fractions first | Begin by explaining that a mixed number is made up of a whole number and a fraction. Emphasize the distinction between the two parts: the whole number represents complete units, while the fraction represents a part of a unit. Provide clear examples of mixed numbers, using visual aids if possible, to help students identify the whole number and fraction parts. Then, guide students through the process of converting mixed numbers to improper fractions, which is necessary for multiplication with other fractions. Use simple, relatable examples to demonstrate this process and prepare students for practice problems where they will apply this knowledge.

Converting Mixed Numbers to Improper Fractions – Why convert for multiplication? – Steps to convert: Multiply, then Add – Multiply the whole number by the denominator, add the numerator, write as a fraction – Let’s practice conversion – Practice with 1 2/5 or 3 3/4 – Example: 2 1/3 becomes 7/3 – 2 x 3 + 1 = 7, so 2 1/3 is 7/3 | When multiplying a mixed number by a fraction, it’s easier to convert the mixed number to an improper fraction first. This slide explains the necessity of conversion for multiplication and outlines the method: multiply the whole number by the denominator, add the numerator, and write the result over the original denominator. Provide practice examples for the students to work through, such as converting 1 2/5 or 3 3/4 to improper fractions. Use the example of 2 1/3, where 2 (the whole number) is multiplied by 3 (the denominator), and 1 (the numerator) is added to get 7, making the improper fraction 7/3. Encourage students to try more examples and ensure they understand each step before moving on to multiplication.

Multiplying a Mixed Number by a Fraction – Convert mixed to improper fraction – Example: 2 1/3 becomes 7/3 – Multiply improper fraction by fraction – Multiply numerators and denominators – Simplify the resulting fraction – Reduce the fraction to simplest form | When teaching fifth graders to multiply a mixed number by a fraction, start by converting the mixed number into an improper fraction. This involves multiplying the whole number by the denominator of the fraction part and adding the numerator. Once converted, multiply the numerators of the improper fraction and the fraction together and do the same with the denominators. After multiplication, simplify the fraction by finding the greatest common divisor and dividing both the numerator and denominator by it. Use examples like 2 1/3 x 1/4 to illustrate the process. Simplifying may not always be necessary if the fraction is already in its simplest form. Encourage students to practice with different mixed numbers and fractions to gain confidence.

Multiplying Mixed Numbers by Fractions – Convert mixed number to improper fraction – 2 1/3 becomes 7/3 – Multiply the improper fraction by the fraction – Multiply 7/3 by 4/5 to get 28/15 – Simplify the resulting fraction – Simplify 28/15 to 1 13/15 – Example: 2 1/3 x 4/5 | Begin by explaining the concept of mixed numbers and improper fractions. Show students how to convert a mixed number into an improper fraction by multiplying the whole number by the denominator and adding the numerator. Next, demonstrate the multiplication of the improper fraction by the other fraction. Emphasize the importance of multiplying numerators with numerators and denominators with denominators. After obtaining the result, guide students through the simplification process to convert the fraction back to a mixed number if necessary. Use the example 2 1/3 x 4/5 to illustrate these steps, ensuring to work through the problem step by step. Encourage students to practice with additional examples.

Multiplying Mixed Numbers by Fractions: Practice – Solve 1 2/5 x 3/8 together – Convert to improper fraction: 7/5 x 3/8 – Solve 3 3/4 x 2/3 together – Convert to improper fraction: 15/4 x 2/3 | This slide is designed for a collaborative classroom activity where students practice multiplying mixed numbers by fractions. Start by guiding students through the process of converting mixed numbers into improper fractions. For example, 1 2/5 becomes 7/5 and 3 3/4 becomes 15/4. Then, demonstrate how to multiply these improper fractions by the given fraction. Encourage students to simplify their answers if possible. Provide step-by-step guidance and allow students to work through the problems, either individually or in small groups. Afterward, discuss the solutions as a class to ensure understanding. Possible activities include peer teaching, using manipulatives for visual learners, or creating a real-life scenario where this math application would be necessary.

Class Activity: Multiplication Relay – Split class into teams – Each member solves a step – Work through mixed number multiplication – Example: 2 1/3 x 4/5, break down into steps like converting mixed number to improper fraction – First team to finish wins! | This activity is designed to encourage teamwork and reinforce the concept of multiplying mixed numbers by fractions. Divide the class into small groups, and give each team a multiplication problem involving a mixed number and a fraction. Each student in the team is responsible for one step of the problem, such as converting the mixed number to an improper fraction, multiplying the numerators, multiplying the denominators, and simplifying the result if possible. The first team to complete all steps correctly wins a small prize. This activity helps students practice the multiplication process and understand the importance of each step. It also adds a fun competitive element to the learning process. Possible variations of the activity could include different levels of difficulty in the problems or a relay race where students physically move to a station to complete their step.

Conclusion & Homework: Mixed Numbers – Excellent work on mixed numbers! – Homework: Finish the worksheet – Worksheet has problems to reinforce today’s lesson – Remember the steps: convert, multiply, simplify – Convert mixed numbers to improper fractions, then multiply, and reduce the result – Practice makes perfect! | Students have learned how to multiply mixed numbers by fractions in today’s lesson. For homework, they are assigned a worksheet that contains problems similar to those discussed in class. This will help reinforce their understanding. Remind them of the steps: converting mixed numbers to improper fractions, multiplying, and then simplifying the results. Encourage them to practice these steps to become more confident in solving multiplication problems involving mixed numbers and fractions. In the next class, review the homework to address any difficulties they may have encountered.