Your next great Lessn starts here.

Multiplying Mixed Numbers Adventure – Welcome to today’s math journey – Objective: Multiply mixed numbers – Learn to multiply fractions, whole numbers, and mixed numbers – Real-life math: Cooking, building, shopping – Use math in recipes, construction, and budgeting – Practice makes perfect | Begin the class with a warm welcome, setting an adventurous tone for learning how to multiply mixed numbers. The objective is to ensure students understand and can apply the multiplication of fractions, whole numbers, and mixed numbers. Highlight the importance of this skill in everyday activities such as cooking (doubling a recipe), building (calculating materials needed), and shopping (figuring out discounts and quantities). Encourage students to see the value of math beyond the classroom and emphasize the importance of practice through class activities and homework.

Understanding Mixed Numbers – What are mixed numbers? – A whole number and a fraction combined, like 2 1/2 – Everyday examples of mixed numbers – Examples: 1 3/4 hours of play, 2 1/2 cups of flour – Converting to improper fractions – Multiply the whole number by the denominator, add the numerator – Practice with real-life problems – Use these concepts to solve problems involving recipes or time | This slide introduces students to mixed numbers, which are numbers made up of a whole number and a fraction. Provide clear examples from daily life to help students relate to the concept. Review the steps to convert mixed numbers to improper fractions, as this is a crucial skill for multiplying mixed numbers. Emphasize the importance of understanding these concepts by connecting them to real-world situations like cooking or scheduling activities. Encourage students to practice these conversions as homework to reinforce their learning.

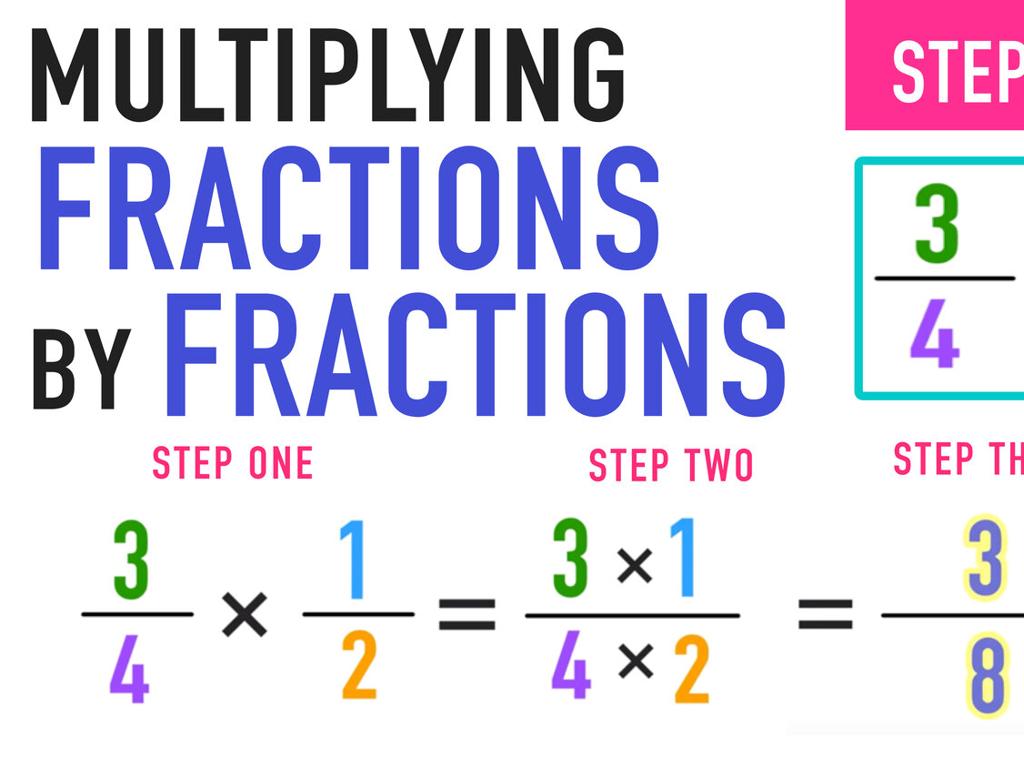
Multiplication Review: Mixed Numbers – Recap multiplying whole numbers – Review: 3 x 4 = 12. Multiplication is repeated addition. – Recap multiplying fractions – Fractions: 1/2 x 3/4 = 3/8. Multiply numerators and denominators. – Simplify fractions importance – Simplifying makes fractions easier to work with. – Steps to multiply mixed numbers – Convert to improper fractions, multiply, then simplify. | Begin with a review of basic multiplication, emphasizing that it is repeated addition, which they are familiar with. Then, transition to multiplying fractions, ensuring to clarify that they multiply the numerators together and the denominators together. Stress the importance of simplifying fractions to make them easier to understand and use in further calculations. Finally, introduce the steps to multiply mixed numbers: converting them to improper fractions, performing the multiplication, and then simplifying the result. Provide examples for each step and encourage students to practice these steps with different numbers.

Step-by-Step: Multiplying Mixed Numbers – Convert to improper fractions – Change mixed numbers like 2 1/3 to improper fractions like 7/3 – Multiply the numerators – Multiply the top numbers of the fractions – Multiply the denominators – Multiply the bottom numbers of the fractions – Convert back to mixed numbers – If the answer is an improper fraction, change it to a mixed number – Simplify the final answer – Make the fraction as simple as possible | Begin by explaining the concept of mixed numbers and improper fractions. Demonstrate how to convert a mixed number to an improper fraction by multiplying the whole number by the denominator and adding the numerator. Next, show how to multiply the numerators of the fractions to get a new numerator and do the same with the denominators for a new denominator. If the result is an improper fraction, teach students how to convert it back to a mixed number by dividing the numerator by the denominator. Lastly, guide students on how to simplify their answer by finding the greatest common divisor and dividing both the numerator and denominator by it. Provide examples and practice problems to reinforce the concept.

Multiplying Mixed Numbers: Example Problem – Convert mixed to improper fractions – 2 1/3 becomes 7/3, 1 1/2 becomes 3/2 – Multiply numerators and denominators – Multiply tops (7x3x3), Multiply bottoms (3x2x1) – Change back to a mixed number – Improper fraction to mixed number – Simplify the final result – Ensure the fraction is in its simplest form | This slide presents a structured approach to multiplying mixed numbers by converting them to improper fractions, multiplying, and then converting back. Start by explaining the conversion process (2 1/3 to 7/3 and 1 1/2 to 3/2). Then, guide students through the multiplication of numerators (7x3x3) and denominators (3x2x1). Next, demonstrate how to convert the resulting improper fraction back into a mixed number. Finally, show how to simplify the fraction, if possible. Encourage students to practice these steps with different numbers to gain confidence in the process.

Multiplying Mixed Numbers: Example Problem – Multiply 4 1/4, 2, and 5 2/5 – Convert to improper fractions: 17/4 * 2/1 * 27/5 – Follow the step-by-step guide – Multiply numerators, then denominators: (17*2*27)/(4*1*5) – Predict the answer first – What do you think the product will be? – Solve the problem together – We’ll multiply step by step and check our prediction | This slide presents an example problem to help students practice multiplying mixed numbers, whole numbers, and fractions. Start by converting all mixed numbers to improper fractions. Then, multiply the numerators across to get the new numerator and the denominators across to get the new denominator. Before solving, ask students to make a prediction to engage them in the process. Work through the multiplication step by step, ensuring to simplify the final answer. This exercise will reinforce their understanding of multiplication and provide practice with mixed numbers and fractions.

Practice Time: Multiplying Mixed Numbers – Work on practice problems – Use visual aids for help – Draw pies or number lines to visualize fractions – Teacher support during practice – Share solutions with the class – Discuss different methods used | This slide is designed for a hands-on practice session where students will apply what they’ve learned about multiplying mixed numbers, fractions, and whole numbers. Provide a variety of problems for individual or group work to cater to different learning paces. Encourage the use of visual aids like fraction pies or number lines to help students better understand the multiplication process. Teachers should move around the classroom to offer guidance and answer questions. Conclude the activity with a discussion where students can share their solutions and the strategies they used, fostering a collaborative learning environment.

Class Activity: Recipe Challenge – Double a recipe using multiplication – Work in groups for new measurements – Collaborate to adjust the recipe accurately – Present your findings to the class – Apply multiplication of mixed numbers – Use skills in multiplying fractions and whole numbers | This activity is designed to help students apply their knowledge of multiplying mixed numbers, fractions, and whole numbers in a practical context. Divide the class into small groups and provide each with a simple recipe. Their task is to double the recipe by calculating the new measurements for each ingredient, which will require them to multiply mixed numbers. This hands-on activity not only reinforces mathematical concepts but also promotes teamwork. As a teacher, circulate the room to assist groups as needed. After calculations, each group will present their doubled recipe to the class, explaining how they arrived at their measurements. Possible variations for different groups could include tripling a recipe or adjusting it by a different factor to cater to diverse learning levels.

Wrapping Up: Multiplication Mastery – Review of multiplying mixed numbers – Homework: Apply what you’ve learned – Solve problems involving mixed numbers – Real-life examples for practice – Use scenarios like cooking or building – Remember: Consistent practice is key | As we conclude today’s lesson on multiplying mixed numbers, fractions, and whole numbers, it’s important to recap the steps we’ve learned. For homework, students should practice with problems that apply these concepts to real-life situations, such as doubling a recipe or calculating the area of a garden. This will help solidify their understanding and show them the practical applications of what they’ve learned. Encourage students to practice regularly, as this will greatly improve their proficiency with multiplication of mixed numbers. In the next class, we can review any challenging problems and celebrate their progress.