Your next great Lessn starts here.

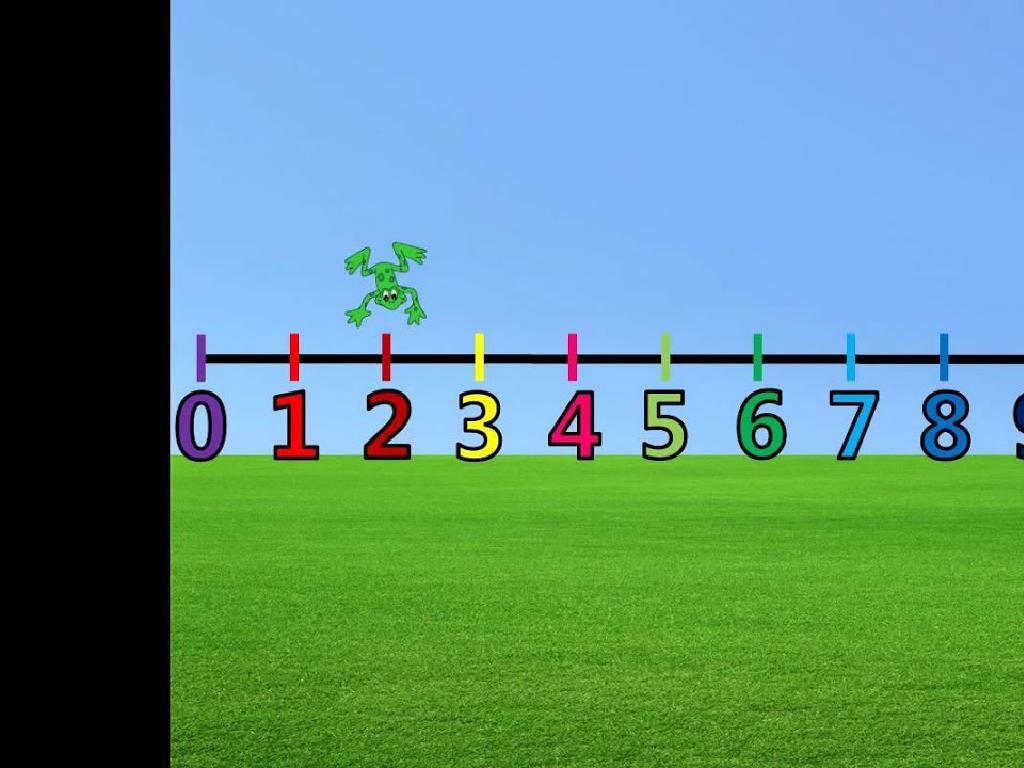
Multiplying Unit Fractions by Whole Numbers – What is a unit fraction? – A unit fraction has a numerator of 1, like 1/2 or 1/3. – Multiplication as repeated addition – Multiplying is like adding a number over and over. – Visualizing on a number line – Each jump on the line represents adding the fraction. – Practice with examples – Let’s try 1/3 x 4 on the number line together! | This slide introduces the concept of multiplying unit fractions by whole numbers using a number line. Begin by explaining that a unit fraction is a fraction with a numerator of 1. Emphasize that when we multiply a fraction by a whole number, we are essentially adding the fraction to itself that many times. Use the number line to visually demonstrate this concept by making equal jumps to represent each addition of the unit fraction. Provide clear examples, such as multiplying 1/3 by 4, and show how each jump on the number line adds another 1/3. Encourage students to draw their own number lines and practice with different unit fractions and whole numbers to solidify their understanding.

Understanding Unit Fractions – Definition of a unit fraction – A fraction with 1 as its top number – Examples of unit fractions – Like 1/2 (half), 1/3 (third), 1/4 (quarter) – Unit fractions build all fractions – Every fraction is a sum of unit fractions – Visualizing with number lines – Use number lines to multiply unit fractions | Introduce the concept of unit fractions by explaining that they have a numerator of 1. Provide examples such as 1/2, 1/3, 1/4, and so on, to illustrate the concept. Emphasize that all other fractions are composed of unit fractions, which helps in understanding how to multiply fractions by whole numbers. Demonstrate how to use number lines to visually represent the multiplication of unit fractions by whole numbers, which will be covered in more detail in subsequent slides. This foundational knowledge is crucial for students to grasp before moving on to more complex fraction multiplication.

Multiplying Whole Numbers by Unit Fractions – Understanding ‘parts of a whole’ – Example: 3 x 1/4 explained – 3 x 1/4 means 3 parts of 1 whole divided into 4 – Using number lines for multiplication – Visualize multiplication as jumps on a line – Practice with different fractions – Try 2 x 1/3 or 4 x 1/5 to strengthen understanding | This slide introduces the concept of multiplying whole numbers by unit fractions, emphasizing the idea of finding ‘parts of a whole’. Use the example 3 x 1/4 to show how to find 3 parts when a whole is divided into 4 equal parts. Explain that each part is 1/4, so 3 parts would be 3/4. Introduce the number line as a tool to visualize this multiplication by making equal jumps to represent each part. Encourage students to practice with different fractions to solidify their understanding. Provide guidance on how to draw and use number lines for this purpose. The goal is for students to feel comfortable with the concept and to apply it to various problems.

Multiplying Unit Fractions Using Number Lines – Visualize multiplication with number lines – ‘Hop’ in unit fraction increments – Imagine jumping forward on the number line by the fraction amount each time – Example: Multiply 1/4 by 3 – Start at 0, make 3 hops of 1/4 each to land on 3/4 – Practice with different fractions – Try multiplying 1/5 by 4 or 1/3 by 2 on a number line | This slide introduces the concept of using number lines to multiply unit fractions by whole numbers, which is a visual and intuitive method for fourth graders. Begin by explaining how number lines represent numbers and how we can use them to understand multiplication as repeated addition. Demonstrate with an example, such as multiplying 1/4 by 3, by showing how to make three ‘hops’ of 1/4 on the number line to reach the product of 3/4. Encourage students to practice with different fractions and whole numbers to solidify their understanding. Provide several number lines for students to use during practice and ensure they are comfortable with the ‘hopping’ concept.

Let’s Practice Together: Multiplying Fractions on a Number Line – Example: 1/3 x 4 on a number line – Make 4 ‘hops’ of 1/3 each – Starting at 0, jump to 1/3, 2/3, 3/3 (which is 1), and finally 4/3 – Observe where we land after the hops – We land at 4/3, which is also 1 1/3 – Discuss the result of the multiplication – Multiplying 1/3 by 4 gives us 4/3 or 1 1/3 | This slide is an interactive class activity to help students understand how to multiply unit fractions by whole numbers using a number line. Begin by explaining the example of multiplying 1/3 by 4. Demonstrate making 4 equal ‘hops’ on the number line, each representing a multiplication of 1/3. After each ‘hop’, mark the new position on the number line. Once all ‘hops’ are completed, discuss where we land and what that represents. This visual representation helps students grasp the concept of fraction multiplication in a tangible way. Encourage students to draw their own number lines and perform the ‘hops’ with different fractions and whole numbers.

Your Turn: Multiplying Fractions on a Number Line – Multiply a unit fraction by a number – Use a number line for 1/5 x 5 – Mark 0, then jump in increments of 1/5 up to 5 – Find the product on the number line – Each jump represents 1/5; count 5 jumps – Share your results with the class | This slide is an interactive class activity where students will apply their knowledge of multiplying unit fractions by whole numbers using a number line. Provide each student with a number line, or have them draw one, and guide them through the process of multiplying 1/5 by 5. Encourage them to make five jumps on the number line, starting from zero, each jump representing 1/5. After completing the jumps, they should arrive at the whole number 1, which is the product of 1/5 multiplied by 5. Have students share their findings with the class to reinforce their understanding and to practice their communication skills. Offer support and clarification as needed, and consider providing additional examples if time allows.

Class Activity: Fraction Number Line Hopscotch – Draw number lines on the floor – Hop the multiplication of a fraction – Each hop represents multiplying by the unit fraction – Understand multiplication as repeated addition – E.g., 1/4 x 4: hop 1/4 four times to reach 1 – Discover the product through hops – Visualize the answer on the number line | This interactive activity is designed to help students visualize the concept of multiplying unit fractions by whole numbers. By drawing number lines on the floor and physically hopping the multiplication, students can see the repeated addition of fractions. For example, if the task is to multiply 1/4 by 4, students will hop 1/4 on the number line four times to reach the whole number 1. This kinesthetic approach reinforces the concept that multiplication is repeated addition. Prepare different scenarios for each student or pair, and ensure safety while hopping. After the activity, discuss as a class how the hops represented the multiplication process and how this method can be used to find the product of a unit fraction and a whole number.

Conclusion & Review: Mastering Unit Fractions – Excellent work with unit fractions! – Multiplication is finding parts of a whole – Think of multiplication as adding a fraction to itself multiple times – Practice with number lines is key – Number lines help visualize the fractions – Keep practicing and improving! | As we wrap up today’s lesson on multiplying unit fractions by whole numbers, it’s important to reinforce the concept that multiplication of fractions is not just a rote process, but a way of finding how many parts of a whole we have. Remind students that using number lines can make this concept more tangible by providing a visual representation of the fractions. Encourage them to continue practicing at home, using number lines to help them see the increments and understand the multiplication process. The more they practice, the more confident they will become in their ability to multiply unit fractions by whole numbers.