Your next great Lessn starts here.

Solving Multi-Step Equations with Fractions – Understand equations with fractions – Equations can include fractions as coefficients, e.g., 1/2x + 3 = 7 – Importance of multi-step equations – Solving these is crucial for algebraic proficiency and advanced math – Real-life equation applications – Used in budgeting, cooking, and construction measurements – Practice solving with examples – Example: Solve 3/4x – 5 = 11 to find x | This slide introduces students to the concept of multi-step equations that include fractional coefficients. It’s essential to explain that fractions are just numbers that represent a part of a whole and can be treated as such in equations. Emphasize the importance of these types of equations in developing algebraic skills, which are foundational for higher-level math. Provide real-world contexts where solving such equations is necessary, like dividing resources or adjusting recipes. Conclude with a guided example, solving a multi-step equation with a fractional coefficient, to demonstrate the process step-by-step.

Review: Solving One-Variable Equations – Recap of one-variable equations – Equations with one unknown, e.g., 2x + 3 = 7 – Goal: Isolate the variable – To find the value of the variable, make it alone on one side – Examples of solved equations – Review past examples like x/3 – 2 = 4 or 5x + 1 = 16 – Transition to fractional coefficients – Introduce equations with fractions, e.g., (3/4)x = 6 | Begin with a brief review of one-variable equations to refresh students’ memory. Emphasize the goal of solving these equations: to isolate the variable on one side. Go through a few examples that the class has previously solved to ensure understanding. This will set the stage for the new concept of solving equations that include fractional coefficients, which adds a layer of complexity but follows the same principles. Encourage students to think of the fraction as another number to work with, and remind them of the methods to clear fractions from equations.

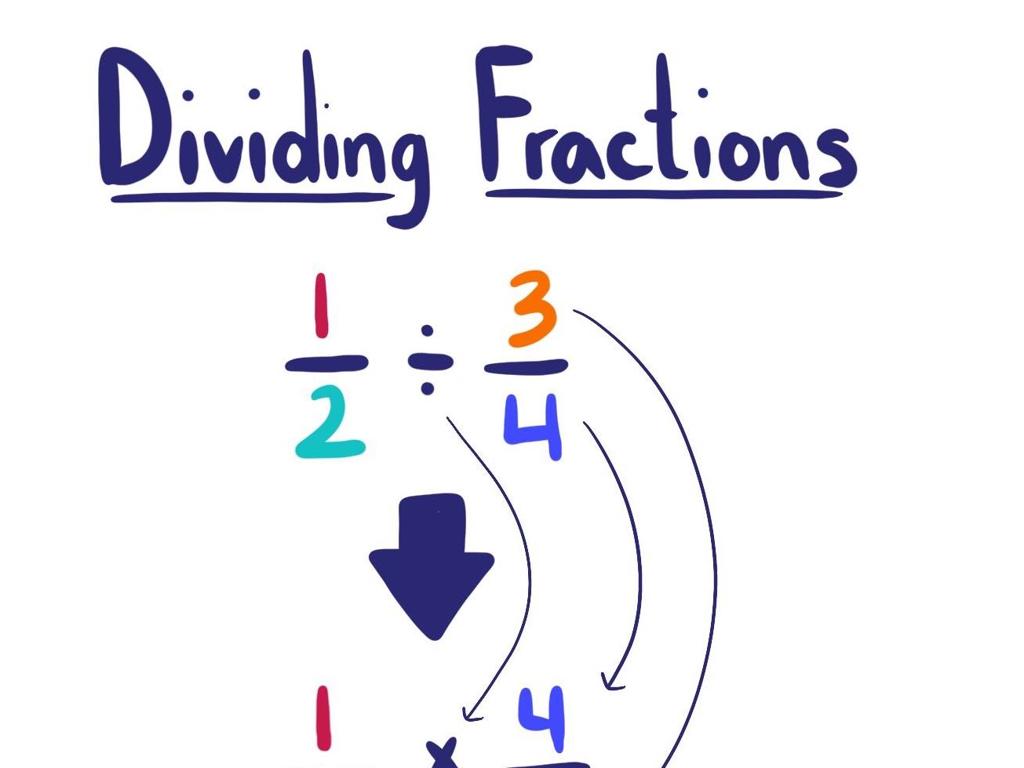
Solving Equations with Fractional Coefficients – Understanding fractions – Fractions represent parts of a whole – Fractions as coefficients – Fractions multiply the variable, e.g., (1/2)x – Simplifying complex equations – Combine like terms, clear fractions – Solving step-by-step – Apply inverse operations to isolate the variable | This slide introduces students to the concept of using fractions within algebraic equations. Begin by ensuring that students have a solid understanding of fractions as parts of a whole. Then, explain how these fractions can act as coefficients, multiplying the variable in an equation. Emphasize the importance of simplifying equations by combining like terms and clearing fractions to make solving easier. Finally, guide students through solving multi-step equations with fractional coefficients by using inverse operations to isolate the variable. Provide examples and practice problems to reinforce the concepts.

Solving Multi-Step Equations with Fractions – Combine like terms first – Add or subtract terms with the same variable – Clear fractions from the equation – Multiply every term by the LCD to eliminate fractions – Isolate the variable step by step – Use inverse operations to get the variable alone – Check your solution – Substitute back to verify the solution works | This slide outlines the systematic approach to solving multi-step equations that include fractional coefficients. Start by combining like terms to simplify the equation. Next, clear any fractions by multiplying every term by the least common denominator (LCD), which will leave you with an equation without fractions. Then, isolate the variable by performing inverse operations step by step, maintaining the balance of the equation. Finally, always check your solution by substituting it back into the original equation to ensure it satisfies the equation. Encourage students to practice these steps with different equations and to always verify their answers.

Solving Equations with Fractional Coefficients – Simplify the equation first – Combine like terms and clear parentheses – Multiply to eliminate fractions – Use the LCD to multiply each term and clear fractions – Solve the simplified equation – Isolate the variable using inverse operations – Check the solution’s validity – Substitute the solution back into the original equation | Begin by simplifying the equation: combine like terms and remove parentheses where possible. Next, to eliminate fractions, multiply each term by the least common denominator (LCD). After clearing the fractions, you’ll have a simplified equation that you can solve using inverse operations (addition, subtraction, multiplication, and division) to isolate the variable. Once you find the solution, it’s crucial to check that it works by substituting it back into the original equation. This process ensures the solution is correct. Encourage students to practice these steps with different problems to gain confidence in solving equations with fractional coefficients.

Class Activity: Solving Equations with Fractions – Solve the given equations – Apply learned solving steps – Show your solution process – Break down the steps taken to solve the equations – Share solutions with the class – Be prepared to explain your answers and methods | This slide is meant to engage students in a hands-on activity where they apply the methods taught for solving multi-step equations with fractional coefficients. Provide a set of equations for students to solve independently, ensuring they vary in difficulty to cater to different skill levels. Encourage students to write down each step of their process, which will aid in their understanding and make it easier to share their solutions. In the next class, create a collaborative environment where students can discuss the different approaches they took and learn from each other’s methods. This activity will reinforce their learning and help identify any areas where they may need further clarification or practice.

Class Activity: Equation Relay Challenge – Form small relay teams – Each team solves equations – Tackle multi-step equations with fractional coefficients – First to finish correctly wins – Verify each other’s work for accuracy – Celebrate teamwork and accuracy | This activity is designed to encourage collaboration and reinforce the concept of solving multi-step equations with fractional coefficients. Divide the class into small groups, ensuring a mix of abilities in each team. Provide each group with a set of equations that they must solve together. The first team to complete all equations correctly wins the challenge. Emphasize the importance of accuracy over speed to prevent careless mistakes. Possible activities for different groups could include solving equations with different levels of complexity, incorporating word problems, or having a mix of both. Monitor the groups to provide guidance and ensure that all students are participating. Celebrate all teams for their effort and teamwork, and review the solutions as a class to reinforce learning.

Wrapping Up: Equations with Fractions – Recap of solving multi-step equations – Practice and perseverance are key – Homework: More fractional equations – Solve 10 equations with different fractional coefficients – Keep trying, even if it’s challenging – Remember: Mistakes are just learning opportunities | As we conclude today’s lesson on solving multi-step equations with fractional coefficients, it’s important to emphasize the role of consistent practice and perseverance in mastering math concepts. For homework, students are assigned to solve an additional set of 10 equations, which will help reinforce the techniques learned in class. Remind students that encountering difficulties is a natural part of the learning process, and persistence will lead to improvement. Encourage them to approach each problem methodically, and to review their steps if they arrive at an incorrect solution. The goal is to build confidence and proficiency in working with fractional coefficients.