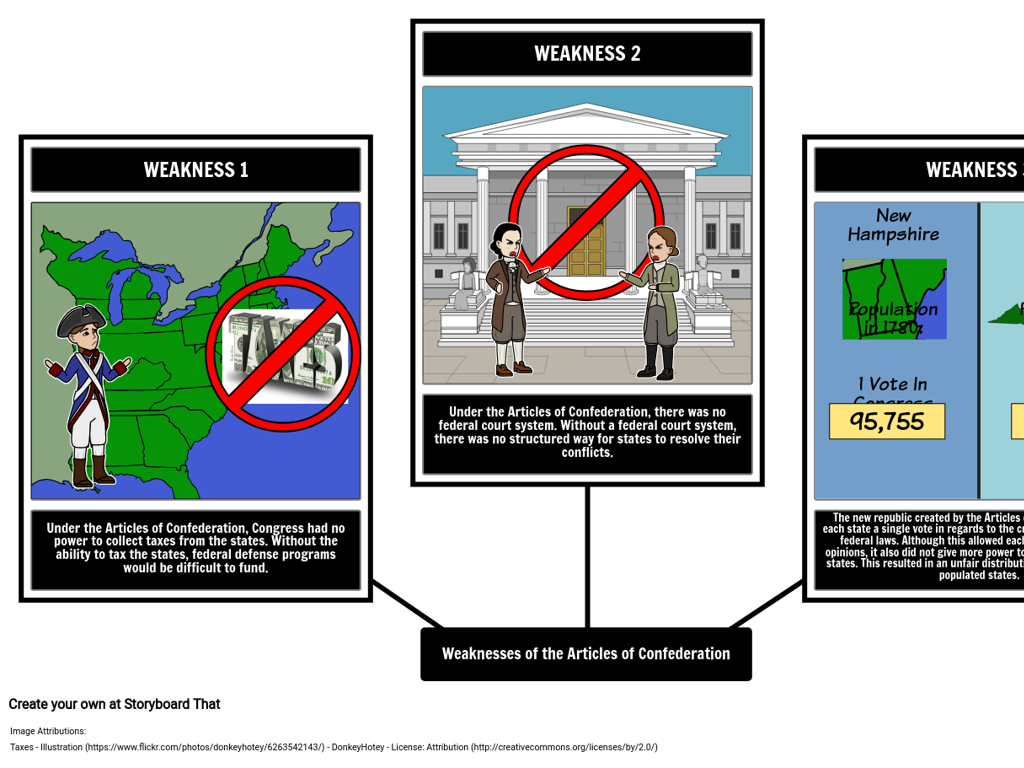
Apply Multiplication And Division Rules
Subject: Math
Grade: Seventh grade
Topic: Operations With Rational Numbers
Please LOG IN to download the presentation. Access is available to registered users only.
View More Content
Multiplication & Division with Rational Numbers
– Define rational numbers
– Numbers expressed as a fraction of two integers, e.g., 1/2, 3/4, -5/6
– Rules for multiplying rationals
– To multiply, simply multiply the numerators and denominators respectively
– Rules for dividing rationals
– To divide, multiply by the reciprocal of the divisor
– Real-life applications
– Understanding these rules helps in situations like splitting bills or converting measurements
|
This slide introduces students to the concept of rational numbers and the rules for multiplying and dividing them. Start by defining rational numbers and ensure students understand they can be written as fractions. Explain that multiplying rational numbers involves multiplying the numerators together and the denominators together. For division, teach them to multiply by the reciprocal (flipping the second fraction). Emphasize the importance of these operations in real-life scenarios, such as dividing a pizza among friends (division) or doubling a recipe (multiplication). Provide examples and encourage students to think of other situations where these operations are used.
Understanding Rational Numbers
– Define rational numbers
– Numbers expressed as a fraction of two integers, e.g., 1/2, 3/4, 5/1
– Examples vs. non-examples
– Examples: 1/2, 3/4; Non-examples: Pi (À), square root of 2
– Rational numbers in daily life
– Money, measurements, and cooking fractions
– Practice identifying rational numbers
|
This slide introduces the concept of rational numbers, which are any numbers that can be expressed as a fraction of two integers, where the denominator is not zero. Provide clear examples of rational numbers, such as common fractions students encounter in daily life. Contrast these with non-examples like irrational numbers, which cannot be expressed as simple fractions. Highlight how rational numbers are used in everyday contexts, such as in dealing with money (dollars and cents), measuring ingredients for a recipe, or determining lengths and heights. Encourage students to practice identifying rational numbers from a list of various numerical expressions to reinforce their understanding.
Multiplying Rational Numbers
– Rules for multiplication
– Multiply numerators and denominators separately. Same signs result in a positive product, while different signs give a negative product.
– Consider the sign of numbers
– Positive x Positive = Positive, Negative x Negative = Positive, Positive x Negative = Negative
– Multiplying fractions step-by-step
– To multiply 3/4 by 2/5, multiply 3 by 2 and 4 by 5. The product is 6/20, which simplifies to 3/10.
– Multiplying decimals
– Align the numbers by their rightmost digits, ignoring the decimal point. Multiply as whole numbers, then place the decimal in the product.
|
This slide aims to solidify the students’ understanding of multiplying rational numbers, including both fractions and decimals. Start by explaining the rules for multiplication, emphasizing the importance of the signs of the numbers. Use examples to show that a positive times a positive or a negative times a negative both yield a positive product, while a positive times a negative results in a negative product. Walk through the process of multiplying fractions, ensuring students are comfortable with multiplying numerators and denominators and simplifying the result. For decimals, demonstrate the multiplication process similar to whole numbers and the correct placement of the decimal point in the answer. Provide practice problems for each case to reinforce learning.
Dividing Rational Numbers
– Rules for dividing rational numbers
– Keep, Change, Flip: Keep the first number, change division to multiplication, flip the second number to its reciprocal.
– Understanding reciprocals in division
– A reciprocal is 1 divided by the number. For example, the reciprocal of 2/3 is 3/2.
– Example: Dividing fractions
– To divide 3/4 by 2/3, we multiply 3/4 by the reciprocal of 2/3, which is 3/2.
– Example: Dividing decimals
– Dividing 6.5 by 2.5, rewrite as 6.5 * 1/2.5 or 6.5 * 0.4.
|
When teaching division of rational numbers, start with the ‘Keep, Change, Flip’ rule to simplify the concept. Explain that the reciprocal of a number is what you multiply that number by to get 1. Provide examples of dividing fractions by demonstrating the multiplication of a fraction by the reciprocal of another. For decimals, show how to rewrite the division as multiplication by the reciprocal, which may involve converting the divisor to a decimal if it isn’t already. Use visual aids or interactive tools to help students visualize the process. Practice problems should include both fractions and decimals to solidify understanding.
Multiplication & Division with Negative Numbers
– Understand the Rule of Signs
– A positive times a positive equals a positive, and the same goes for negatives. A positive times a negative equals a negative.
– Examples with negatives
– Multiply -3 x 4 and divide -12 / -3. What are the results?
– Work on practice problems
– Solve -6 x -2, -18 / 3, 7 x -1, and -8 / -2.
– Discuss answers and strategies
|
This slide introduces students to the fundamental rules for multiplying and dividing with negative numbers, known as the Rule of Signs. It’s crucial for students to understand that a positive number multiplied by a positive, or a negative by a negative, results in a positive product, while a positive multiplied by a negative results in a negative product. The same rules apply for division. Provide clear examples to illustrate these rules, and then give students a set of practice problems to apply what they’ve learned. Encourage students to work through the problems and then discuss the answers as a class, focusing on the strategies used to determine the correct sign of the result.
Applying Multiplication & Division in Real-World Scenarios
– Apply learned concepts to word problems
– Solve real-world mathematical problems
– Group activity: collaborative problem-solving
– Work together to find solutions to given problems
– Explain your methods and reasoning
– Share your problem-solving process with the class
|
This slide is designed to encourage students to apply the multiplication and division rules they’ve learned to solve word problems that they might encounter outside the classroom. The group activity is meant to foster collaborative learning, where students can discuss and solve problems together, thereby enhancing their understanding of the concepts. Teachers should prepare a set of real-world problems that require the application of multiplication and division with rational numbers. During the activity, students should be encouraged to discuss their approach to solving the problems and to explain their reasoning to the rest of the class. This will not only help them understand the material better but also improve their communication and critical thinking skills.
Class Activity: Multiplication & Division Relay
– Form teams of four students
– Solve problems as a group
– Work on assigned multiplication and division problems
– Each team presents a problem
– Choose one problem for presentation
– Discuss solutions collectively
– Share different solving strategies
|
This activity is designed to promote teamwork and collaborative problem-solving among students. Each team will work together to solve a set of multiplication and division problems related to rational numbers. After solving the problems, each team will select one problem to present to the class, explaining their solution process and the multiplication or division rules applied. The teacher should prepare a diverse set of problems of varying difficulty to cater to different skill levels. Possible activities include: a relay race where each student solves a part of the problem, a ‘gallery walk’ where teams rotate to solve different problems, or a ‘jigsaw’ where each student becomes an expert on one problem and then teaches it to their group. The goal is to reinforce the understanding of multiplication and division rules through practice and peer teaching.
Wrapping Up: Multiplication & Division of Rational Numbers
– Review key multiplication rules
– Review key division rules
– Emphasize the value of practice
Practice solidifies understanding and improves skills.
– Homework: Practice worksheet
Complete the worksheet on multiplying and dividing rational numbers.
|
As we conclude today’s lesson, it’s important to recap the rules for multiplying and dividing rational numbers. Remember, when multiplying, the product of two rational numbers with the same sign is positive, while with different signs is negative. For division, the quotient of two rational numbers with the same sign is positive, and with different signs is negative. Emphasize to students the importance of practice in mastering these concepts. For homework, students are assigned a worksheet that provides a variety of problems to help them apply what they’ve learned and reinforce their skills. Encourage them to attempt all problems and remind them that practice is key to becoming proficient in working with rational numbers.