Your next great Lessn starts here.

Understanding Proportional Relationships – Proportions in daily life – Ratios in recipes or speed and time in travel – Defining proportional relationships – A relationship where two quantities increase at the same rate – Recognizing examples – Mixing paint colors, buying fruits by weight – Graphing proportions – Plotting points on a graph to see the constant rate of change | This slide introduces the concept of proportional relationships by connecting it to everyday experiences, such as following a recipe or calculating travel time. Explain that a proportional relationship is when two quantities increase or decrease at the same rate, which can be represented by a straight line when graphed. Provide examples like mixing paints in a consistent ratio or purchasing fruits where the price is directly proportional to the weight. Emphasize the importance of understanding proportions in various real-life situations. Demonstrate how to complete a table of values for a proportional relationship and how to graph these values to show the constant rate of change.

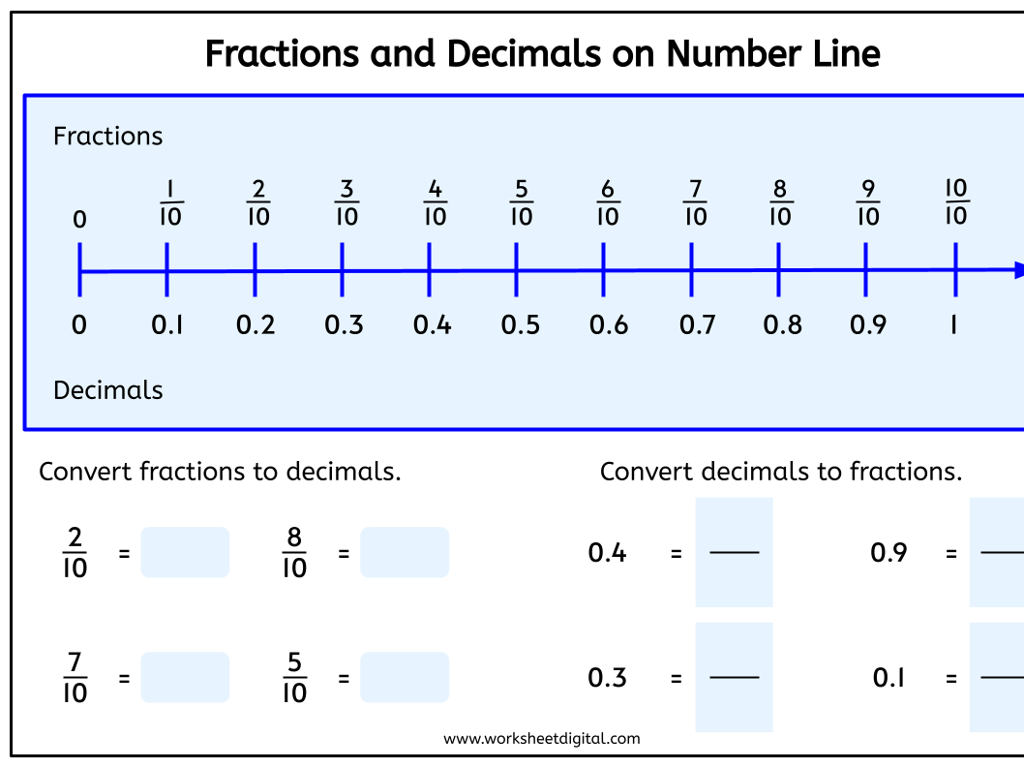
Defining Proportional Relationships – Two quantities change at the same rate – Understanding constant of proportionality – It’s the ratio that is the same for all pairs of data – Identifying proportional relationships in tables – If the ratio of one pair of numbers is equal to the ratio of another pair, they are proportional – Graphing proportional relationships – Use a table of values to plot points on a graph | This slide introduces the concept of proportional relationships, a fundamental aspect of understanding ratios and linear functions. Begin by explaining that a proportional relationship exists when two quantities increase or decrease at the same rate, which can be observed in real-life situations like recipes or speed. Introduce the constant of proportionality, which is the consistent ratio between two quantities. Teach students how to identify proportional relationships by checking if the ratio between corresponding values in a table remains constant. Finally, demonstrate how to graph proportional relationships using a table of values, plotting points on a coordinate plane to visualize the linear relationship. Encourage students to practice with different tables and to recognize the straight-line graphs that result from proportional relationships.

Creating a Table from a Proportional Relationship – Start with a real-world problem – Example: If 3 apples cost $1.50, how much do 6, 9, 12 apples cost? – Find the constant of proportionality – Constant of proportionality (k) is the ratio of total cost to number of apples – Fill in the table with the constant – Use k to determine the cost for different numbers of apples – Graph the proportional relationship – Plot the pairs (number of apples, cost) on a graph | This slide is aimed at teaching students how to create a table from a proportional relationship, starting with a relatable real-world problem. The constant of proportionality, or unit rate, is the key to filling in the table. It’s the value that remains constant when comparing two directly proportional quantities. Once the constant is determined, students can complete the table by multiplying the constant with the corresponding values. Finally, students should graph the relationship to visually represent the proportionality. The graph should be a straight line passing through the origin, showing the constant rate of change. Encourage students to come up with their own real-world problems and practice creating tables and graphs to solidify their understanding.

Graphing Proportional Relationships – Plot points from a table – Use the table values to plot points on the graph. – Recognize straight-line graphs – A proportional relationship graph is always a straight line. – Interpret graph points – Each point on the graph represents a ratio equivalent to others. – Relate graphs to real-world – Understand how graphs translate to everyday situations. | This slide aims to teach students how to graph proportional relationships by plotting points from a table onto a coordinate plane. Emphasize that the graph of a proportional relationship will always form a straight line, which indicates a constant rate of change. Students should learn to interpret the meaning of each point on the graph as a representation of the relationship between two quantities. Relate this concept to real-world scenarios, such as comparing the cost to the number of items bought, to solidify their understanding. Encourage students to practice by creating their own tables and graphs and interpreting them.

Graphing Proportional Relationships: Practice – Work through examples together – Teacher-led example on the board – Pair up for practice problems – Choose a partner, solve problems from worksheet – Discuss solutions and methods – Share different approaches with the class – Reflect on learning outcomes – Understand how to identify and graph proportional relationships | This slide is designed to facilitate active learning through collaboration and discussion. Begin by solving a proportional relationship example as a class, ensuring to involve students in the process. Then, have students pair up to tackle practice problems, encouraging them to communicate and assist each other. After completing the problems, lead a class discussion on the various methods used and solutions found, highlighting different strategies and common mistakes. Conclude by summarizing the key points of identifying and graphing proportional relationships, and reflect on the learning outcomes to reinforce the concepts covered.

Class Activity: Graph Your Own! – Receive a table with values – Complete the table and graph – Use the values to plot points on a graph and draw a line through them – Share and explain your graph – Pair up with a classmate to discuss what your graph shows about the relationship – Discuss the proportional relationship – How does the graph show a constant rate of change? | In this activity, students will apply their understanding of proportional relationships by completing a given table and graphing the data. Distribute tables with incomplete values to the students and instruct them to fill in the missing information. Once the tables are complete, students will use the data to plot points on a graph and draw a line that represents the proportional relationship. After graphing, students will pair up to explain their graphs to each other, discussing how the graph illustrates a constant rate of change. This peer-to-peer interaction will reinforce their comprehension. As a teacher, circulate the room to assist and ask probing questions to deepen their understanding. Possible variations of the activity could include different rates of change or using real-world scenarios to generate the tables.

Wrapping Up: Proportional Relationships – Recap: What are proportional relationships? – Why are they important? – Homework: Real-world proportional relationships – Find an example like speed and travel time, or recipe ingredients. – Create and graph your findings – Use a table to organize your data, then draw a graph to represent it. | As we conclude today’s lesson, remind students of the key characteristics of proportional relationships, such as constant ratios and straight-line graphs through the origin. Emphasize the importance of recognizing these relationships in various contexts, as they are foundational for understanding more complex mathematical concepts. For homework, students should find a real-world example of a proportional relationship, such as the relationship between distance and time at a constant speed, or the amount of ingredients in proportion to servings in a recipe. They should create a table of values and graph the relationship, practicing how to visually represent proportional relationships. This exercise will reinforce their understanding and help them see the practical applications of what they’ve learned.