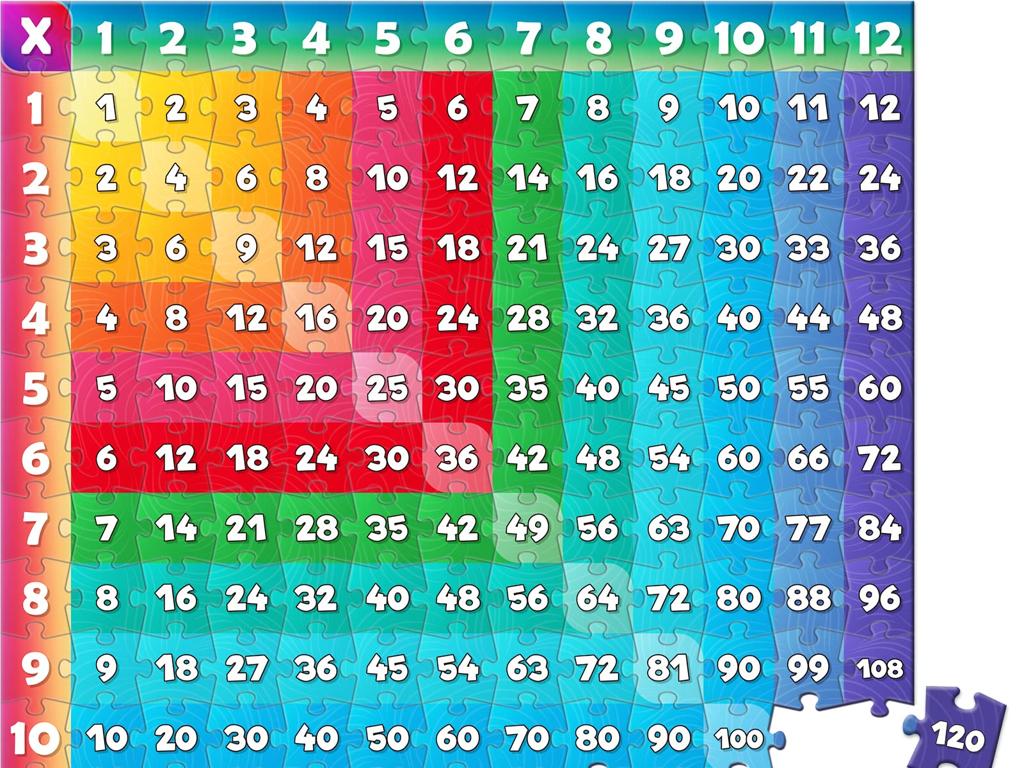
Find The Constant Of Proportionality From A Table
Subject: Math
Grade: Eighth grade
Topic: Proportional Relationships
Please LOG IN to download the presentation. Access is available to registered users only.
View More Content
Finding the Constant of Proportionality
– Understand proportional relationships
– A proportional relationship has a constant ratio between two quantities.
– Real-life proportionality examples
– Examples: recipes, currency exchange, speed/distance
– Today’s goal: constant from a table
– The constant of proportionality is the ratio y/x for every x,y pair in the table.
– Practice with real data
– We’ll use tables from real-life scenarios to find this constant.
|
This slide introduces the concept of proportional relationships and sets the objective for the class, which is to find the constant of proportionality from a table. Begin by explaining that in a proportional relationship, two quantities increase or decrease at the same rate. Use relatable examples like recipes (ingredients to servings) or currency exchange rates to illustrate this concept. Then, guide students on how to find the constant of proportionality by dividing the y-value by the x-value for each pair in a table and verifying that this ratio remains constant. Provide tables with real-life data for students to practice this skill. Encourage students to explain their reasoning and how they determined the constant in each example.
Understanding Proportionality
– Define proportionality
– Proportionality: relationship where two quantities increase or decrease at the same rate
– Constant of proportionality
– The constant ratio between two proportional quantities, often represented by ‘k’
– Proportional vs. non-proportional
– Compare tables where ‘k’ is constant (proportional) and where ‘k’ changes (non-proportional)
– Examples in tables
– Show tables where the ratio of y to x is the same (proportional) and where it isn’t (non-proportional)
|
This slide introduces the concept of proportionality, which is a cornerstone of understanding relationships between quantities in mathematics. Begin by defining proportionality as a consistent, linear relationship between two variables. Explain the constant of proportionality as the unchanging ratio in a proportional relationship, often denoted by ‘k’. Illustrate the difference between proportional and non-proportional relationships using examples in tabular form, ensuring to highlight how in proportional tables the ratio of one quantity to another remains constant. Encourage students to think of real-life examples of proportional relationships, such as recipes or speed and travel time, to solidify their understanding.
Exploring Tables: Proportional Relationships
– Organizing data in tables
– Arrange data in rows and columns for clarity
– Identifying proportional relationships
– Proportional relationships have a constant ratio
– Understanding table ratios
– Ratios compare two quantities in a table
– Finding the constant of proportionality
– Divide y-value by x-value to find the constant
|
This slide introduces students to the concept of organizing data in tables and identifying proportional relationships within them. Emphasize the importance of arranging data clearly to make it easier to analyze. Explain that in proportional relationships, the ratio between corresponding x and y values is constant. Show how to understand ratios by comparing two quantities and how these ratios can help us find the constant of proportionality by dividing the y-value by the x-value. Provide examples of tables with proportional and non-proportional relationships for students to practice identifying them. Encourage students to work on problems where they find the constant of proportionality from given tables.
Finding the Constant of Proportionality
– Steps to determine proportionality
– Identify pairs, divide y by x to find k
– Divide to find the constant
– For each pair (x, y), divide y by x
– Verify the constant in the table
– Check that y/x is consistent for all pairs
– Practice with an example
– Use a sample table to apply these steps
|
This slide is aimed at teaching students how to find the constant of proportionality (k) from a given table. Start by explaining the steps: identifying the pairs of corresponding values, dividing the output (y) by the input (x) to find k. Emphasize the importance of consistency in the value of k across all pairs in the table to confirm proportionality. Provide a clear example with a table of values for students to practice this process. Encourage students to work through the example provided and verify the constant themselves. This reinforces the concept and ensures they understand how to apply the steps to different problems.
Finding the Constant of Proportionality
– Work through a sample problem
– Find the constant in a table
– Divide the y-value by the x-value for each pair
– Verify the constant’s consistency
– Ensure the constant is the same across all pairs
– Review the steps taken
|
This slide is aimed at demonstrating how to find the constant of proportionality from a table. Start with a worked example, guiding students through the process step by step. Show them how to divide the output (y-value) by the input (x-value) for each pair of numbers in the table to find the constant. Emphasize the importance of consistency; the constant should be the same for all pairs. If it is not, there may be a mistake. After finding the constant, check the work by multiplying each x-value by the constant to see if the original y-values are obtained. This reinforces the concept and ensures students understand the process. Encourage students to ask questions and work through additional examples for practice.
Practice: Finding the Constant of Proportionality
– Work through examples together
– Identify constants in various tables
– Look for a consistent ratio across the table’s values
– Pair up for group activity
– Discuss strategies and compare findings with a partner
– Solve practice tables collaboratively
– Use the formula k = y/x to find the constant in each table
|
This slide is designed to engage students in hands-on practice with finding the constant of proportionality from tables. Start by solving a few examples as a class to demonstrate the process. Emphasize looking for a consistent ratio (y divided by x) in the tables provided. Then, have students pair up to work through practice problems, encouraging collaboration and discussion. Provide a variety of tables with different constants to ensure a comprehensive understanding. As a teacher, circulate the room to offer guidance and support. After the activity, regroup and discuss as a class, allowing pairs to share their strategies and solutions. This will reinforce the concept and allow for peer learning.
Class Activity: Create Your Own Table
– Form groups and create a table
– Ensure table has a constant proportionality
– A constant ratio between two variables, for example, x and y where y=kx
– Present table to the class
– Explain your constant finding process
– Describe steps taken to determine the constant in your table
|
This activity is designed to reinforce the concept of constant of proportionality through hands-on learning. Divide the class into small groups and provide them with instructions to create a table of values that demonstrates a proportional relationship between two variables. Each group should calculate the constant of proportionality (k) for their table. After creating their tables, students will present their findings to the class, explaining how they determined the constant. For the teacher: Prepare to guide students on how to calculate the constant of proportionality, and have a few examples ready in case some groups struggle. Possible activities for different groups could include creating tables based on real-life scenarios such as recipes, speed/distance, or cost of items. Encourage students to think creatively and relate the concept to everyday situations.
Conclusion & Homework: Proportionality Constants
– Recap: Finding proportionality constants
– Review the steps to determine the constant of proportionality from a given table.
– Real-world application significance
– Understanding this concept is crucial for solving problems in science, economics, and more.
– Homework: Analyze 3 tables
– Identify the constant of proportionality in each table and explain your reasoning.
|
As we conclude today’s lesson, remember that finding the constant of proportionality is a key skill in understanding proportional relationships. This skill is not only important in mathematics but also has practical applications in various fields such as physics, engineering, and finance. For homework, students are expected to find and interpret the constant of proportionality from three different tables. This will reinforce their understanding and prepare them for more complex problems. Encourage students to explain each step clearly and to consider the real-world relevance of this concept. In the next class, we will review the homework and discuss any challenges faced.