Your next great Lessn starts here.

Exploring the Pythagorean Theorem – Grasp the Pythagorean Theorem – a^2 + b^2 = c^2, where c is the hypotenuse – Theorem’s real-world use cases – Used in construction, navigation, and more – Today’s focus: missing triangle sides – Use the theorem to solve for unknown sides – Practice with right-angled triangles | This slide introduces the Pythagorean Theorem, a fundamental concept in geometry that relates the lengths of the sides of a right-angled triangle. Start by explaining the theorem’s formula, a^2 + b^2 = c^2, and ensure students understand that ‘c’ represents the hypotenuse. Discuss how this theorem is not just a mathematical idea but also a practical tool used in various real-life situations such as construction, navigation, and art. The main objective of today’s lesson is to apply the theorem to find missing sides of right-angled triangles, which will involve identifying the hypotenuse, the longest side opposite the right angle, and the legs, the other two sides. Provide examples and exercises for students to practice calculating unknown side lengths, reinforcing their understanding through application.

Understanding the Pythagorean Theorem – Definition of Pythagorean Theorem – In right triangles, the sum of squares of legs equals the hypotenuse square. – Formula: a^2 + b^2 = c^2 – ‘a’ and ‘b’ are legs, ‘c’ is the hypotenuse. – Hypotenuse in right triangles – The longest side opposite the right angle. – Practical applications – Use it to solve real-world problems, like in construction. | The Pythagorean Theorem is a fundamental concept in mathematics, specifically in geometry, that relates the lengths of the sides of a right-angled triangle. The formula a^2 + b^2 = c^2 allows students to calculate the length of any side of the triangle if the lengths of the other two are known. This theorem is not only a cornerstone of geometry but also has practical applications in various fields such as construction, navigation, and physics. During the lesson, emphasize the theorem’s proof and derivation, and provide examples where students can apply the theorem to find missing side lengths in right-angled triangles. Encourage students to think of situations where they could use the theorem outside the classroom.

Identifying Parts of a Right Triangle – Hypotenuse: longest side – Opposite the right angle, crucial for theorem – Legs: sides forming right angle – Adjacent to the right angle, play a key role in calculations – Practice identifying parts – Use example triangles to locate hypotenuse and legs – Understand their roles in Pythagoras’ theorem | This slide introduces students to the fundamental components of a right triangle, which is essential for understanding the Pythagorean theorem. The hypotenuse is the longest side of the triangle and is always opposite the right angle. The legs are the two sides that form the right angle. During the practice activity, students should identify these parts in various right triangles to reinforce their understanding. This knowledge is crucial for applying the Pythagorean theorem to find the length of a missing side. Ensure that students can distinguish between the hypotenuse and the legs, as this will be the foundation for solving problems using the theorem.

Finding the Missing Leg in Right Triangles – Example: Given one leg and hypotenuse – If a right triangle has a leg of 6 units and hypotenuse of 10 units, find the other leg. – Rearrange Pythagorean theorem – To find the missing leg (a), use the formula a^2 = c^2 – b^2, where c is the hypotenuse and b is the known leg. – Calculate the missing leg length – Take the square root of the difference between the square of the hypotenuse and the square of the known leg. – Discuss the solution steps – Step-by-step calculation ensures understanding of the theorem’s application in solving for a missing side. | This slide focuses on applying the Pythagorean theorem to find the length of a missing leg in a right triangle when the length of one leg and the hypotenuse are known. Start with a concrete example to illustrate the problem. Guide students through rearranging the theorem to solve for the missing leg. Emphasize the importance of squaring the lengths, subtracting, and then finding the square root to calculate the missing length. Discuss each step in detail to ensure students grasp the concept and can apply it to similar problems. Encourage students to practice with additional problems for mastery.

Finding the Hypotenuse with Pythagorean Theorem – Start with an example problem – Given legs a=3, b=4, find the hypotenuse c – Apply the Pythagorean formula – Use c^2 = a^2 + b^2 to express c in terms of a and b – Calculate the hypotenuse length – For a=3, b=4, c^2 = 3^2 + 4^2, so c^2 = 9 + 16 – Discuss the solution steps – Take the square root of c^2 to find c: (9+16) = 25 = 5 | This slide introduces the process of finding the hypotenuse of a right triangle using the Pythagorean theorem. Start with a simple example where both legs are known, such as a 3-4-5 triangle. Explain the formula c^2 = a^2 + b^2, where c represents the length of the hypotenuse and a and b represent the lengths of the other two sides. Walk through the calculation step by step, ensuring to square the lengths of both legs, add the results, and then take the square root to find the length of the hypotenuse. Emphasize the importance of each step and how the theorem allows us to find the missing side length in right-angled triangles. Encourage students to practice with different sets of leg lengths to become comfortable with the theorem.

Pythagorean Theorem: Practice Problems – Solve problems as a class – Attempt problems individually first – Try to find the missing side without help to test your understanding – Review solutions collectively – Discuss the steps taken and answer any questions about the process – Understand the theorem application – Apply the a^2 + b^2 = c^2 formula to different triangles | This slide is focused on reinforcing the students’ understanding of the Pythagorean theorem through practice problems. Start by working through problems as a class to demonstrate the process. Encourage students to solve the problems on their own, fostering independence and confidence in their math skills. After individual attempts, review the solutions together to ensure comprehension and correct any misunderstandings. Emphasize the application of the theorem in various scenarios, not just in the classroom. Provide additional problems of varying difficulty for students to work on, and consider creating small groups to facilitate peer learning. Remember to praise effort and correct reasoning as much as correct answers to build a positive learning environment.

Real-World Applications of the Pythagorean Theorem – Used by architects and engineers – To determine distances and structural integrity – Navigation and map reading – Helps in finding shortest path between two points – Solving real-life problems – Apply theorem to calculate distances indirectly – Open discussion for questions | This slide aims to show students how the Pythagorean theorem is applied beyond the classroom, particularly in professions like architecture and engineering, where it’s used to calculate distances and ensure the structural integrity of buildings and bridges. In navigation, it helps with map reading and finding the shortest route between two points. Encourage students to think of situations where they can apply the theorem to solve real-life problems. The open discussion at the end is to address any uncertainties and to help students connect the theorem with practical scenarios they may encounter.

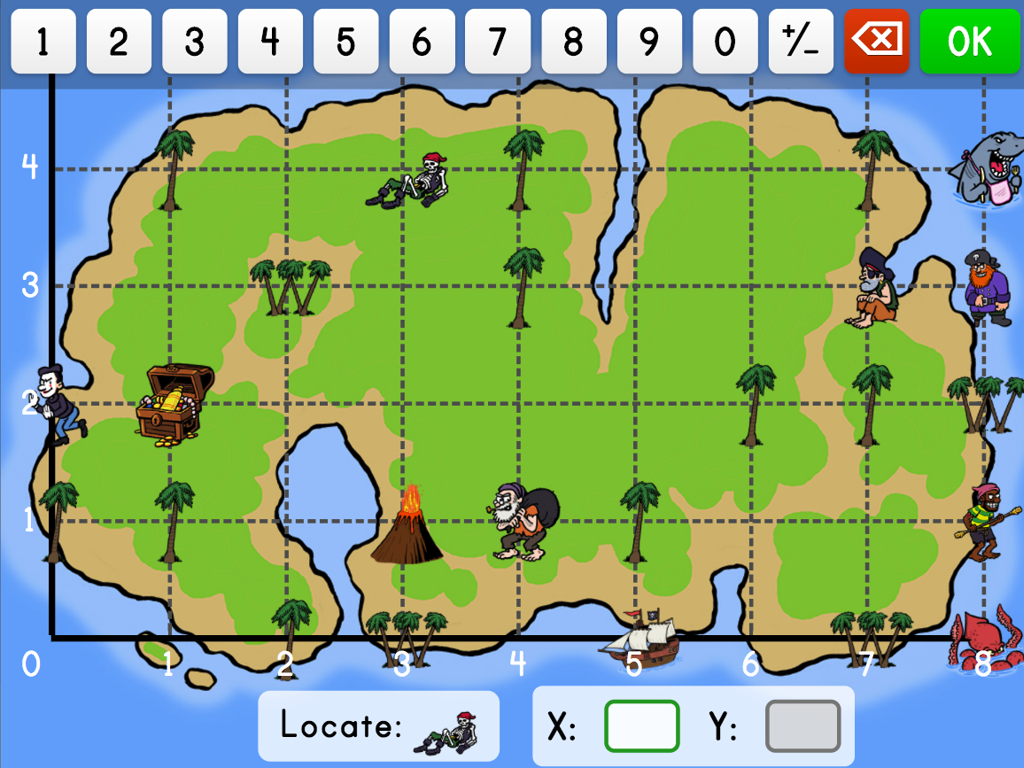
Class Activity: Find Your Way – Receive a map with right-angled paths – Use Pythagorean theorem for distance – Apply a^2 + b^2 = c^2 to find missing lengths – Practical application of theorem – Enhance understanding of concept | In this engaging class activity, students will be provided with maps that include various right-angled paths. They will be tasked with using the Pythagorean theorem to calculate the distance between points on the map. This hands-on exercise will not only reinforce their comprehension of the theorem but also demonstrate its practical use in real-world scenarios. For the activity, consider creating maps that resemble their school or local neighborhood to make the activity more relatable. Possible variations of the activity could include finding the shortest route between two points, calculating distances for a scavenger hunt, or even creating their own map with right-angled paths to challenge classmates.

Wrapping Up: Pythagorean Theorem – Recap: Pythagorean theorem formula – a^2 + b^2 = c^2, find missing sides of right triangles – Homework: Practice problems assigned – Solve for legs and hypotenuse in given exercises – Next class: Advanced applications – We’ll explore how to use the theorem in 3D shapes – Keep practicing and ask questions! | As we conclude today’s lesson on the Pythagorean theorem, remind students of the formula a^2 + b^2 = c^2 and its application to right-angled triangles. For homework, assign problems that require finding both legs and the hypotenuse to reinforce their understanding. Let them know that in the next class, we will delve into more complex applications of the Pythagorean theorem, including in three-dimensional shapes. Encourage students to practice the theorem and bring any questions they have to the next class for clarification.