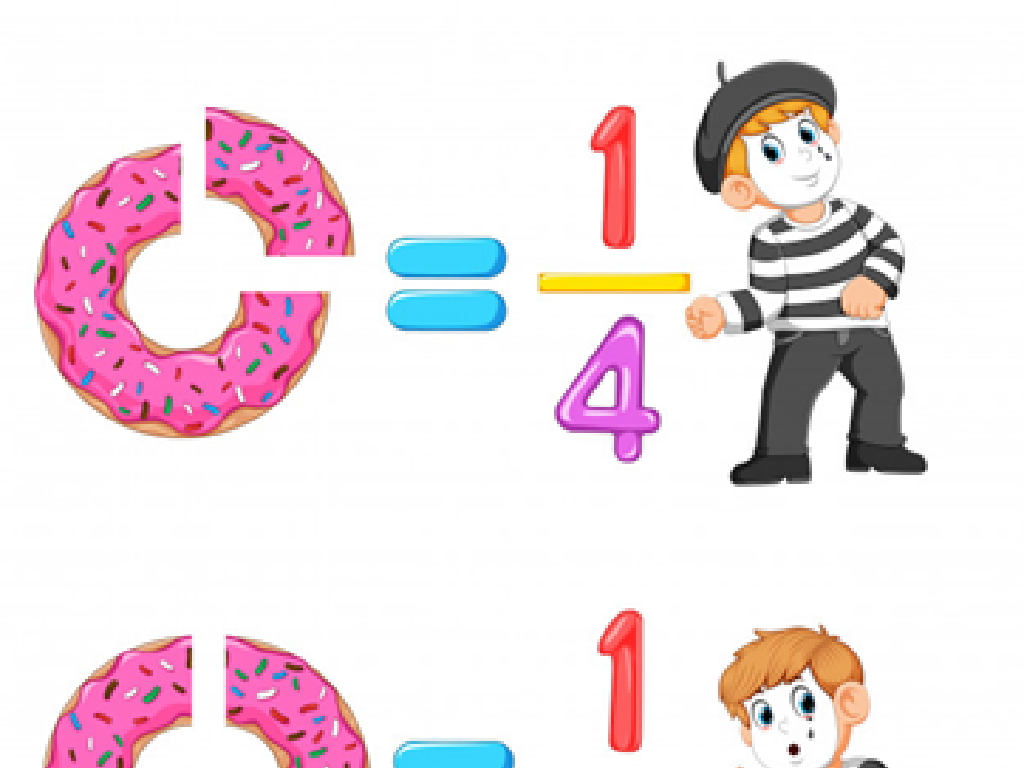Your next great Lessn starts here.

Welcome to Rational Numbers! – Understanding Rational Numbers – Numbers that can be expressed as a fraction – Rational numbers in daily life – Used in cooking, budgeting, and measuring – Review: fractions and integers – Fractions are part of a whole, integers are whole numbers – Placing numbers on a number line – Visualize and compare rational numbers | This slide introduces the concept of rational numbers, which are any numbers that can be expressed as the quotient or fraction of two integers. The presentation will show how these numbers are not abstract concepts but are used in everyday activities such as cooking (recipes and measurements), budgeting (money and expenses), and measuring (distances, lengths, weights). A quick review of fractions and integers will help students recall prior knowledge before they learn to place these numbers on a number line, which is a visual tool that helps in understanding the concept of rational numbers better. Encourage students to think of examples from their daily lives that involve rational numbers.

Understanding Rational Numbers – Definition of rational numbers – A number is rational if it can be expressed as a fraction a/b, where a and b are integers and b is not zero. – Examples vs. non-examples – Examples: 1/2, 0.75 (3/4); Non-examples: À, 2 – Rational numbers as fractions – Every rational number can be written as a fraction, even if it’s a whole number or a decimal. – Identifying rational numbers | Introduce the concept of rational numbers by defining them as any number that can be expressed as the quotient or fraction of two integers, with the denominator not being zero. Provide clear examples of rational numbers, such as 1/2 or 0.75, and contrast them with non-examples like pi (À) or the square root of 2, which are irrational. Emphasize that all whole numbers, decimals that terminate or repeat can also be written as fractions, thus classifying them as rational. Encourage students to practice identifying rational numbers from various representations and to convert decimals to fractions as part of understanding their rationality.

Rational Numbers on the Number Line – Locating rational numbers – Use a number line to position rationals accurately. – Infinite rationals between integers – Between any two integers, there’s an endless set of rational numbers. – Placing fractions on the line – Find the correct interval for a fraction, then mark its position. – Placing decimals on the line – Convert decimals to fractions if needed, then plot. | This slide introduces students to the concept of rational numbers and how to represent them on a number line. Emphasize that rational numbers include all integers, fractions, and decimals that can be expressed as a fraction. Highlight the fact that there are infinitely many rational numbers between any two integers, which helps students understand the density property of rational numbers. Provide clear instructions on how to place fractions by identifying the correct interval between integers and then marking the precise location. For decimals, teach students how to convert them to fractions or to understand their value as a part of a whole when placing them on the number line. Use examples to illustrate these concepts and encourage students to practice with different rational numbers.

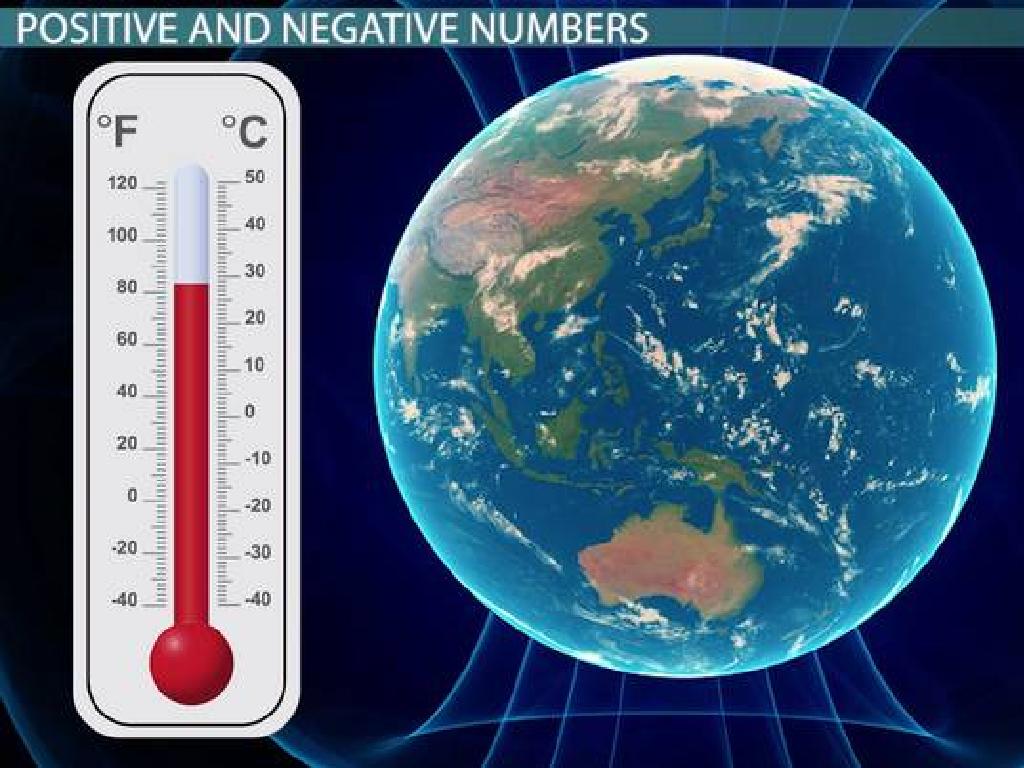
Positive and Negative Rational Numbers – Rational numbers: positive or negative – Opposites on the number line – Opposite numbers are the same distance from zero, in opposite directions. – Examples of negative rational numbers – Temperatures below zero, bank account overdrafts. – Placing rational numbers on a line – Visualize placing 3/4, -3/4 on a number line. | This slide introduces students to the concept that rational numbers can be both positive and negative, and how they are represented on a number line. Emphasize the understanding of opposites; for example, +5 and -5 are opposites and equidistant from zero on the number line. Provide real-life contexts to help students grasp negative rational numbers, such as temperatures below freezing or owing money (debt). Finally, demonstrate how to correctly place positive and negative rational numbers on a number line, ensuring students understand that each point on the line corresponds to a unique rational number. Encourage students to practice with different examples and to visualize the number line as a tool for understanding the relationship between numbers.

Equivalent Rational Numbers – Same number, different forms – 1/2 is the same as 2/4 or 0.5 – Simplify fractions for equivalents – Reduce 4/8 to 1/2 by dividing by 4 – Convert decimals to fractions – 0.75 becomes 3/4 by understanding place values – Convert fractions to decimals – 3/4 is 0.75 by dividing numerator by denominator | This slide introduces students to the concept of equivalent rational numbers, emphasizing that a single rational number can be represented in various forms. Start by explaining that fractions can be simplified by dividing the numerator and the denominator by the same number to find an equivalent fraction. Then, demonstrate how to convert decimals to fractions by placing the decimal over the appropriate place value (tenths, hundredths, etc.) and simplifying if necessary. Conversely, show how to convert fractions to decimals by dividing the numerator by the denominator. Use visual aids like number lines or pie charts to help students visualize these concepts. Encourage students to practice with different numbers to gain confidence in converting between decimals and fractions.

Ordering Rational Numbers on a Number Line – Compare and order rational numbers – Determine which numbers are larger or smaller – Use a number line for values – Visualize and find numbers’ positions – Class activity: Arrange numbers – List: 1/2, 3/4, -1/3, 0, 2/5 – Understanding ascending order | This slide introduces the concept of ordering rational numbers using a number line, which is a visual tool that helps students understand the relative positions of numbers. Start by explaining how to compare rational numbers by looking at their positions on the number line. The class activity involves arranging a given set of numbers in ascending order, which will help students apply their understanding of number lines and rational numbers. Provide guidance on how to place positive and negative fractions, as well as whole numbers, on the number line. Encourage students to discuss their reasoning with peers. For the activity, consider having different sets of numbers for groups to ensure all students are engaged and challenged.

Class Activity: Number Line Challenge – Place rational numbers on a number line – Work in pairs on the worksheet – Discuss the placement rationale – Why did you place the number there? – Share results with the class | This activity is designed to help students understand the concept of rational numbers and their representation on a number line. Provide each pair with a worksheet that includes a number line and a list of rational numbers to place on it. Encourage students to discuss with their partners why each number belongs in a specific spot based on its value. After completing the worksheet, have each pair share their number line with the class and explain their reasoning for the placement of the numbers. This will foster a collaborative learning environment and enhance their understanding of rational numbers. Possible variations of the activity could include using different ranges on the number line, including negative rational numbers, or having students create their own number lines with their own sets of rational numbers.

Rational Numbers: Conclusion & Homework – Recap: Rational numbers on lines – Remember, rational numbers can be plotted on a number line. – Importance of rational numbers – They are essential for math concepts like fractions, division, and real-world applications. – Homework: Find 5 home rational numbers – Look for examples like measuring cups, clocks, or money. – Be ready to discuss your findings | As we wrap up today’s lesson on rational numbers, it’s crucial for students to understand that these numbers are everywhere, not just in the classroom. They are used in daily life, such as in cooking or telling time. For homework, students should find five examples of rational numbers at home, like the fraction of a gallon of milk or the decimal on a digital thermometer. This will help them relate classroom learning to real-world scenarios. Encourage them to be prepared to explain how they identified the numbers and why they are rational. This exercise will reinforce their understanding and help them see the practical application of rational numbers.