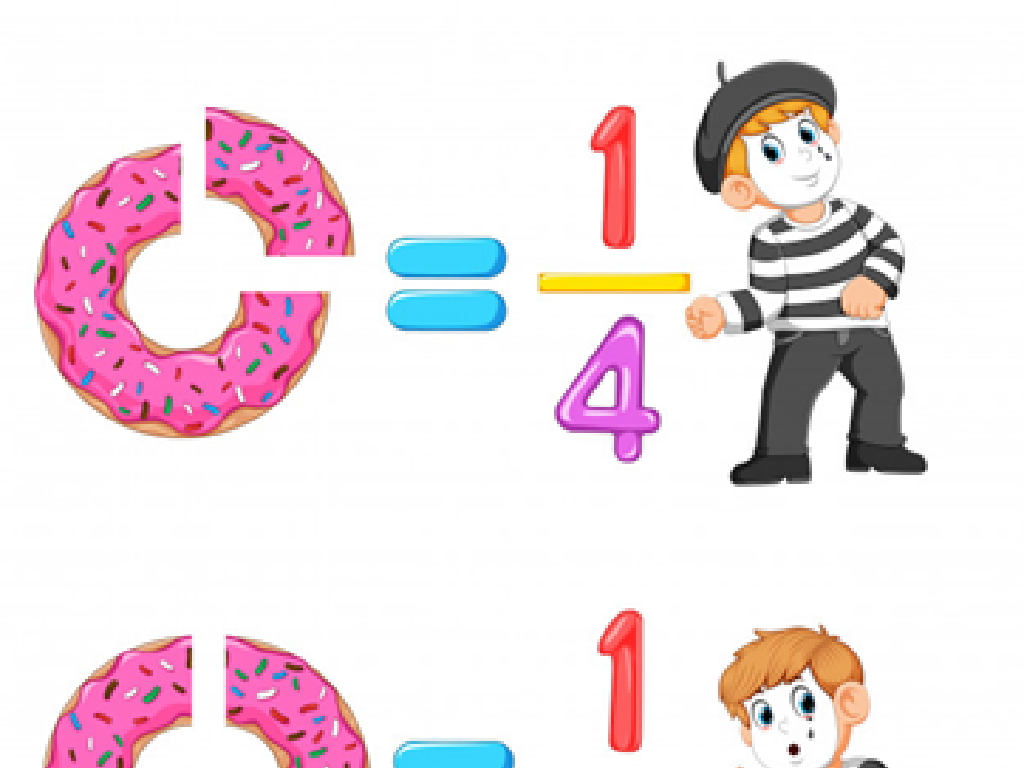Your next great Lessn starts here.

Introduction to Rational Numbers – Understanding Rational Numbers – Definition of a Rational Number – A number expressible as a fraction a/b, where a and b are integers, and b is not zero. – Examples of Rational Numbers – Examples: 1/2, 3/4, 0.75, 8 – Non-examples of Rational Numbers – Non-examples: Pi (À), square root of 2 | This slide introduces the concept of rational numbers, which is foundational for understanding how to convert decimals to fractions or mixed numbers. Start by explaining that rational numbers include all the numbers that can be written as a fraction, where both the numerator and the denominator are integers, and the denominator is not zero. Provide clear examples of rational numbers, including both fractions and decimals, and contrast these with non-examples like irrational numbers, which cannot be expressed as a simple fraction. Emphasize that understanding rational numbers is crucial for performing conversions between decimals and fractions, which is a skill they will frequently use in mathematics.

Decimals and Fractions: Understanding the Relationship – Define decimals – Decimals are numbers with a decimal point, representing parts of a whole. – Define fractions – Fractions consist of a numerator over a denominator, indicating parts of a whole. – Relate decimals to fractions – Every decimal has an equivalent fraction form. – Conversion process – To convert, place the decimal over 1 and multiply by 10 for each decimal place. | This slide introduces the basic concepts of decimals and fractions, setting the stage for understanding their relationship. Decimals are a way to express numbers that are not whole, using a decimal point. Fractions also represent parts of a whole, with a numerator and denominator. The key takeaway is that decimals and fractions are two sides of the same coin and can be converted into one another. For example, 0.75 can be expressed as 75/100, which simplifies to 3/4. Encourage students to practice converting between decimals and fractions with various examples, reinforcing the concept that they are interconnected representations of rational numbers.

Converting Decimals to Fractions – Decimal over one as a fraction – Multiply to remove decimal point – If decimal is 0.75, multiply by 100 to get 75/100 – Convert to a whole number – 75/100 is a whole number fraction – Simplify the resulting fraction – Reduce 75/100 to its simplest form, 3/4 | This slide outlines the process of converting decimals to fractions, a key concept in understanding rational numbers. Start by writing the decimal over one to create a fraction. Then, multiply both the numerator (the decimal) and the denominator (1) by 10 repeatedly until the numerator becomes a whole number. For example, to convert 0.75 to a fraction, multiply by 100 to get 75/100. Once you have a whole number numerator, simplify the fraction by finding the greatest common divisor for the numerator and denominator and dividing both by that number. In our example, 75 and 100 can both be divided by 25, resulting in the simplified fraction 3/4. Encourage students to practice this method with different decimals to become comfortable with the process.

Converting Decimals to Mixed Numbers – Identify whole and decimal parts – Separate the number left of the decimal from the right – Convert decimal to fraction – Use place value to write the fraction part – Combine whole number and fraction – Write as a sum of the whole number and fraction | This slide is aimed at teaching students the process of converting decimals to mixed numbers. Start by identifying the digits to the left of the decimal point as the whole number part. Then, look at the digits to the right of the decimal point, which represent the decimal part. Convert this decimal part into a fraction by considering its place value (tenths, hundredths, etc.). Finally, combine the whole number with the newly converted fraction to form a mixed number. For example, for the decimal 3.25, the whole number part is 3, and the decimal part is .25, which is 25/100 or simplified to 1/4, resulting in the mixed number 3 1/4. Encourage students to practice with different decimals to gain confidence in this conversion process.

Converting Decimals to Fractions & Mixed Numbers – Convert 0.75 to a fraction – 0.75 equals 75/100, which simplifies to 3/4 – Convert 1.25 to a mixed number – 1.25 equals 1 25/100, which simplifies to 1 1/4 – Step-by-step class walkthrough – Practice and apply concepts | This slide is aimed at providing practice examples for the class to work through together. Start by converting the decimal 0.75 to a fraction. Explain that the decimal places determine the denominator (in this case, 100), and then simplify the fraction to its lowest terms. Next, demonstrate converting the decimal 1.25 to a mixed number, emphasizing the whole number part and the fractional part that comes from the decimal. Walk through these examples step by step with the class, ensuring that students understand each part of the process. After the walkthrough, encourage students to practice with additional examples and apply the concepts learned to ensure mastery of converting decimals to fractions and mixed numbers.

Class Activity: Decimal to Fraction Conversion – Convert 0.5 to a fraction – 0.5 equals 1/2 – Turn 2.08 into a mixed number – 2.08 equals 2 2/25 – Change 3.75 to a mixed number – 3.75 equals 3 3/4 – Rewrite 0.125 as a fraction – 0.125 equals 1/8 | This activity is designed to help students practice converting decimals to fractions and mixed numbers. Students will pair up to encourage collaboration and peer learning. Each pair will work on converting the given decimals. After completing the task, we will review the answers as a class to ensure understanding and correct any misconceptions. Possible variations for different pairs could include converting decimals from their textbooks, creating their own decimals to convert, or using dice to generate random decimals for conversion. This will help cater to different learning paces and styles.

Homework: Decimals to Fractions Conversion – Convert decimals to fractions – Change decimal numbers into fraction form – Complete the conversion worksheet – Solve extra problems to master the concept – Review for the upcoming quiz – Study rational numbers for the quiz – Practice with mixed numbers – Also convert decimals to mixed numbers | This homework assignment is designed to reinforce the students’ understanding of converting decimals to fractions and mixed numbers, which is a key skill in working with rational numbers. The worksheet provides additional practice problems to ensure mastery of the concept. Encourage students to review their class notes and textbook examples to prepare for the quiz on rational numbers. Remind them to pay special attention to the place value of decimals when converting to fractions and to check their work for accuracy. Offer some example problems during class and discuss common mistakes to avoid.