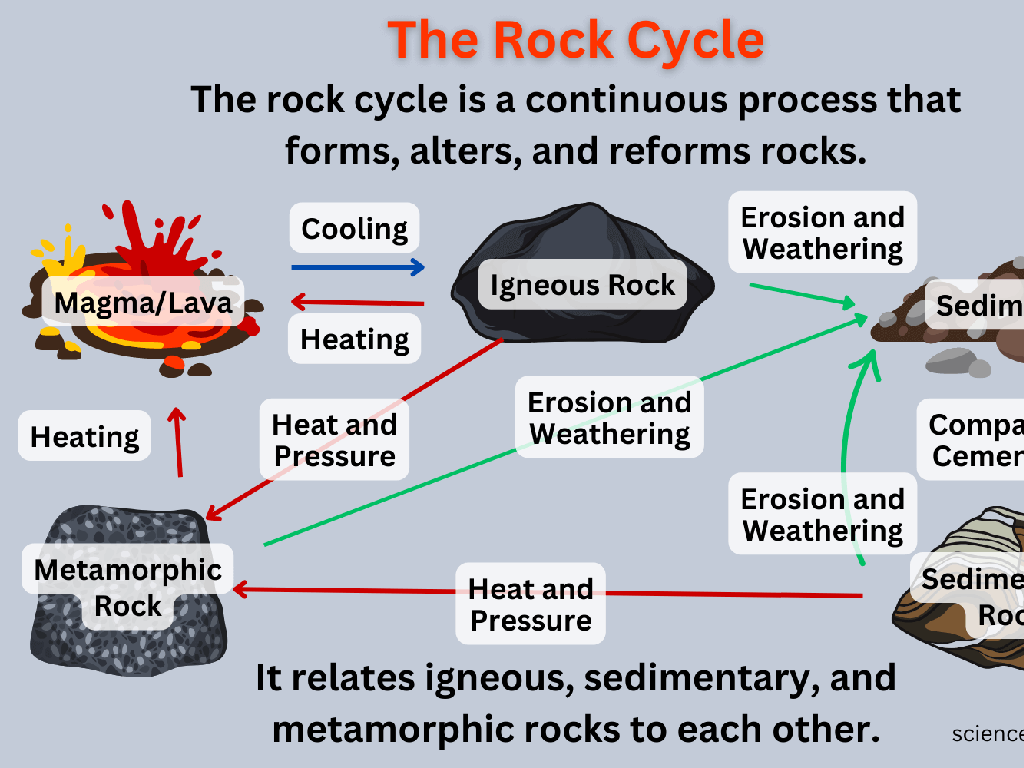
Mean, Median, Mode, And Range: Find The Missing Number
Subject: Math
Grade: Sixth grade
Topic: Statistics
Please LOG IN to download the presentation. Access is available to registered users only.
View More Content
Introduction to Statistics: Understanding the Basics
– Explore Mean, Median, Mode, Range
– Mean: average, Median: middle value, Mode: most frequent, Range: difference between highest and lowest
– Significance in math and real life
– Used in daily decisions, sports, science, and more
– Overview of lesson objectives
– Learn to calculate each measure and find missing numbers
– Engage with practical examples
|
This slide introduces the fundamental concepts of statistics that are crucial for understanding data analysis. Mean, median, mode, and range are the building blocks for summarizing and interpreting data. Emphasize the relevance of these concepts in various real-life scenarios such as calculating average grades, determining the most common score in sports, or analyzing scientific data. The lesson objectives include teaching students how to compute each measure and apply this knowledge to find missing numbers in a data set. Incorporate hands-on activities where students can practice with actual data to solidify their understanding.
Understanding Mean in Statistics
– Mean: The average of numbers
– Add all numbers and divide by the number of terms
– Calculating Mean: Add and divide
– Sum all numbers in a set, then divide by how many numbers there are
– Example: Mean of 4, 8, 6, 5, 3
– (4+8+6+5+3) / 5 = 26 / 5 = 5.2 is the mean
|
The mean is a fundamental concept in statistics, representing the average value in a set of numbers. To calculate the mean, students should add all the numbers together to find the sum, then divide by the total count of numbers to find the average. For example, with the numbers 4, 8, 6, 5, and 3, the sum is 26. Since there are 5 numbers, dividing 26 by 5 gives us the mean, which is 5.2. Ensure students understand each step and encourage them to practice with different sets of numbers to become comfortable with the process of finding the mean.
Understanding Median in Statistics
– Median: Middle value in a list
– When numbers are in order, median is the center number
– Steps to find the median
– 1. Arrange numbers 2. Find the middle value
– Example: Median of 7, 12, 3, 5, 9
– Arrange: 3, 5, 7, 9, 12. Median is 7
|
The median is a type of average that represents the middle value of a dataset. When finding the median, students should first arrange the numbers in ascending order. If there is an odd number of terms, the median is the middle number. If there is an even number of terms, the median is the average of the two middle numbers. In the example provided, once the numbers are arranged from smallest to largest (3, 5, 7, 9, 12), the median is the third number, which is 7. This concept is fundamental in understanding data distribution and is a key component of descriptive statistics. Encourage students to practice with different sets of numbers to become comfortable with finding the median.
Understanding Mode in Statistics
– Define mode in a dataset
– Mode: The value that appears most often
– Mode can be unimodal or multimodal
– Unimodal has one mode, multimodal has several
– No mode in a uniform dataset
– A set with all unique numbers has no mode
– Example: Finding the mode
– For 2, 4, 4, 5, 7, 7, 7, mode is 7
|
This slide introduces the concept of mode, which is a basic statistical measure used to find the most frequent number in a dataset. It’s important to explain that a dataset may have one mode (unimodal), more than one mode (multimodal), or no mode at all if all numbers are different. Use the example provided to show how to find the mode in a simple dataset. Encourage students to practice with different sets of numbers to identify the mode. This will help them understand how mode can give insights into the most common or popular items in a dataset, such as the most common shoe size in a class or the most popular lunch choice in the cafeteria.
Understanding Range in Statistics
– Range: highest minus lowest
– The range is 22 – 8 in our example.
– Calculating range step-by-step
– First, identify the smallest and largest numbers. Next, subtract the smallest from the largest.
– Example: Range of given numbers
– For 15, 22, 8, 19, 13, the range is 22 – 8 = 14.
|
The concept of range is a fundamental statistical measure that indicates the spread of a set of numbers. It’s calculated by subtracting the smallest number from the largest. When teaching this concept, ensure that students can identify the highest and lowest values in a data set. Use the example provided to walk through the process step by step. Have students practice with additional sets of numbers to solidify their understanding. Encourage them to explain the process in their own words to assess comprehension.
Finding the Missing Number Using Statistics
– Understanding mean, median, mode, range
– Mean: average, Median: middle value, Mode: most frequent, Range: difference between highest and lowest
– Solving problems with these measures
– Use these to determine unknown values in a data set
– Example: Known mean, find missing number
– If the mean of 5 numbers is 10 and the sum of 4 numbers is 38, what is the 5th number?
– Practice with different data sets
|
This slide introduces the concept of using statistical measures to find missing numbers in a data set. Students should understand that the mean is the average of all numbers, the median is the middle value when numbers are in order, the mode is the most frequently occurring number, and the range is the difference between the highest and lowest numbers. By knowing any of these measures, students can work backwards to find a missing number. For example, if they know the mean and the sum of the other numbers, they can multiply the mean by the total number of values to find the sum of all numbers, and then subtract the sum of known numbers to find the missing one. Encourage students to practice with different sets of data to become comfortable with these concepts.
Class Activity: Let’s Find the Missing Number!
– Group activity with number sets
– Calculate mean to find missing number
– Add all numbers, divide by total count
– Calculate median for missing number
– Order numbers, find middle value
– Discuss findings with the class
|
This interactive group activity is designed to help students apply their knowledge of statistical measures to solve problems. Each group will receive a set of numbers with one number missing. They must work together to calculate the mean, median, mode, and range, using these calculations to deduce the missing number. Afterward, they will present their methodology and findings to the class. For the teacher: Prepare sets of numbers in advance, ensuring that each set has a clear mean, median, mode, and range. Consider creating different difficulty levels for each group. Encourage students to explain their thought process during the sharing session, fostering a collaborative learning environment.
Conclusion: Understanding Measures of Central Tendency
– Recap: Mean, Median, Mode, Range
– Mean: average, Median: middle, Mode: most frequent, Range: difference between highest and lowest
– Activity Reflection
– How did we apply these concepts to find the missing number?
– Open Q&A Session
– Share Observations
– Did anyone notice patterns or have insights?
|
This slide wraps up our lesson on the measures of central tendency and their application in finding missing numbers. Begin by reviewing the definitions of mean, median, mode, and range, ensuring students understand each concept. Reflect on the class activity, discussing how these statistical tools helped solve problems. Open the floor for a question and answer session, allowing students to clarify any doubts. Finally, encourage students to share any interesting observations or patterns they noticed during the activity. This engagement helps solidify their understanding and promotes curiosity in the subject matter.
Homework: Mastering Statistics
– Practice calculating mean, median, mode, and range
– Solve the special problem with a missing number
– Find the missing value in a set to complete the sequence
– Use class lessons to find the missing number
– Apply the methods learned to deduce the missing value
– Get ready for a statistics quiz next class
|
This homework assignment is designed to reinforce today’s lesson on mean, median, mode, and range. Students are expected to solve practice problems to become proficient in calculating these statistical measures. The special problem requires students to apply their knowledge creatively to find a missing number in a set, ensuring they understand the concepts deeply. Encourage students to review their notes and practice additional problems to prepare for the upcoming quiz on statistics. Provide tips on how to approach each type of problem and remind them of the formulas and techniques discussed in class.