Your next great Lessn starts here.

Exploring Transformations: Movements in Math – What are transformations? – Transformations move or change shapes in a plane – Types: Reflections, Rotations, Translations – Reflection: flip over a line, Rotation: turn around a point, Translation: slide in any direction – Effects on shape positions – These actions change a shape’s location and orientation – Visualizing movement on a grid – Use graph paper to see how points shift during transformations | This slide introduces the concept of transformations in mathematics, focusing on how shapes can move within a plane. Transformations include reflections (flipping a shape over a line), rotations (turning a shape around a point), and translations (sliding a shape in any direction). It’s crucial to help students visualize these movements, possibly by using a grid or graph paper to track the changes in position and orientation of shapes. Encourage students to practice by drawing shapes and demonstrating each type of transformation. This will prepare them for more complex exercises and an understanding of how transformations apply to real-world situations.

Exploring Geometric Transformations – Define geometric transformation – A movement of figures in a plane – Explore types of transformations – Reflections, rotations, translations – Real-life transformation examples – Architecture, art, and technology – Understanding transformations’ impact | Begin with a clear definition of geometric transformation, emphasizing the movement of figures on a plane without altering their shape or size. Introduce the three main types of transformations: reflections (flips), rotations (turns), and translations (slides). Provide real-life examples such as reflections in water, rotational symmetry in wind turbines, and translations in the movement of vehicles. Highlight the importance of understanding transformations for practical applications in various fields like engineering, graphic design, and animation. Encourage students to visualize these concepts with hands-on activities or interactive software.

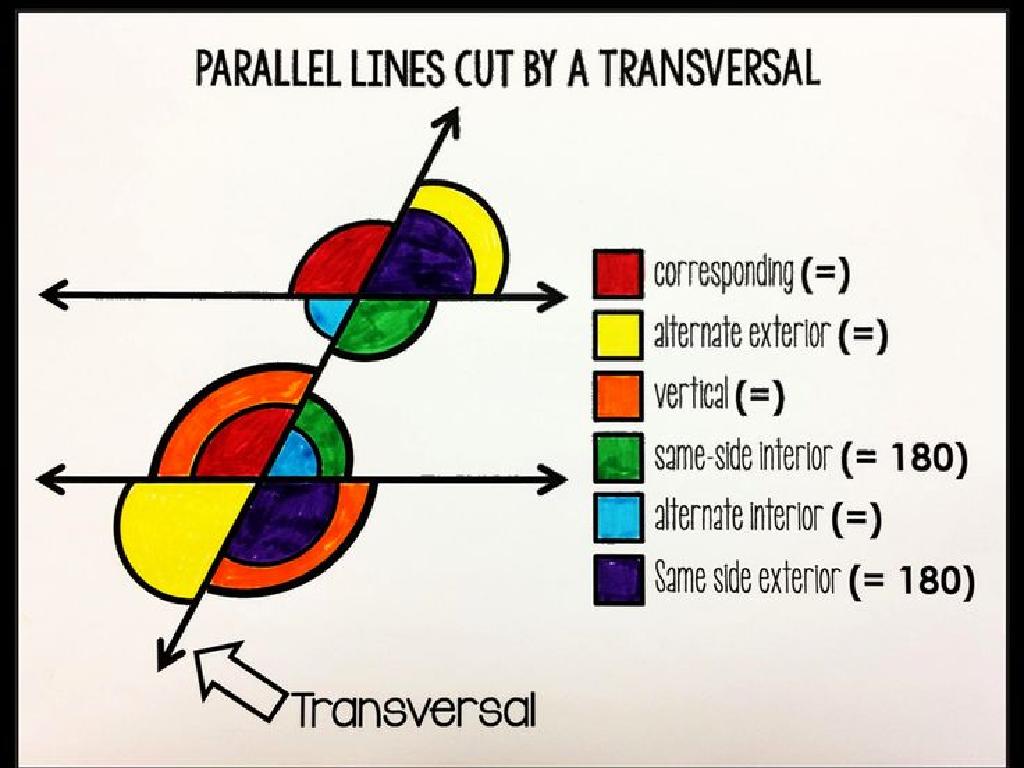
Exploring Reflections in Transformations – Define a Reflection – A flip over a line where the figure is the same distance from the line on the opposite side. – ‘Mirror Image’ concept – Imagine flipping an image over a line like looking in a mirror. – Reflections on a coordinate plane – Using the x or y-axis as the ‘mirror line’, figures are flipped across the line. – Properties of reflections | This slide introduces the concept of reflections as a type of transformation in mathematics. A reflection is essentially a ‘flip’ of a figure over a line, known as the line of reflection, where the image created is a mirror image of the original figure. It’s important for students to understand that the reflected figure is the same distance from the line of reflection as the original figure but on the opposite side. When identifying reflections on a coordinate plane, students should practice using the x-axis or y-axis as the line of reflection and observe how the coordinates of the original figure change. Emphasize that the size and shape of the figure do not change during a reflection, only its position does. Provide examples of reflections on a coordinate plane for students to analyze.

Understanding Rotations in Transformations – Define a rotation in geometry – A rotation turns a shape around a fixed point – Explore degrees of rotation – Degrees measure how far the shape turns, e.g., 90°, 180° – Rotational symmetry concept – A shape has rotational symmetry if it looks the same after a rotation less than 360° – Practice rotating shapes – Use a coordinate plane to visualize rotations | This slide introduces the concept of rotation as a type of transformation in geometry, where a figure is turned around a fixed point. Emphasize the importance of degrees in determining the extent of rotation, with common rotations being 90, 180, and 270 degrees. Discuss rotational symmetry as a property of shapes that appear identical after certain rotations. Provide examples of rotating shapes on a coordinate plane to help students visualize the concept. Encourage students to practice with different shapes and degrees of rotation to gain a better understanding. In the next class, students can work on exercises to identify and perform rotations on various figures.

Understanding Translations in Geometry – Define a Translation – A translation slides a shape to a new location without rotating or resizing it. – Translating shapes intact – When translating, the shape stays congruent and identical to the original. – Translation on coordinate plane – Use ordered pairs to slide shapes along the grid, keeping orientation. – Maintaining size and shape | This slide introduces the concept of translation in the context of geometric transformations. A translation is a type of transformation that moves every point of a shape the same distance in the same direction. It’s crucial to emphasize that translations do not alter the shape’s size, shape, or orientation. When translating shapes on a coordinate plane, students should practice using ordered pairs and vector notation to describe the movement. Encourage students to visualize translations as sliding motions and to remember that the shapes remain congruent to their pre-translation state. Provide examples of translations on a coordinate plane to solidify understanding.

Identifying Transformations in Math – Types of transformations – Reflections flip, rotations turn, translations slide – Practice with examples – Use grid paper to visualize transformations – Rules for transformations – Specific movements for each type: mirror line, degrees of rotation, direction and distance – Reflections, rotations, translations | This slide aims to help students recognize and differentiate between the three main types of transformations: reflections, rotations, and translations. Reflections act as a mirror image across a line, rotations involve turning a shape around a point by a certain number of degrees, and translations move a shape in a particular direction for a certain distance without changing its orientation. Provide practice examples on grid paper to help students visualize these concepts. Emphasize the rules that govern each transformation type, such as the line of reflection, the center and angle of rotation, and the direction and magnitude of translation. Encourage students to articulate how they identify each transformation and to use proper mathematical language when describing them.

Class Activity: Transformation Stations – Rotate, Reflect, and Translate Shapes – Follow the Activity Instructions – Each station has step-by-step guides – Work Together in Groups – Collaborate to solve transformation problems – Apply Transformation Knowledge – Use learned concepts to manipulate shapes | This interactive class activity is designed to help students apply their knowledge of geometric transformations in a collaborative setting. Set up stations around the classroom, each dedicated to a different type of transformation: rotation, reflection, and translation. Provide clear instructions at each station, guiding students on how to manipulate shapes according to the transformation type. Encourage students to work in small groups to foster teamwork and discussion. As they rotate through the stations, they should apply what they’ve learned about transformations to complete the tasks. This hands-on experience will reinforce their understanding and give them practical skills in identifying and performing reflections, rotations, and translations. Possible activities include rotating shapes on graph paper, reflecting shapes over a line of symmetry, and translating shapes by sliding them across the grid.

Class Challenge: Transformation Exploration – Understand reflections, rotations, translations – A reflection flips over a line, a rotation turns around a point, a translation slides in any direction. – Use grid paper for visualization – Grid paper helps see the movement and position of shapes after transformations. – Perform each transformation – Try reflecting a shape over a line, rotating it around a point, and translating it along the grid. – Share and discuss findings – Explain your process and compare with classmates. | This slide introduces a class activity focused on identifying and performing the three main types of transformations: reflections, rotations, and translations. Students will use grid paper to help them visualize how shapes move and change position during each type of transformation. They will then perform each transformation themselves, which will aid in their understanding of the concepts. After completing the transformations, students will share their solutions and discuss their understanding with the class. This activity will help solidify their knowledge through practical application and peer learning. For the teacher: Prepare different transformation tasks for students to perform, ensure they understand the axis and point of rotation, and guide them in using the correct notation for transformations.

Transformations: Review & Homework – Recap key transformation points – Review reflections, rotations, translations – Q&A session – Homework: Transformation worksheet – Complete the provided worksheet – Practice with reflections, rotations, translations – Apply today’s lesson to solve problems | This slide aims to consolidate the students’ understanding of geometric transformations covered in today’s lesson. Begin with a brief review of the key points, emphasizing the characteristics of reflections (flips), rotations (turns), and translations (slides). Open the floor for a Q&A session to address any uncertainties the students may have. For homework, assign a worksheet that includes problems on all three types of transformations, ensuring that the tasks are varied and reinforce the concepts learned. Encourage students to apply the rules and processes discussed in class to complete the worksheet. The next class will start with a discussion of the homework to correct any misconceptions and to celebrate successes.