Your next great Lessn starts here.

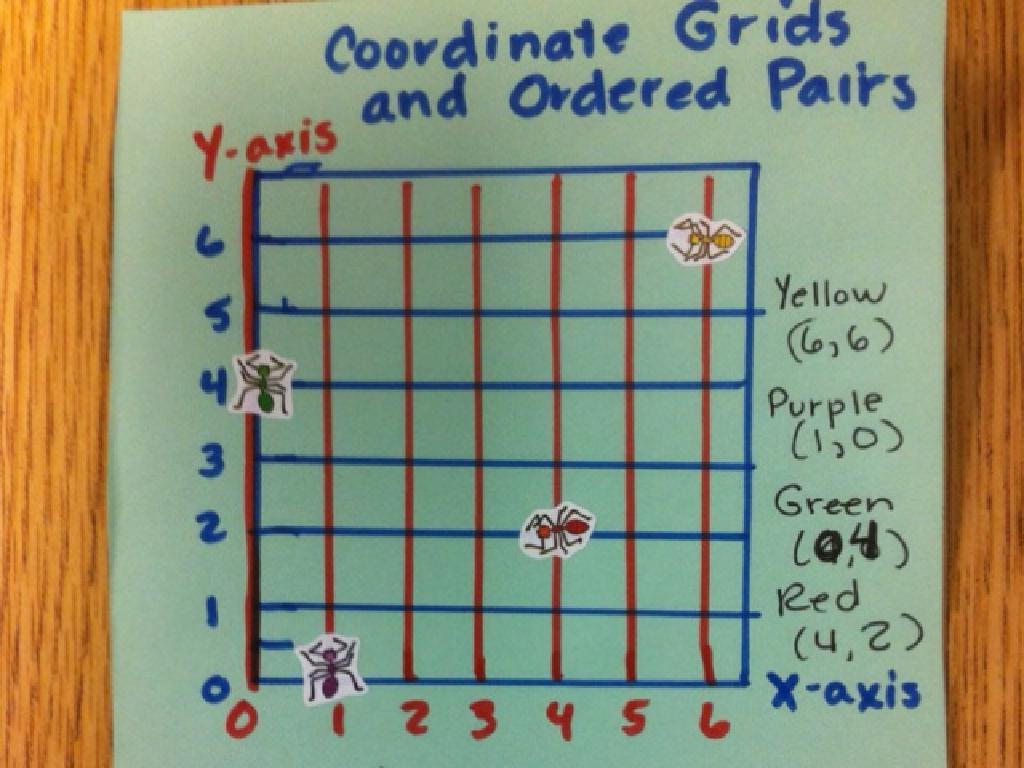
Reflections over the x- and y-axes – What are transformations? – Reflections: Flipping over axes – A reflection is like a mirror image across an axis – How to find reflected coordinates – To reflect over x-axis, invert y-coordinate. Over y-axis, invert x-coordinate – Practice with coordinate reflections – We’ll reflect points (a, b) over both axes and find new coordinates | Begin with a brief explanation of transformations as operations that alter the position of shapes on a coordinate plane. Today, we focus on reflections, which can be thought of as ‘flipping’ a shape over a line, such as the x- or y-axis. The learning objective is for students to understand how to find the coordinates of a point after it has been reflected over the x- or y-axis. Emphasize that when reflecting over the x-axis, the y-coordinate changes sign, and when reflecting over the y-axis, the x-coordinate changes sign. Provide several examples on the board and encourage students to work through practice problems to solidify their understanding.

Understanding Reflections in Math – Reflection: flipping a shape – A shape is flipped over a line, keeping size and shape consistent. – Mirror image concept – Like looking in a mirror, everything is reversed! – Reflection over the x-axis – Flipping a shape over the x-axis inverts its y-coordinates. – Reflection over the y-axis – Flipping a shape over the y-axis inverts its x-coordinates. | This slide introduces the concept of reflection in the context of mathematical transformations. Reflection is akin to creating a mirror image of a shape, which involves flipping the shape over a specific line called the axis of reflection. When reflecting over the x-axis, the y-coordinates of the shape’s points change sign, while reflecting over the y-axis changes the sign of the x-coordinates. It’s crucial to emphasize that the size and shape of the figure remain unchanged during reflection. Use visual aids to demonstrate this concept, and consider having students practice with simple shapes on graph paper to solidify their understanding.

Reflections Over the X-axis – Reflecting over the x-axis – Y-coordinate changes sign – For point (x, y), reflection is (x, -y) – Example: Point A to A’ – Original A (3, 4) becomes A’ (3, -4) after reflection – X-coordinate remains constant | When a point is reflected over the x-axis, its position relative to the x-axis is flipped. This means that the y-coordinate of the point changes its sign, while the x-coordinate stays the same. For example, if we have a point A with coordinates (3, 4), reflecting it over the x-axis will result in point A’ with coordinates (3, -4). It’s crucial for students to understand that the x-axis acts like a mirror, and the reflection changes the vertical position of the point. Have students practice with multiple points to solidify their understanding of reflections over the x-axis.

Reflections Over the Y-axis – Reflecting over the y-axis – X-coordinate changes sign – The x-value becomes its opposite, while y-value stays the same – Example: Point B to B’ – Original B (5, -2), Reflected B’ (-5, -2) – Y-coordinate remains unchanged | This slide introduces the concept of reflection over the y-axis in coordinate geometry. When a point is reflected over the y-axis, its x-coordinate is negated, meaning the sign in front of the x-coordinate is reversed. The y-coordinate remains the same because the point is still the same distance from the x-axis. It’s crucial to emphasize that the reflection is a ‘mirror image’ across the y-axis. Use the example of Point B to illustrate this transformation clearly. Encourage students to practice with additional points and to visualize the reflection by actually drawing the points and their reflections on graph paper.

Reflections Over Axes: Practice Example – Reflect point C (2, 3) over axes – Over x-axis: C’ (2, -3) – Invert the y-coordinate for x-axis reflection – Over y-axis: C” (-2, 3) – Invert the x-coordinate for y-axis reflection – Observe coordinate changes | This slide provides a practical example to help students understand reflections over the x- and y-axes. Start by plotting point C (2, 3) on the coordinate plane. To reflect over the x-axis, keep the x-coordinate the same and invert the sign of the y-coordinate, resulting in point C’ (2, -3). For a reflection over the y-axis, keep the y-coordinate the same and invert the sign of the x-coordinate, resulting in point C” (-2, 3). Encourage students to visualize the point flipping over the respective axis. This exercise will solidify their understanding of how coordinates change during reflections and prepare them for more complex transformation tasks.

Your Turn to Reflect! – Activity: Reflect given points – Work in pairs for reflection – Reflect points over x-axis – If point is (a, b), its reflection is (a, -b) – Reflect points over y-axis – If point is (a, b), its reflection is (-a, b) | This slide introduces an interactive class activity where students will work in pairs to find the reflections of given points across the x-axis and y-axis. Provide students with a set of points and graph paper or a digital graphing tool. For the x-axis reflection, guide them to understand that the y-coordinate changes sign while the x-coordinate remains the same. For the y-axis reflection, the x-coordinate changes sign while the y-coordinate remains the same. After completing the activity, have each pair share their reflected points with the class to ensure understanding and correct application of the reflection process. This activity will help solidify their understanding of reflections in a coordinate plane.

Class Activity: Reflection Art – Create artwork with reflections – Choose a shape to reflect – Reflect your chosen shape over the x-axis and y-axis – Plot shapes on graph paper – Ensure original and reflections are plotted accurately – Present your art to the class | This activity is designed to help students understand reflections in a fun and creative way. Students will select a shape and then draw its reflection across both the x-axis and y-axis, using graph paper for precision. Encourage them to be creative with their shapes and use different colors for the original and reflected shapes to enhance the visual effect. Possible shapes include triangles, squares, or any polygon. After completing their artwork, students will present to the class, explaining the process of reflection they used. This will reinforce their understanding and provide an opportunity for peer learning. For students who finish early, challenge them to reflect shapes over both axes simultaneously or to create a pattern using multiple reflected shapes.

Reflections & Homework Assignment – Recap on shape reflections – Flipping a shape over a line is called reflection – Rule for reflecting over axes – Over y-axis: x-coord changes; over x-axis: y-coord changes – Homework: Reflection worksheet – Prepare for class discussion – Review your answers and think about the reflection rules | This slide concludes the lesson on reflections over the x- and y-axes. Start by recapping that reflections involve flipping a shape over a line, which can be the x-axis or y-axis. Emphasize the rule that when a shape is reflected over the y-axis, its x-coordinates change sign, and when reflected over the x-axis, its y-coordinates change sign. Assign the worksheet on reflections for homework, and ask students to complete it before the next class. Encourage them to be ready to discuss their answers and how they applied the rules of reflection to find the coordinates of the reflected shapes. This will help reinforce their understanding and prepare them for more complex transformation tasks.