Your next great Lessn starts here.

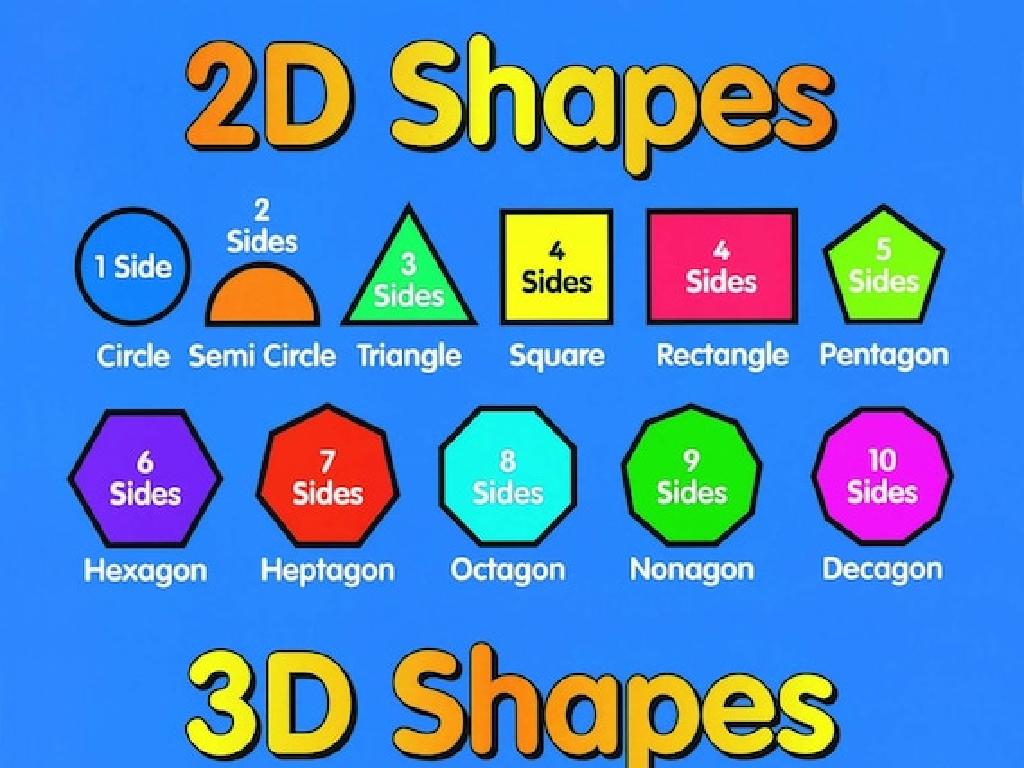
Exploring Triangle Inequality – What are two-dimensional figures? – Properties of shapes – Shapes have sides, angles, and can be congruent or similar – Triangle Inequality Theorem – The sum of lengths of any two sides of a triangle must be greater than the length of the third side – Applying the theorem – Use the theorem to determine if three lengths can form a triangle | This slide introduces students to the concept of two-dimensional figures, focusing on their properties and leading to the Triangle Inequality Theorem. Begin by explaining that two-dimensional figures are flat and have only length and width. Discuss various properties such as sides, angles, and the concepts of congruence and similarity. Introduce the Triangle Inequality Theorem, ensuring students understand that it is a rule that helps us know if three given lengths can make a triangle. Emphasize that for any triangle, the sum of the lengths of any two sides must be greater than the length of the remaining side. Provide examples and encourage students to apply the theorem to different sets of lengths to conclude if they can form a triangle.

Exploring Triangles – Define a triangle – A shape with three sides and three angles – Classify triangles by sides – Equilateral, isosceles, and scalene – Classify triangles by angles – Acute, right, and obtuse triangles – Understand triangle properties – Triangle inequality: sum of any two sides > third side | Begin with the definition of a triangle, emphasizing its three-sided nature. Move on to classification by sides: equilateral (all sides equal), isosceles (two sides equal), and scalene (no sides equal). Then, classify by angles: acute (all angles 90°). Discuss properties, focusing on the triangle inequality theorem, which states that the sum of the lengths of any two sides of a triangle must be greater than the length of the remaining side. This is crucial for understanding what makes a shape a triangle and will be foundational for future geometry lessons.

Understanding Triangle Inequality – Triangle Inequality Theorem – The sum of any two sides must be greater than the third side. – Valid vs. Invalid Triangles – Triangles with sides 3, 4, 8 are not possible. – Sum of Two Sides and the Third – If two sides are 5 and 3, the third side must be less than 8. – Practical Implications | The Triangle Inequality Theorem is a fundamental concept in geometry that states the sum of the lengths of any two sides of a triangle must be greater than the length of the remaining side. This slide will explain the theorem and provide examples to illustrate valid and invalid triangles, helping students understand why a triangle cannot exist if the sum of any two sides is less than the third side. Use real-life examples, such as sticks or straws, to demonstrate how trying to form a triangle with invalid side lengths is impossible. This concept is crucial for students to grasp as it lays the groundwork for understanding the properties of triangles and is widely applicable in various mathematical problems.

Exploring Triangle Inequality – Visual examples of triangle inequality – See how different side lengths affect triangle formation – Interactive questioning session – Can sides of length 3, 4, and 8 form a triangle? – Group discussion on triangle formation – Share thoughts on what makes a triangle possible – Predicting triangle possibility | This slide is designed to engage students with the concept of triangle inequality through visual aids and interactive participation. Begin by showing examples of triangles with various side lengths to illustrate when the triangle inequality holds true. Move on to an interactive questioning session where students predict if certain sets of side lengths can form a triangle, fostering critical thinking. Encourage group discussions to allow students to share their reasoning and solidify their understanding of the triangle inequality theorem. The goal is to help students grasp that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side. This understanding is crucial for their further studies in geometry.

Triangle Inequality in Real Life – Triangle inequality in daily life – Wood piece triangle example – Can three pieces of wood form a triangle? The sum of lengths of any two sides must be greater than the third side. – Triangle inequality in construction – It’s crucial for determining if materials can form a stable structure. – Ensuring safety and stability – It helps in designing structures that can withstand stress without collapsing. | This slide aims to show students the practical application of the triangle inequality theorem beyond the classroom. By understanding that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side, students can relate this concept to real-world scenarios such as construction. For example, when determining if a piece of wood can form a stable triangle frame, the triangle inequality theorem is used to ensure that the pieces fit together properly. Emphasize the importance of this theorem in construction, where it is used to design buildings and bridges, ensuring they are safe and stable. Encourage students to think of other areas where this principle might apply, such as in art, design, or even in nature.

Class Activity: Create Your Triangle! – Use straws to form triangles – Explore various straw combinations – Try long, medium, and short straws – Record working and non-working sets – Note which lengths can’t form a triangle – Share findings with the class | This hands-on activity is designed to help students understand the triangle inequality theorem, which states that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side. Provide students with straws of different lengths and instruct them to attempt to form triangles. Encourage them to explore and record their observations on which combinations of straws are successful in forming a triangle and which are not. This will lead them to discover that not all sets of three lengths can create a triangle. After the activity, have students share their findings with the class to reinforce the concept. Possible variations for different students could include using different materials, working in pairs, or challenging them to find the maximum or minimum length of the third side given two sides.

Conclusion & Homework: Triangle Inequality – Recap Triangle Inequality Theorem – Summarize the theorem’s key points – Engage in Q&A for clarity – Address any student uncertainties – Homework: Test Triangle Inequality – Find objects forming a triangle – Measure sides of home objects – Ensure the sum of two sides is greater than the third | As we conclude today’s lesson on the Triangle Inequality Theorem, let’s ensure we understand that in any triangle, the sum of the lengths of any two sides must be greater than the length of the remaining side. Encourage students to ask questions to clarify their understanding. For homework, students are to find three objects at home that form a triangle and measure their sides to verify the theorem. This practical activity will help solidify their understanding by applying the theorem to real-world objects. Remind students to write down their measurements and whether or not they satisfy the triangle inequality. They should be prepared to discuss their findings in the next class.