Your next great Lessn starts here.

Central Angles of Circles – Explore the circle’s properties – A circle is a round shape with all points equidistant from the center. – Define a central angle – A central angle has its vertex at the circle’s center and sides are radii. – Measure central angles – Use a protractor to measure the angle between the radii. – Central angles in daily life – Examples: Clock hands at different hours, slices of pie. | This slide introduces students to the concept of central angles within the context of circles, a fundamental aspect of two-dimensional figures in geometry. Begin by discussing the basic properties of a circle, emphasizing the uniform distance from the center to any point on the circle’s edge. Then, define a central angle as one whose sides are radii of the circle and whose vertex is the center of the circle. Demonstrate how to measure a central angle using a protractor, and relate the concept to real-life examples such as the angles formed by clock hands or the slices of a pie. Encourage students to think of other examples where they might encounter central angles in their daily lives.

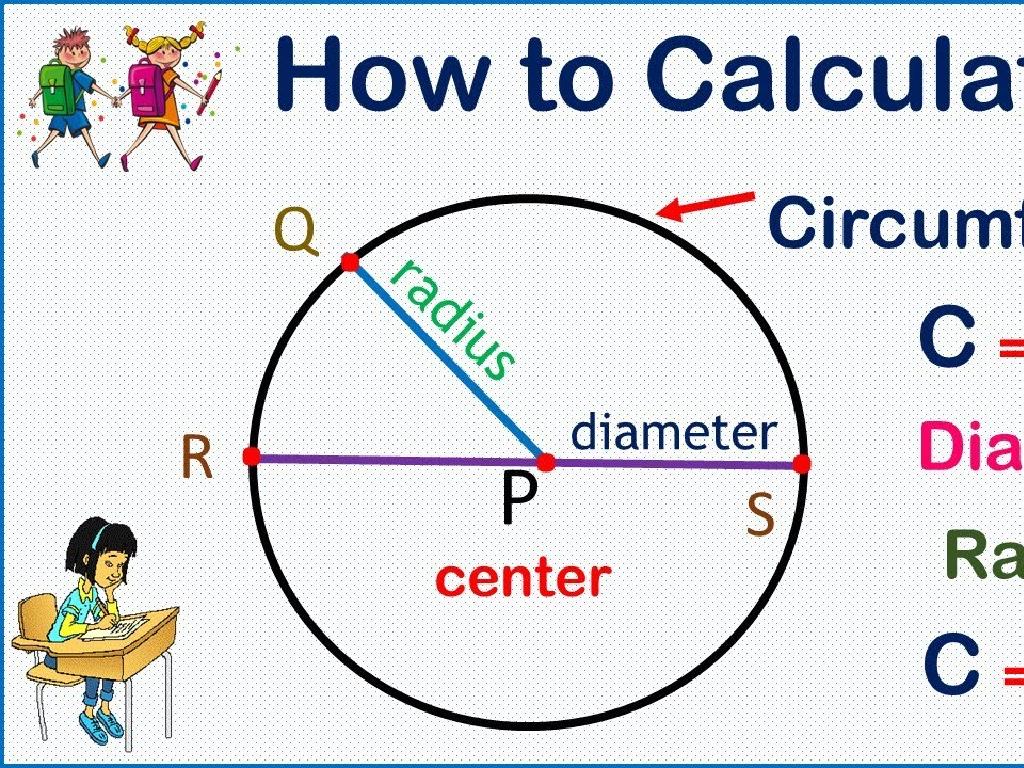
Understanding Circles – Circle: a round 2D shape – All points are equidistant from the center – Key parts: center, radius, diameter – Radius: line from center to edge, Diameter: line through center, connecting edges – Circumference: the perimeter – The distance around the circle – A circle’s total degrees: 360 – Every circle, regardless of size, has 360 degrees | Introduce the concept of a circle by defining it as a two-dimensional shape where all points are equally distant from the center. Highlight the key parts of a circle: the center, radius, diameter, and circumference. Explain that the radius is a line from the center to the edge of the circle, while the diameter is a line that passes through the center and touches two points on the edge. The circumference is the total distance around the circle. Emphasize that regardless of the size of the circle, the total degrees around the center point is always 360. This foundational knowledge will be crucial for understanding central angles and other properties of circles.

Understanding Central Angles in Circles – Central angle’s vertex at circle’s center – The point where the angle starts is the center of the circle. – Formed by two radii of the circle – Two straight lines from the center to the circle’s edge make an angle. – Arc corresponds to angle’s measure – The arc is the curved part of the circle’s edge between the radii. – Central angle and circle’s circumference – The angle’s size determines the length of the arc on the circle’s edge. | This slide introduces the concept of central angles in circles, which is fundamental for understanding circle geometry. A central angle is defined by its vertex located at the center of the circle and its sides (radii) extending to the circumference. The arc created by the central angle is a portion of the circle’s circumference, and its length is directly related to the measure of the angle. It’s important for students to visualize this relationship and recognize how the central angle and the arc it intercepts are interconnected. Have students practice drawing central angles and identifying the associated arc to reinforce the concept.

Measuring Central Angles – Central angles in degrees – Measure with a protractor – Place the protractor’s center at the vertex – Angle equals arc measure – The arc’s length is equal to the angle’s degree – Practice measuring angles – Use various circle diagrams to measure angles | Central angles are the angles whose vertex is the center of the circle and whose sides are radii. These angles are measured in degrees using a protractor. When measuring, ensure the protractor’s center is aligned with the circle’s center and one side of the angle aligns with the zero line of the protractor. The measure of the central angle is directly equal to the measure of the intercepted arc. Provide students with practice problems that include various circle diagrams and ask them to measure the central angles using a protractor. This will help them understand the concept of central angles and how they relate to the arcs they intercept.

Exploring Central Angles in Circles – A quarter circle’s central angle – 90 degrees is the central angle for a quarter circle – A half circle’s central angle – 180 degrees is the central angle for a half circle – Example: 45-degree central angle – A pizza slice can represent a 45-degree central angle – Understanding central angles | This slide aims to provide clear examples of central angles in circles, which are angles with their vertex at the center of the circle and their sides are radii of the circle. A quarter of a circle, resembling a pizza slice or a pie piece, is a practical example of a 90-degree central angle. Similarly, a half circle, like a semicircle window, forms a 180-degree central angle. To visualize a 45-degree central angle, imagine cutting a quarter circle in half. Encourage students to draw these angles in a circle and measure them with a protractor. This will help solidify their understanding of central angles and their measurements.

Calculating Central Angles – Central angle calculation method – Use arc length to find the central angle – Central Angle formula – Central Angle = (Arc Length / Circumference) * 360 – Practice Problem – Find the angle with a 5-inch arc in a 10-inch radius circle | This slide introduces the method to calculate the central angle of a circle when the arc length is known. The central angle in degrees can be found by dividing the arc length by the circumference of the circle and then multiplying by 360. For the practice problem, guide students to first calculate the circumference of the circle using the formula C = 2 * pi * radius, which in this case would be 2 * pi * 10. Then, they can use the central angle formula to find the angle corresponding to a 5-inch arc length. This exercise will help solidify their understanding of the relationship between arc length, central angles, and the circumference of a circle.

Class Activity: Create Your Own Circle – Gather materials: paper, compass, protractor, ruler – Draw a circle and mark the center – Draw two radii to form a central angle – Use the compass to draw two straight lines from the center to the edge – Measure the angle with a protractor – Place the protractor’s center mark at the vertex and measure the angle | This hands-on activity is designed to help students understand central angles in circles. Provide each student with the necessary materials. Guide them through the steps of drawing a circle using a compass and marking the center. Next, instruct them to draw two radii from the center to the circle’s edge, creating a central angle. Show them how to use a protractor to measure the angle accurately. Once everyone has measured their angles, facilitate a class discussion where students can share their measurements and reflect on the activity. Possible variations for different students could include drawing different-sized circles, comparing central angles, or creating and measuring multiple central angles within the same circle.

Homework: Central Angle Hunt – Find real-life central angles – Sketch and measure the angles – Use a protractor to measure accurately – Note observations about each angle – Were the angles acute, obtuse, or right? – Ready to discuss findings in class | This homework assignment is designed to help students recognize and understand central angles in a practical context. Students should look for objects or settings in their environment that contain central angles, such as a slice of pizza or the hands of a clock. They should sketch these examples, use a protractor to measure the angles, and make notes about their observations, considering whether the angles are acute, obtuse, or right angles. Encourage students to be creative in their hunt and to be prepared to share their findings with the class the next day. This activity will reinforce their ability to identify and measure central angles and understand their significance in real-life objects.

Central Angles: Conclusion & Review – Recap of central angles – Central angle is formed by two radii in a circle – Central angles in geometry – They are key in understanding circle properties – Significance in real-world shapes – Used in design, architecture, and navigation – Open floor for questions | As we conclude, recap the definition of central angles and their properties. Emphasize their importance in geometry, particularly in circle theorems and calculations. Highlight how understanding central angles is crucial for real-world applications such as design, architecture, and navigation, where circular shapes are common. Finally, encourage students to ask questions to clarify any doubts, ensuring they are comfortable with the concept before moving on to the next topic.