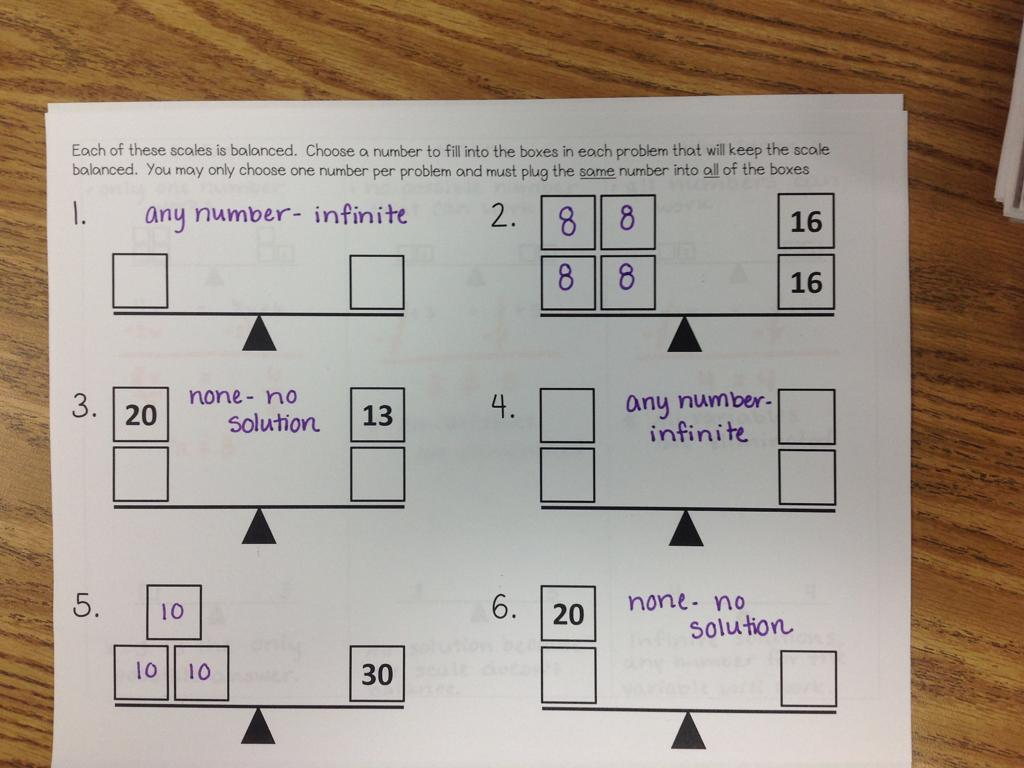
Triangle Angle-Sum Theorem
Subject: Math
Grade: Eighth grade
Topic: Two-Dimensional Figures
Please LOG IN to download the presentation. Access is available to registered users only.
View More Content
Exploring Triangles: Angle-Sum Theorem
– Basics of two-dimensional figures
– Triangles: A key geometric shape
– A polygon with three edges and vertices
– Triangle Angle-Sum Theorem
– The sum of angles in any triangle is always 180°
– Theorem application in problems
– Use theorem to find missing angles in triangles
|
This slide introduces the Triangle Angle-Sum Theorem, a fundamental concept in the study of two-dimensional figures in geometry. Begin by discussing the properties of two-dimensional figures, emphasizing that these are flat shapes with width and height but no depth. Highlight the significance of triangles in geometry, noting that they are the simplest polygon, and a building block for more complex shapes. Explain the Triangle Angle-Sum Theorem, which states that the sum of the interior angles of a triangle is always 180 degrees. This theorem is crucial for solving various geometric problems, such as finding missing angles in triangles when two angles are known. Provide examples and encourage students to apply the theorem to solve problems, reinforcing their understanding of the concept.
Exploring Triangles and Their Properties
– Define a triangle
– A shape with three sides and three angles
– Types: equilateral, isosceles, scalene
– Equilateral: all sides equal, Isosceles: two sides equal, Scalene: no sides equal
– Triangle properties
– Angles add to 180°, sides and angles have unique relationships
– Triangle Angle-Sum Theorem
– The sum of the interior angles in any triangle is always 180 degrees
|
This slide introduces students to the basic concept of triangles, an essential element in geometry. Begin with the definition of a triangle, ensuring students understand it as a three-sided polygon. Discuss the different types of triangles: equilateral with all sides and angles equal, isosceles with two sides and angles equal, and scalene with no equal sides or angles. Highlight the fundamental properties of triangles, such as the sum of interior angles always being 180 degrees, which is the Triangle Angle-Sum Theorem. Use diagrams to illustrate each type of triangle and their properties. Encourage students to draw their own examples and to remember these properties as they will be the foundation for understanding more complex geometric concepts.
Exploring Angles in a Triangle
– Understanding angles and measurement
– Angles are measured in degrees, using tools like protractors
– Identifying angles in triangles
– Triangles have interior angles at each corner
– Using a protractor to measure angles
– A protractor helps accurately measure angles in degrees
– Triangle Angle-Sum Theorem
– The sum of angles in any triangle is always 180 degrees
|
Begin the lesson by explaining what angles are and how they are measured, emphasizing the unit of degrees. Show different types of triangles (e.g., acute, obtuse, right) and point out the angles within them. Demonstrate the correct use of a protractor to measure these angles. Introduce the Triangle Angle-Sum Theorem, stating that the sum of the interior angles of any triangle is always 180 degrees. Provide examples and encourage students to measure angles of various triangles to verify the theorem. This foundational knowledge is crucial for understanding more complex geometric concepts.
Triangle Angle-Sum Theorem
– Statement of the theorem
– The sum of the angles in any triangle always add up to 180 degrees.
– Sum of interior angles = 180°
– No matter the type of triangle, the angles inside always total 180 degrees.
– Proof with an example
– Consider a triangle ABC, where angle A + angle B + angle C = 180°.
|
The Triangle Angle-Sum Theorem is a fundamental concept in geometry that states the interior angles of a triangle always add up to 180 degrees. This is true for all triangles, regardless of their shape. To prove this, we can draw a line parallel to one side of the triangle from an adjacent vertex and use alternate interior angles to show that the angles of the triangle must sum to 180 degrees. For example, if we have a triangle with angles measuring 50°, 60°, and 70°, adding them confirms the theorem as 50° + 60° + 70° = 180°. Encourage students to draw their own triangles, measure the angles, and verify that they sum to 180 degrees. This exercise not only solidifies their understanding of the theorem but also reinforces measuring skills and the concept of supplementary angles.
Applying the Triangle Angle-Sum Theorem
– Using the theorem to find angles
– Add two known angles and subtract from 180° to find the third
– Work through example problems
– Example: For a triangle with angles 50° and 60°, the missing angle is 180° – (50° + 60°) = 70°
– Learn common application mistakes
– Don’t assume a triangle is equilateral without evidence, and remember to subtract from 180°
– Practice problems for mastery
|
This slide focuses on the practical application of the Triangle Angle-Sum Theorem, which states that the sum of the angles in a triangle is always 180 degrees. Start by explaining how to use the theorem to find a missing angle when the other two angles are known. Provide example problems to demonstrate the theorem in action, ensuring to include different triangle types. Highlight common mistakes, such as assuming all triangles are equilateral or forgetting to subtract the sum of the known angles from 180 degrees. Conclude with practice problems to reinforce the concept and allow students to apply what they’ve learned.
Real-World Applications of Triangle Angle-Sum Theorem
– Importance of triangles in real life
– Triangles provide strength and stability in structures.
– Triangles in architecture and engineering
– Examples: bridges, roofs, and towers use triangular shapes.
– Triangle Angle-Sum Theorem in design
– It ensures the correct angles are used for stability.
– Construction relies on triangle principles
– Accurate measurements are crucial for safe buildings.
|
Understanding triangles is crucial because they form the basis of many structures in the real world due to their inherent strength and stability. The Triangle Angle-Sum Theorem, which states that the sum of the angles in a triangle is always 180 degrees, is particularly important in fields like architecture and engineering. This theorem is used to design and construct various structures, ensuring that they are safe and stable. For example, in bridge construction, the theorem helps in calculating precise angles for the components of the bridge, which is essential for the structure’s integrity. Encourage students to look for triangular shapes in their surroundings and consider how the Triangle Angle-Sum Theorem might have been used in their design and construction.
Class Activity: Triangle Investigators
– Form groups for activity
– Distribute triangle cut-outs
– Measure and record triangle angles
– Use protractors to measure each angle accurately
– Verify Triangle Angle-Sum Theorem
– Add angles to check if they sum up to 180°
|
In this engaging class activity, students will work in small groups to explore the Triangle Angle-Sum Theorem hands-on. Provide each group with several triangle cut-outs. Students will use protractors to measure the angles of their triangles and record these measurements. They will then add the angles together to verify that the sum is 180 degrees, thus confirming the Triangle Angle-Sum Theorem. After the activity, each group will present their findings to the class, discussing any discrepancies and reinforcing their understanding of the theorem. Possible variations of the activity could include using different types of triangles (scalene, isosceles, and equilateral), comparing theoretical versus experimental results, or exploring the theorem’s application in real-world scenarios.
Homework: Mastering Triangle Angle-Sum Theorem
– Complete the Angle-Sum worksheet
– Worksheet to reinforce today’s lesson
– Practice finding missing angles
– Use the theorem to solve for unknown angles
– Study for a quiz on 2D figures
– Quiz will cover all types of 2D figures
– Review notes and ask questions
– Ensure understanding before the quiz
|
This slide outlines the homework and further practice required for students to master the Triangle Angle-Sum Theorem. The worksheet provided will help reinforce the day’s lesson and give students practice with the theorem. Encourage them to find missing angles in various triangles using the theorem that the sum of angles in a triangle is always 180 degrees. Remind students to prepare for the upcoming quiz on two-dimensional figures by reviewing their notes, textbook, and any additional resources provided. It’s important for students to ask questions if they’re unsure about any concepts before the quiz. Differentiate homework for varying levels of student ability where necessary, and provide additional practice worksheets for those who may need them.
Triangle Angle-Sum Theorem: Conclusion
– Recap of Angle-Sum Theorem
– Sum of angles in a triangle is always 180°
– Theorem’s role in geometry
– Fundamental for solving geometric problems
– Encourage further exploration
– Investigate other two-dimensional figures
– Apply theorem in practice
|
As we conclude, remember that the Triangle Angle-Sum Theorem states that the sum of the interior angles of a triangle is always 180 degrees. This theorem is crucial in geometry as it allows us to solve for unknown angles and is applicable in various real-world contexts. Encourage students to further explore the properties of two-dimensional figures and see how this theorem plays a role in understanding their structures. Students should be prompted to apply this theorem to different types of triangles and geometric problems to solidify their understanding.