Your next great Lessn starts here.

Introduction to Two-Variable Equations – Understand equations with two variables – An equation like y = 2x + 3 has two variables, x and y. – Equations represent real-world scenarios – Think of buying fruit: y apples at x dollars each. – Writing equations from graphs – Learn to translate a graph into a table, then into an equation. – Preview of today’s lesson | Begin the lesson by explaining that equations with two variables are a way to describe relationships between different quantities. Use relatable examples, such as purchasing items, to show how these equations apply to real life. Introduce the concept of writing an equation from a graph by explaining that the graph represents a visual story of the relationship between variables, and the equation is the narrative form of that story. Today’s lesson will focus on teaching students how to interpret graphs, create tables of values, and write corresponding equations. Encourage students to think about how graphs they see in everyday life can be expressed mathematically.

Understanding Graphs in Math – Review the coordinate plane – A grid with x (horizontal) and y (vertical) axes – Graphs show variable relationships – Visual way to see how one thing affects another – Types of graphs – Bar, line, and pie charts are common examples – Interpreting graphs | Begin with a review of the coordinate plane, ensuring students recall the x-axis (horizontal) and y-axis (vertical), and how they intersect at the origin. Explain that graphs are powerful tools for showing how two variables are related, such as time and speed in a race. Provide examples of different types of graphs, including bar graphs, line graphs, and pie charts, and discuss when each type might be used. Emphasize the importance of being able to interpret these graphs, as this skill will be crucial for understanding how to write equations from graphs using a table. Encourage students to ask questions and provide their own examples of relationships that could be represented on a graph.

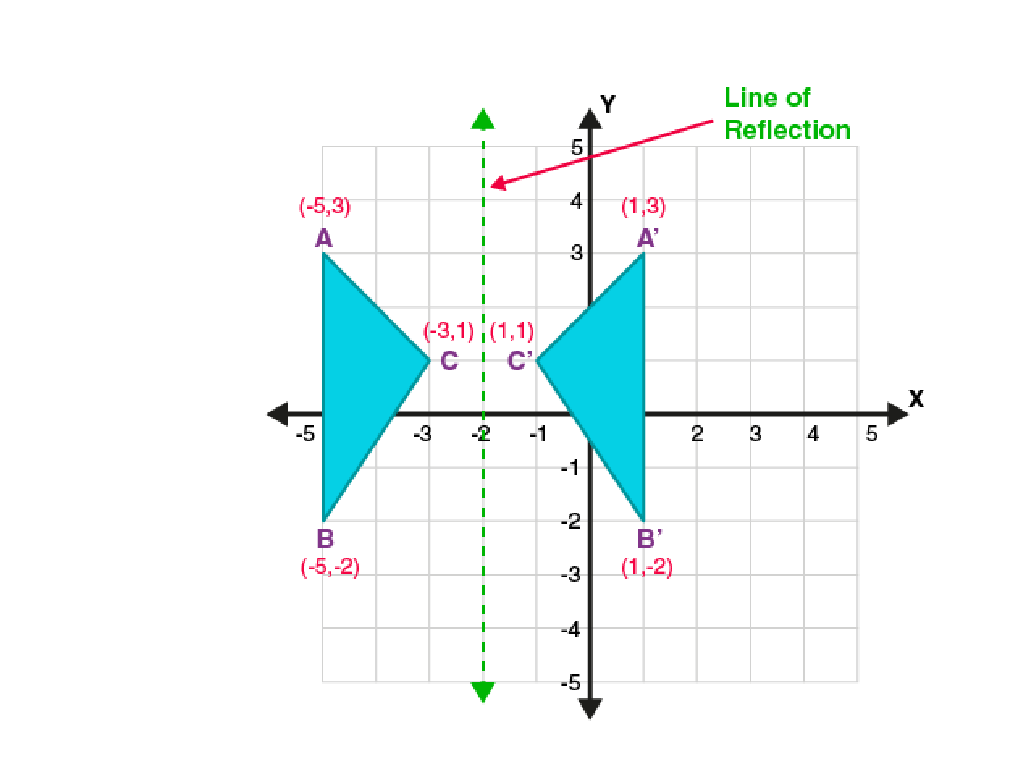
Identifying Points on a Graph – Reading graph points (x, y) – Each point on a graph has an x (horizontal) and y (vertical) coordinate. – Practice with graph points – Let’s plot points like (3, 5) or (2, 4) on a graph together. – Points represent two variables – X and Y correspond to variables in an equation, like x for time and y for speed. – Relating points to equations – We can write equations like y = mx + b using points from the graph. | This slide introduces students to the concept of reading and identifying points on a graph, which is a fundamental skill in understanding two-variable equations. Start by explaining the coordinate system, where the horizontal value represents the x-coordinate and the vertical value represents the y-coordinate. Engage the class with hands-on practice by plotting given points on a graph. Clarify how these points reflect the relationship between two variables in real-world situations. Finally, demonstrate how to derive a linear equation using the points from the graph, setting the stage for understanding how tables of values can help in writing equations.

Creating a Table from a Graph – Steps to create a table – Identify points on the graph, list them as ordered pairs (x, y). – Fill table with graph values – Use the graph’s points to fill in the x and y columns. – Recognize patterns with tables – Tables show how y changes with x, revealing trends or patterns. – Table to equation example – Example: From the table, derive the equation representing the relationship. | This slide is aimed at teaching students how to translate information from a graph into a table format, which is a critical step in understanding the relationship between variables in a two-variable equation. Start by explaining how to identify points on a graph and how these points correspond to x and y values. Emphasize the importance of accuracy when transferring these values into a table. Once the table is filled, guide students to look for patterns in the data, such as consistent increases or decreases, which can help in determining the type of relationship between the variables. Conclude by showing an example of how a table can help us write an equation that models the graphed data. Encourage students to practice with different graphs to become comfortable with the process.

Equations from Graphs: The Role of Tables – Discover patterns in data tables – Learn about slope and y-intercept – Slope is the rate of change; y-intercept is where the line crosses the Y-axis – Find the slope from a table – Slope (m) is rise over run between any two points – Determine the y-intercept from a table – Y-intercept (b) is the value when x is 0 in the table | This slide introduces students to the process of writing equations from graphs by using tables. Start by guiding students to look for patterns in the table that indicate a consistent relationship between the x (independent variable) and y (dependent variable) values. Introduce the concept of slope as a measure of how steep a line is and the y-intercept as the point where the line crosses the y-axis. Teach students how to calculate the slope by dividing the difference in y-values by the difference in x-values (rise over run) and how to find the y-intercept by identifying the y-value when x is zero. Provide examples and practice problems to help students understand and apply these concepts.

Writing Equations from Graphs – Use slope and y-intercept – Find the rate of change and starting point – Linear equation format: y = mx + b – m is the slope, b is the y-intercept – Writing equations from tables – Convert table data into an equation – Practice with real examples – Apply the formula to graphed data | This slide introduces students to the process of writing a linear equation from a graph by using a table. Start by explaining the concept of slope as the rate of change and y-intercept as the starting point. Emphasize the linear equation format y = mx + b, where ‘m’ represents the slope and ‘b’ the y-intercept. Provide examples of how to extract these values from a table and use them to write an equation. Encourage students to practice with real examples by converting the data from a table into a linear equation and then plotting it on a graph. This will help them understand the relationship between the equation, the table, and the graph.

Practice Time: Writing Equations from Graphs – Let’s write an equation together – Work through a class example – We’ll use a graph and table to form an equation – Encourage questions and discussion – No question is too small, every thought can lead to understanding – Think aloud as we solve – Share your thought process, it helps everyone learn | This slide is designed for an interactive class activity where students will engage in writing an equation from a graph using a table. Start by presenting a graph with plotted points and a corresponding table of values. Walk through the process of determining the slope and y-intercept from the graph and how to translate this into an equation. Encourage students to participate by asking questions and thinking aloud as they work through the problem. This will help to create an environment where students feel comfortable sharing their thought process and learn from each other. Provide guidance and support as needed, and be prepared with additional practice problems for students who may grasp the concept quickly and need more challenges.

Class Activity: Graphs & Equations – Pair up and create a graph – Fill in a table from your graph – Use the graph to determine values for the table – Write an equation for your graph – Use the table to derive the equation – Present your findings to the class – Explain how you created the graph and equation | This activity is designed to reinforce students’ understanding of the relationship between graphs, tables, and equations. Students will work in pairs to foster collaboration. They should start by plotting points on a graph, then fill in a corresponding table with the x (independent variable) and y (dependent variable) values. Next, they will use the table to write a two-variable equation that represents the relationship shown in their graph. Encourage creativity in their graph creation but ensure that the resulting graph is a straight line, indicating a linear relationship. Possible activities: 1) Graphing a simple linear function, 2) Creating a story context for their graph, 3) Exchanging graphs with another pair to write the equation, 4) Discussing how changes in the graph affect the equation. This will help them understand the concept of slope and y-intercept in a two-variable equation.

Conclusion: Graphs, Tables, and Equations – Recap of graphing two-variable equations – Homework: Real-world two-variable example – Find an example like temperature over a week – Create a graph and table for your example – Plot points on a graph and organize in a table – Write the equation from your graph – Use the table to write the equation of the line | As we conclude today’s lesson, remind students of the importance of understanding the relationship between graphs, tables, and equations. For homework, they should find a real-world example of a two-variable relationship, such as the temperature over a week or the height of a plant over time. They need to create a graph, organize the data in a table, and then write the corresponding equation. This exercise will help solidify their understanding of how to write an equation from a graph using a table. In the next lesson, we will focus on solving these two-variable equations, building on what they’ve learned today. Encourage creativity in their example selection and ensure they understand the steps for the homework.